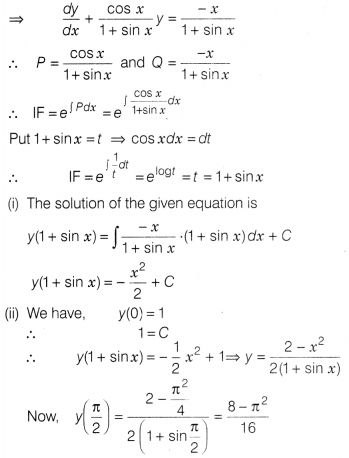CBSE Sample Papers for Class 12 Maths Set-4

CBSE Sample Papers for Class 12 Maths Set 4 with Solutions

Time Allowed: 3 Hours
Maximum Marks: 80

General Instructions:

1. This question paper contains – five sections A, B, C, D and E. Each section is compulsory. However, there are internal choices in some questions.
2. Section A has 18 MCQ’s and 02 Assertion-Reason based questions of 1 mark each.
3. Section B has 5 Very Short Answer (VSA) type questions of 2 marks each.
4. Section C has 6 Short Answer (SA) type questions of 3 marks each.
5. Section D has 4 Long Answer (LA) type questions of 5 marks each.
6. Section E has 3 source based/case/passage based/intergrated units of assessment (4 marks each) with sub-parts.

Section A
(Multiple Choice Questions) Each question carries 1 mark

Question 1.
The area of the region bounded by the curve y = \(\frac{1}{x}\), the X-axis and between x = 1 to x = 6 is
(a) log_e 3 sq units
(b) log_e 6 sq units
(c) log5 sq units
(d) 6 sq units
Solution:
(b) Required area = \(\int_1^6\)y dx = \(\int_1^6\frac{1}{x}\) dx
= [log x]⁶₁
= log_e 6 sq units.

Question 2.
The rate of change of the area of a circle with respect to its radius r, when r = 3 cm, is
(a) \(\frac{6\pi}{5}\)cm²/cm
(b) 3π cm²/cm
(c) 6π cm²/cm
(d) \(\frac{3\pi}{5}\)cm²/cm
Solution:
(c) Area of circle = πr²
i.e. A = πr²
\(\frac{dA}{dr}\) = 2πr
(\(\frac{dA}{dr}\))_r=3 = 2π(3)
= 6π cm2/cm

Question 3.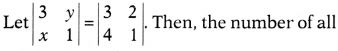

possible pair of values of x and y, if x and y are natural numbers.
(a) 1
(b) 2
(c) 3
(d) 4
Solution:

⇒ 3 – xy = 3 – 8 ⇒ xy = 8
⇒ (x, y) = (1, 8), (2, 4), (4, 2), (8, 1)

Question 4.
The value of ∫x² e^x³dx is
(a) \(\frac{1}{3}\) e^x³ + C
(b) \(\frac{1}{3}\) e^x4 + C
(c) \(\frac{1}{2}\) e^x³ + C
(d) \(\frac{1}{2}\) e^x² + C
Solution:
(a) Let ∫x² e^x³ dx
Put x³ = t ⇒ 3x²dx = dt
∴ l = \(\frac{1}{3}\)∫e^t dt = \(\frac{1}{3}\)e^t + C ⇒ l = \(\frac{1}{3}\)e^x³ + C

Question 5.
If y = 3e^2x + 2e^3x then the value of y₂ – 5y₁ + 6y is
(a) 1
(b) 0
(c) 2
(d) 3
Solution:
(b) We have, y = 3e^2x + 2e^3x
y₁ = 6e^2x + 6e^3x
y₂ = 12e^2x + 18e^3x
y₂ = 6(2e^2x + 3e^3x)
Hence,
y₂ – 5y₁ + 6y = 6(2e^2x + 3e^3x) – 30(e^2x + e^3x) + 6(3e^2x + 2e^3x)
= 0

Question 6.
If the function f defined as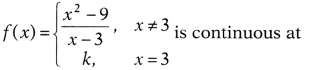

x = 3, then the value of k is
(a) 1
(b) 2
(c) 6
(d) 5
Solution: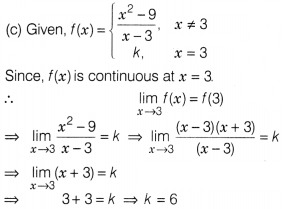

Question 7.
For what values of a, the vectors \(\hat{i}-3\hat{j}+4\hat{k}\) and \(a\hat{i}+6\hat{j}-8\hat{k}\) are collinear?
(a) 4
(b) -4
(c) -2
(d) 2
Solution:

Question 8.
If P(A) = \(\frac{1}{2}\), P(B) =0, then P(\(\frac{A}{B}\)) is
(a) zero
(b) 2
(c) not defined
(d) 11
Solution:
(c) It is given that P(A) = \(\frac{1}{2}\) and P(B) = 0

Therefore, P (\(\frac{A}{B}\)) is not defined.

Question 9.
If f'(1) = 2 and y = f (log_e x), then find \(\frac{dy}{dx}\) at x = e.
(a) \(\frac{2}{e}\)
(b) \(\frac{3}{e}\)
(c) \(\frac{1}{e}\)
(d) \(\frac{2}{5e}\)
Solution:
(a) We have, y = f(log_e x)
∴ \(\frac{dy}{dx}\) = f'(log_e x).\(\frac{1}{x}\)
Now, on putting x =e, we get
\(\frac{dy}{dx}\)|_x=e = f'(log_e e).\(\frac{1}{e}\) = \(\frac{1}{e}\)f’(1) [∵ log_ee = 1]
= \(\frac{2}{e}\) [∵ f'(1) = 2]

Question 10.
The particular solution of the differential equation \(\frac{dy}{dx}\) = y tan x at y = 1, x = 0 is
(a) y = cos x
(b) y = sec x
(c) y sin x = 6
(d) y = tan x
Solution:
(b) We have, \(\frac{dy}{dx}\) = y tan x ⇒ \(\frac{dy}{y}\) = tan x dx
⇒ ∫\(\frac{dy}{y}\) = ∫tan x dx
⇒ log y = log |secx| + C
On putting y = 1 and x = 0 in (i), we get
C = 0
∴ y = sec x

Question 11.
\(\int_1^4\)|x – 5| dx is equal to
(a) \(\frac{15}{2}\)
(b) \(\frac{13}{2}\)
(c) 1
(d) 4
Solution: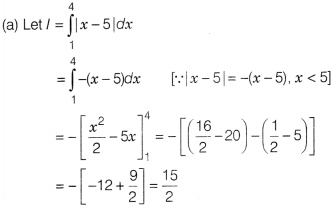

Question 12.
The value of p, for which the vectors \(3\hat{i}+2\hat{j}+9\hat{k}\) and \(\hat{i}-2p\hat{j}+3\hat{k}\) are parallel, is
(a) –\(\frac{1}{3}\)
(b) \(\frac{1}{3}\)
(c) 2
(d) \(\frac{1}{\sqrt3}\)
Solution:
(a) Given, \(3\hat{i}+2\hat{j}+9\hat{k}\) and \(\hat{i}-2p\hat{j}+3\hat{k}\) are two parallel vectors, so their direction ratios will be proportional.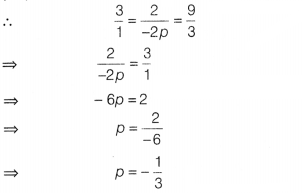

Question 13.
The degree of the differential equation \((\frac{dy}{dx})^5=2x^2(\frac{d^2y}{dx^2})^4\) = 0 is
(a) 1
(b) 2
(c) 3
(d) 4
Solution:
(d) Degree = 4

Question 14.
The minimum value of the function f(x) = |x – 4| exists at
(a) x = 0
(b) x = 2
(c) x = 4
(d) x = -4
Solution:
(c) Given function, f(x) = | x – 4|
Graph of f(x),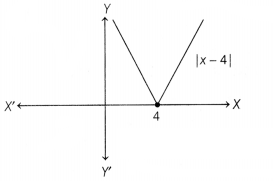

From graph, we observe that f(x) has minimum value at x = 4.

Question 15.
The function f: N → N, N being the set of natural numbers, defined by f(x) = 2x + 3 is
(a) injective and surjective
(b) injective but not surjective
(c) not injective but surjective
(d) neither injective nor surjective
Solution:
(b) Given, f: N → N defined by f(x) = 2x + 3
Let f(x₁) = f(x₂)
⇒ 2x₁ + 3 = 2x₂ + 3
⇒ x₁ = x₂
Hence, f(x) is injective.
Let f(x) = y
⇒ y = 2x + 3
⇒ x = \(\frac{y-3}{3}\)
Let  y = 4
i.e. y ∈ N but x ∉ N.
Hence, f(x) is not surjective.

Question 16.
The corner points of the feasible region determined by the following system of linear inequalities 2x + y ≤ 10, x + 3y ≤ 15, x, y ≥ 0 are (0, 0) (5, 0) (3, 4) and (0, 5). Let Z = px + qy, where p, q > 0. Condition on p and q, so that the maximum of Z occurs at both (3, 4) and (0, 5), is
(a) p = q
(b) p = 2q
(c) p = 3q
(d) q = 3p
Solution:
(d) The maximum value of Z is unique.
It is given that the maximum value of Z occurs at two points (3, 4) and (0, 5).
Value of Z at (3, 4) = Value of Z at (0, 5)
⇒ p(3) + g(4) = p(0) + q(5)
⇒ 3p + 4q = 5q
⇒ 3p = q

Question 17.
Suppose there is a relation R between the positive numbers x and y given by x Ry if and only if x ≤ y². Then, which one of the following is correct?
(a) R is reflexive but not symmetric
(b) R is symmetric but not reflexive
(c) R is neither reflexive nor symmetric
(d) None of the above
Solution:
(a) Reflexive Given, xRy ⇒ x is less than y².
∴ xRx ⇒ x is less than x², which is true.
Hence, R is reflexive.
Symmetric xRy is not equivalent to yRx because
1R2 ⇒ 1 is less than 2².
2R1 ⇒ 2 is less than 1².
Thus, it is not symmetric.

Question 18.
sin^-1(cos \(\frac{3\pi}{5}\)) is equl to
(a) \(\frac{\pi}{10}\)
(b) \(\frac{3\pi}{5}\)
(c) \(\frac{-\pi}{10}\)
(d) \(\frac{-3\pi}{5}\)
Solution: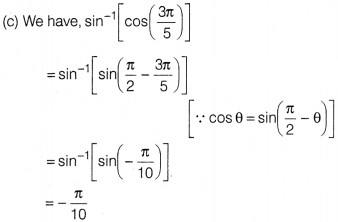

Assertion-Reason Based Questions
In the following questions, a statement of Assertion (A) is followed by a statement of Reason (R). Choose the correct answer out of the following choices.
(a) Both A and R are true and R is the correct explanation of A
(b) Both A and R are true but R is not the correct explanation of A
(c) A is true but R is false
(d) A is false but R is true

Question 19.
Assertion (A) f(x) is continuous at x = a, if

Reason (R) If fix) is continuous at a point, then \(\frac{1}{f(x)}\) is also continuous at the point.
Solution:
(c) Assertion We know that

x = a, while both limits must exist.
Reason If f(x) is continuous at a point, then it is not necessary that \(\frac{1}{f(x)}\) is also continuous at that point.
e.g. f(x) = x is continuous at x = 0 but \(\frac{1}{f(x)}=\frac{1}{x}\) is not continuous at x = 0

Question 20.
Assertion (A) The matrix

matrix of order 3.
Reason (R) If A = [a_ij]_m×l, then A is column matrix.
Reason In general, A = [a,y]m x -i is a column matrix.
Solution: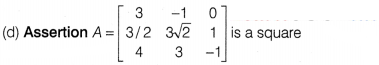

matrix of order 3.
Reason in general, A = [a_ij]_m×1, is a column matrix.

Section B
(This section comprises of very short answer type questions (VSA) of 2 marks each)

Question 21.
Evaluate \(\int_0^1\frac{2x}{5x^2+1}\)dx.
Or
Evaluate \(\int_0^2\)[x²]dx, where [•] is the greatest integer function.
Solution: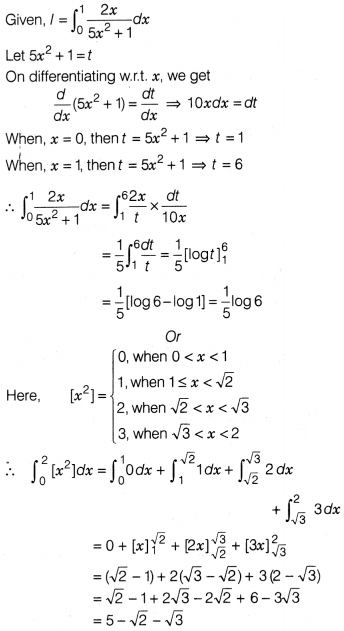

Question 22.
Evaluate ∫(x + 1) e^x log (xe^x)dx.
Solution:
Let l = ∫(x + 1) e^x log (xe^x)dx
On putting, xe^x = t ⇒ (e^x + xe^x)dx = df
⇒ (1 + x)e^xdx = dt

[using integration by parts]
= t.logt – ∫1dt = t.logt – t + C
= xe^x log(xe^x) – xe^x +C [∵ t = xe^x]
= xe^x[log(xe^x) – 1] + C

Question 23.
A committee of 4 students is selected at random from a group consisting of 8 boys and 4 girls. If there is atleast one girl in the committee, then calculate the probability that there are exactly 2 girls in the committee.
Or
If P(A) = \(\frac{1}{4}\), P(B) = \(\frac{1}{5}\) and P(A ∩ B) =\(\frac{1}{7}\), then find P(\(\overline{\mathrm{A}}/\overline{\mathrm{B}}\)).
Solution:
Let A denotes the event that atleast one girl will be chosen and B denotes the event that exactly 2 girls will be chosen. Then, to find P(\(\overline{\mathrm{B}}/\overline{\mathrm{A}}\))
Now, P(A) = 1 – P(\(\overline{\mathrm{A}}\)) = 1 – P (no girl is chosen)

Question 24.
A balloon which always remains spherical has a variable diameter \(\frac{3}{2}\)(2x + 1). Then, find the rate of change of its volume with respect to x.
Solution:
Given, diameter of the balloon = \(\frac{3}{2}\)(2x + 1)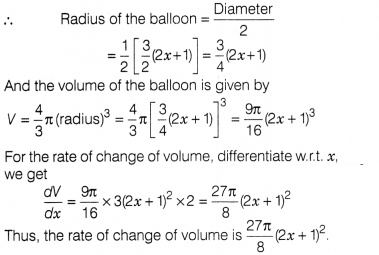

Question 25.
Given, \(\vec{a}+\vec{b}+\vec{c}\) = 0 |\(\vec{a}\)| = 5, |\(\vec{b}\)| = 6 and |\(\vec{c}\)| = 9, then find angle between \(\vec{a}\) and \(\vec{b}\).
Solution: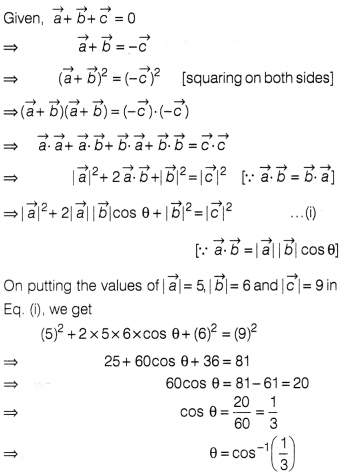

Section C
This section comprises of short answer type questions (SA) of 3 marks each

Question 26.
Three persons A, B and C apply for the job of Manager in a private company. Chances of their selection (A, B and C) are in the ratio 1 : 2 : 4. The probabilities that A, B and C can introduce changes to improve profits of the company are 0.8, 0.5 and 0.3, respectively. If the change does not take place, find the probability that it is due to the appointment of C.
Solution:
Let us define the following events
A = Selecting person A
B = Selecting person B
C = Selecting person C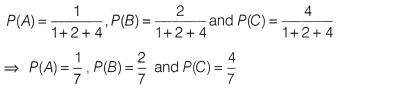

Let E = Event of introducing the changes in their profit.
Also, given P(\(\frac{E}{A}\)) = 0.8, P(\(\frac{E}{B}\)) = 0.5 and P(\(\frac{1}{C}\)) = 0.3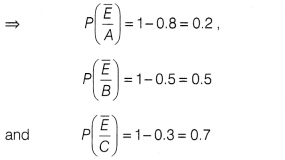

The probability that change does not take place due to the appointment of C,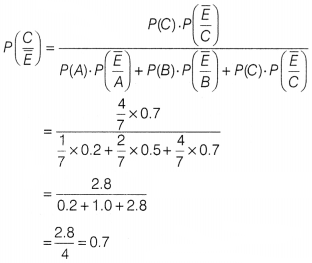

Question 27.
Evaluate ∫\(\frac{dx}{1-3sin x}\)
Or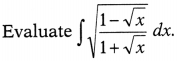

Solution: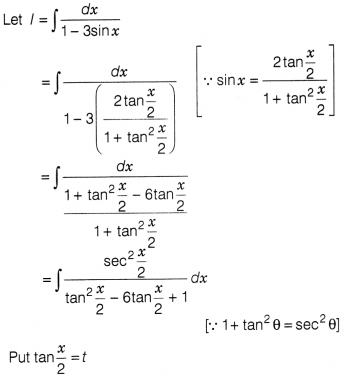

On differentiating both sides w.r.t. x, we get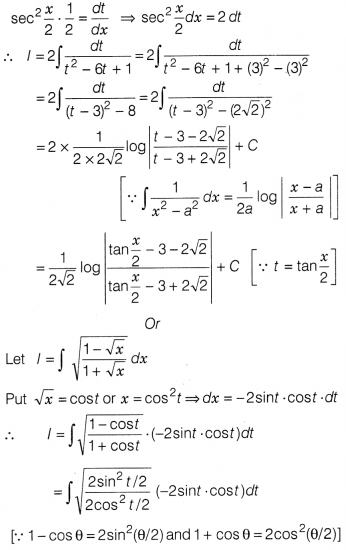

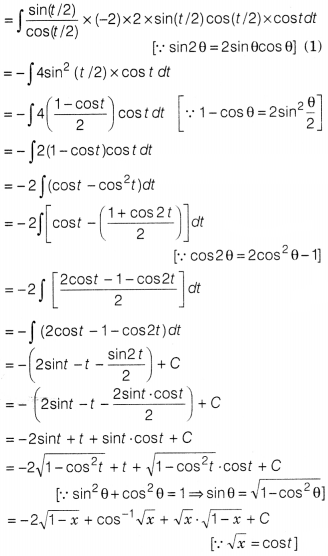
Question 28.
Solve the following differential equation
x(\(\frac{dy}{dx}\)) = y – x tan(\(\frac{y}{x}\))
Or
Solve the following differential equation
x cos(\(\frac{y}{x}\))\(\frac{dy}{dx}\) = y cos(\(\frac{y}{x}\)) + x; x ≠ 0
Solution:
Given, differential equation is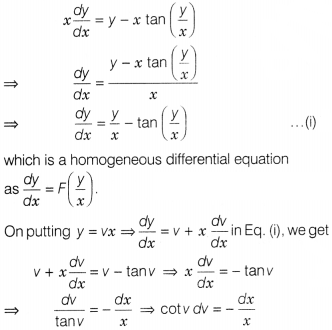

On integrating both side, we get

witch is the required solution of given differential equation.

Question 29.
For any two vectors \(\vec{a}\) and \(\vec{b}\), show that
(l + |\(\vec{a}\)|²)(1 + |\(\vec{b}\)|²) = {(1 – \(\vec{a}.\vec{b}\))}² + |\(\vec{a}+\vec{b}\) + (\(\vec{a}\times\vec{b}\))|²
Or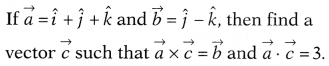

Solution: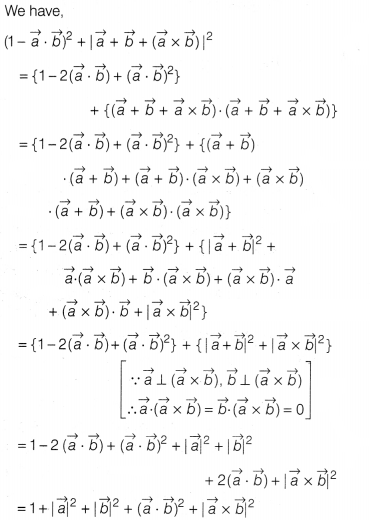

Question 30.
Bag A contains 4 black and 6 red balls and bag B contains 7 black and 3 red balls. A die is thrown if 1 or 2 appears on it, then bag A is chosen, otherwise bag B. If two balls are drawn at random (without replacement) from the selected bag, then find the probability of one of them being red and another black.
Solution:
Given, bag A = 4 black and 6 red balls
and bag B = 7 black and 3 red bails.
Let E₁ = The event that die shows 1 or 2
E₂ = The event that die show 3 or 4 or 5 or 6
E = The event that among two drawn balls, one of them is red and other is black

Question 31.
Find the shortest distance between the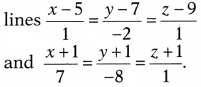

Solution:
Given equations of lines are

On comparing above equations with one point form
of equation of line, which is \(\frac{x-x_1}{a}=\frac{y-y_1}{b}=\frac{z-z_1}{c}\),
we get
a₁ = 1, b₁ = -2, c₁ = 1, x₁ = 5, y₁ = 7, z₁ = 9 and a₂ = 7, b₂ = 8, c₂ = 1, x₂ = -1, y₂ = – 1, z₂ = -1
We know that the shortest distance between two lines is given by

Hence, the required shortest distance is 8√3 units.

Section D
This section comprises of long answer type questions (LA) of 5 marks each

Question 32.
Show that the function f : R → R defined by f(x) = \(\frac{x}{x^{2}+1}\) ∀ x ∈ R is neither one-one nor onto.
Solution:
Given, f : R → R, defined by
f(x) = \(\frac{x}{x^{2}+1}\) ∀ x ∈ R
Let x₁ x₂ ∈ R such that f(x₁) = f(x₂)

or (1 – 2k) (1 + 2k) < 0 i.e. k > 1/2 or k < -1/2
So, f is not onto.
Hence, f is neither one-one nor onto.

Question 33.
Solve the following system of equations by matrix method when x ≠ 0, y ≠ 0 and z ≠ 0.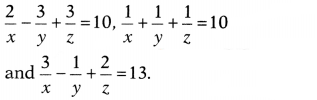

Or
The sum of three numbers is 6. Twice the third number when added to the first number gives 7. On adding the sum of the second and third numbers to thrice the first number, we get 12. Find the numbers, using matrix method.
Solution:
Given, system of equations is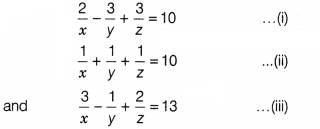

Given equations can be written in matrix form as AX = B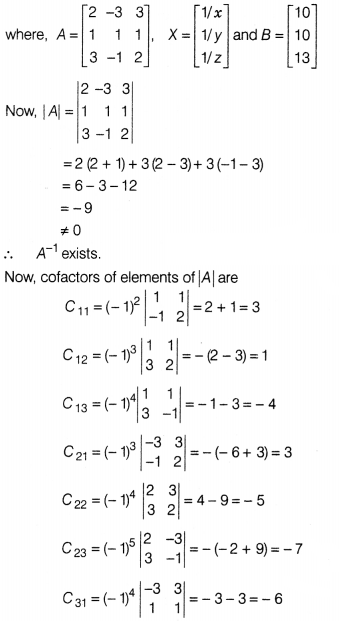

On comparing the corresponding elements, we get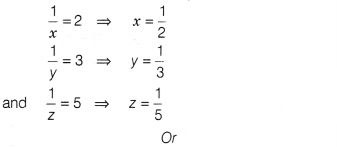

Let the first, second and third numbers be x, y and z, respectively. Then,
x + y + z = 6 …(i)
x + 2z = 7 …(ii)
3x + y+z = 12 …(iii)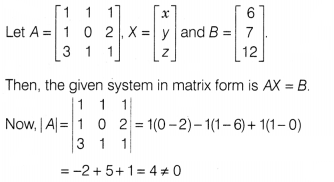

∴ A is invertible.
So, the given system has a unique solution, X = A^-1B.
The minors of the elements of |A| are
M₁₁ = -2, M₁₂ = -5, M₁₃ = 1,
M₂₁ = 0, M₂₂ = -2, M₂₃ = -2,
M₃₁ = 2, M₃₂ = 1, M₃₃ = -1
The cofactors of the elements of j /4| are
A₁₁ = -2, A₁₂ = 5, A₁₃ = 1,
A₂₁ = 0, A₂₂ = -2, A₂₃ = 2,
A₃₁ = 2, A₃₂ = -1, A₃₃ = -1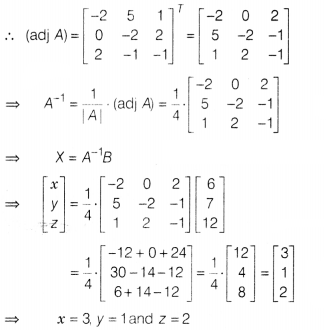

Hence, the required numbers are 3, 1 and 2.

Question 34.
Find the value of p, so that the lines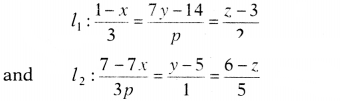

are perpendicular to each other. Also, find the equation of a line passing through a point (3, 2, -4) and parallel to line l₁.
Or
Find the shortest distance between the lines

Also, find the equations of the shortest distance.
Solution:
Given equation of lines can be written in standard form as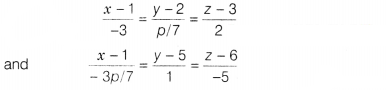

Direction ratios of these lines are -3, \(\frac{p}{7}\), 2 and –\(\frac{3p}{7}\), 1, -5, respectively.
We know that two lines with direction ratios a₁, b₁, c₁ and a₂, b₂, c₂ are perpendicular to each other, if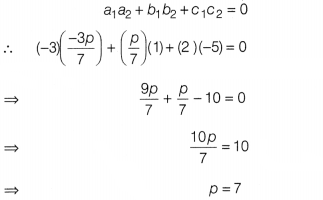

Thus, the value of p is 7.
Also, we know that the equation of a line which passes through the point (x₁, y₁, z₁) with direction ratios a, b and c is given by

Any point P on line (i) is
P(3λ + 8, -16λ – 9, 7λ + 10) …(iii)
and any point G on line (ii) is
Q(3µ + 15, 8µ + 29, -5µ + 5) … (iv)
So, the direction ratios of PQ are
(3µ + 15 – 3λ – 8, 8µ + 29 + 16λ + 9, – 5µ + 5 – 7λ – 10)
i.e. (3µ – 3λ + 7, 8µ + 16λ + 38, -5µ – 7λ – 5)
Now, |PQ| will be the shortest distance between lines (i) and (ii), if PQ is perpendicular to both lines (i) and (ii)
3(3µ – 3λ + 7) – 16(8µ + 16λ + 38) + 7(-5µ – 7λ – 5) = 0 [∵ a₁a₂ + b₁b₂ + c₁c₂ = 0]
⇒ 9µ – 9λ + 21 -128µ – 256λ – 608 – 35µ – 49λ – 35 = 0
⇒ -154µ – 314λ – 622 = 0
⇒ 77µ + 157λ + 311 = 0 [dividing by (-2)] …(v)
and 3(3µ – 3λ +7) + 8(8µ + 16λ + 38) -5(-5µ – 7λ – 5) = 0 [∵ a₁a₂ + b₁b₂ + c₁c₂ = 0]
⇒ 9µ – 9λ + 21 + 64µ + 128λ + 304 + 25µ + 35λ + 25 = 0
⇒ 98µ + 154λ + 350 = 0
⇒ 7µ + 11λ + 25 = 0 [dividing by 14] …(vi)

On multiplying Eq. (vi) by 11 and then subtracting it from Eq. (v), we get
(77µ + 157λ + 311) – (77µ + 121λ + 275) = 0
⇒ 36λ + 36 = 0 ⇒ λ = -1

On putting the value of λ in Eq. (v), we get
77µ + 157(-1) + 311 = 0
⇒ 77µ – 157 + 311 = 0
⇒ 77µ + 154 = 0
⇒ µ = -2

On putting the values of 3 and (i in Eqs. (iii) and (iv), we get
Coordinates of P = (-3 + 8, 16 – 9, -7 + 10) = (5,7, 3)
and coordinates of Q = (-6 + 15, -16 + 29, 10 + 5)
= (9, 13, 15)
∴ Shortest distance between two lines,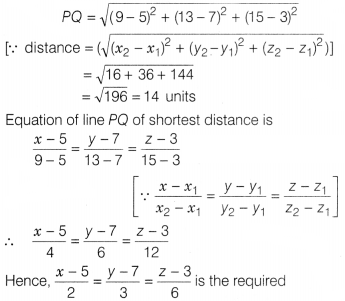

equation of the line which gives shortest distance.

Question 35.
Find the minimum value of (ax + by), where xy = c².
Solution:
Let f(x) = ax + by, whose minimum value is required.

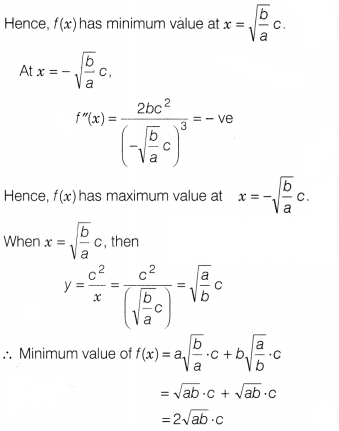
Section E
This section comprises of 3 case-study/passage-based questions of 4 marks each

Question 36.
If A = [a_ij] be a m × n matrix, then the matrix obtained by interchanging the rows and columns of A is called the transpose of A.
A square matrix A = [a_ij] is said to be symmetric, if A^T = A for all possible values of i and l.
A squere matrix A = [a_ij] is said to be skew-symmetric, if A^T = -A for all possible values of i and j.
Based on the above information, answer the following questions.
(i) Find the transpose of [1 – 2 – 5].
(ii) Find the transpose of matrix (ABC).
(iii) Evaluate (A + B)^T – A, where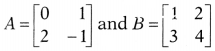

Or

Solution:

Question 37.
The feasible solution for a LPP is shown below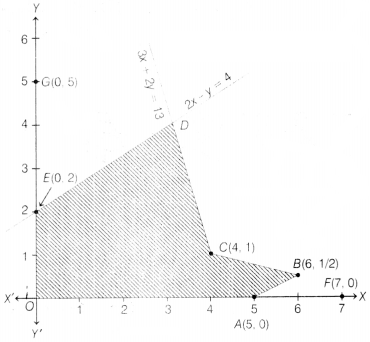

and the objective function is Z = 15x – 4y.
Based on the above information, answer the following questions.
(i) Find the value of (n – 1)², where n is number of corner points.
(ii) Find Z _{(6, \(\frac{1}{2}\))} + Z_{(0, 2)}
(iii) Find the coordinate of point D.
Or
Find the maximum of Z.
Solution:
(i) Since, shaded region is OABCDEO.
The number of corner points, n = 6
∴ (n – 1)² =(6 – 1)² = 5² = 25

(ii) Z _{(6, \(\frac{1}{2}\))} + Z_{(0, 2)} = 88 + (-8) = 80

(iii) Given, equation of lines 3x + 2y = 13 …(i)
and 2x – y = 4 …(ii)
The point D is the intersection point of the above two lines.
On multiplying by 2 in Eq. (ii), we get
4y – 2y = 8 …(iii)
On adding Eqs. (i) and (iii), we get
7x = 21 ⇒ x = 3
From Eq. (ii), 2x – 4 = y
⇒ y = 2 × 3 – 4 = 2
Hence, the coordinates of point D are (3, 2).
Or
Value of objective function at all corner points.

Corner points	Value of Z = 15x – 4y
O(0, 0)	15(0) – 4(0) = 0
A(5, 0)	15 × 5 – 4 × 0 = 75
B(6, \(\frac{1}{2}\))	15 × 6 – 4 × \(\frac{1}{2}\)) = 88 (Maximum)
C(4, 1)	15 × 4 – 4 × 1 = 56
D(3, 2)	15 × 3 – 4 × 2 = 37
E(0, 2)	15 × 0 – 4 × 2 = -8 (Minimum)

∴ Maximum of Z is 88.

Question 38.
Consider the given equation \(\frac{dy}{dx}\) + Py = Q.
The above equation is known as linear differential equation. Here, IF = e^∫Pdx and solution is given by y . IF = ∫Q . IF dx + C
Now, consider the given equation
(1 + sin x)\(\frac{dy}{dx}\) + ycos x + x = 0.
On the basis of above information, answer the following questions.
(i) What is the solution of the given equation?
(ii) If y (0) = 1, then what is the value of y(\(\frac{\pi}{2}\))?
Solution:
We have, (1 + sin x)\(\frac{dy}{dx}\) + y cos x + x = 0