Nuclei

Class 12 Physics Chapter 13 Important Extra Questions Nuclei

Short Answer Type

Question 1.
An electron and alpha particle have the same de-Broglie wavelength associated with them. How are their kinetic energies related to each other?
(Delhi 2008)
Answer:

Question 2.
State the reason, why heavy water is generally used as a moderator in a nuclear reactor. (Delhi 2008)
Answer:
Neutrons produced during fission get slowed if they collide with a nucleus of the same mass. As ordinary water contains hydrogen atoms (of mass nearly that of neutrons), so it can be used as a moderator. But it absorbs neutrons at a fast rate via reaction :

Here d is deutron. To overcome this difficulty, heavy water is used as a moderator which has negligible cross-section for neutron absorption.

Question 3.
Name the absorbing material used to control the reaction rate of neutrons in a nuclear reactor. (Delhi 2008)
Answer:
Control rod or cadmium rod.

Question 4.
State tzvo characteristic properties of nuclear force. (All India 2008)
Answer:
(i) Nuclear forces are the strongest force in nature.
(ii) They are saturated forces.
(iii) They are charge independent.

Question 5.
Two nuclei have mass numbers in the ratio 1: 2. What is the ratio of their nuclear densities? (Delhi 2009)
Answer: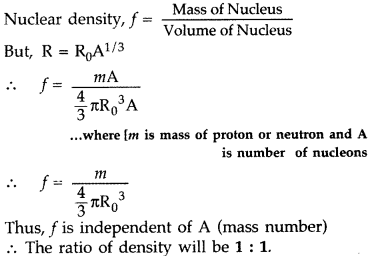

Question 6.
Two nuclei have mass number in the ratio 1 : 3. What is the ratio of their nuclear densities? (Delhi 2009)
Answer:
Since nuclear density is independent of the mass number, the ratio of nuclear densities will be 1:1.

Question 7.
Two nuclei have mass numbers in the ratio 2 : 5. What is the ratio of their nuclear densities? (Delhi 2009)
Answer:
Nuclear density is independent of mass number, so the ratio will be 1 : 1.

Question 8.
Two nuclei have mass numbers in the ratio 1: 8. What is the ratio of their nuclear radii? (All India 2009)
Answer:

Question 9.
Two nuclei have mass numbers in the ratio 8:125. What is the ratio of their nuclear radii? (All India 2009)
Answer: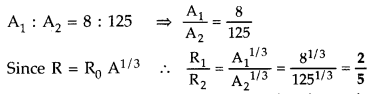

Question 10.
Two nuclei have mass numbers in the ratio 27:125. What is the ratio of their nuclear radii? (All India 2009)
Answer: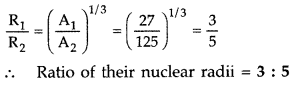

Question 11.
Write any two characteristic properties of nuclear force. (All India 2009)
Answer:
1. Nuclear forces are strongest forces in nature.
2. Nuclear forces are charge independent.

Question 12.
What is the relationship between decay constant and mean life of a radioactive nucleus?

Question 13.
What will be the ratio of the radii of two nuclei of mass numbers A₁ and A₂?
Answer:
The ratio is \(\frac{R_{1}}{R_{2}}=\left(\frac{A_{1}}{A_{2}}\right)^{1 / 3}\)

Question 14.
Two nuclei have mass numbers in the ratio 1: 2. What is the ratio of their nuclear densities?
Answer:
The densities of both nuclei are equal as they do not depend upon mass number.

Question 14 a.
A nucleus of mass number A has a mass defect Δm. Give the formula, for the binding energy per nucleon of this nucleus.
Answer:
The formula is E = \(\frac{\Delta m \times c^{2}}{A}\)

Question 15.
Write the relation between half-life and decay constant of a radioactive sample.
The relation is T_1/2 = \(\frac{0.693}{\lambda}\)

Question 16.
Write the nuclear decay process for β-decay of 15³²P.
Answer:
The process is

Question 17.
State the relation between the mean life (τ) of a radioactive element and its decay constant λ.
Answer:
The two are related as τ = 1 / λ.

Question 18.
Write any two characteristic properties of nuclear force. (CBSE AI 2011)
Answer:

1. Do not obey inverse square law and
2. Spin-dependent.

Question 19.
How is the radius of a nucleus related to its mass number? (CBSE AI 2011C, AI 2013C)
Answer:
The radius R of the nucleus and mass number A is related as R = R_oA^1/3, where R_o is a constant.

Question 20.
A nucleus undergoes β – decay. How does
(i) the mass number,
(ii) atomic number change? (CBSE Delhi 2011C)
Answer:
During β – decay
(i) the mass number remains the same,
(ii) atomic number increases by one.

Question 21.
Define the activity of a given radioactive substance. Write its SI unit. (CBSE AI 2013)
Answer:
The rate of disintegration in a radioactive substance is known as its activity. SI unit is becquerel (Bq).

Question 22.
Why is it found experimentally difficult to detect neutrinos in nuclear β-decay? (CBSE AI 2014)
Answer:
They are very difficult to detect since they can penetrate a large quantity of matter (even earth) without any interaction.

Question 23.
Four nuclei of an element undergo fusion to form a heavier nucleus, with the release of energy. Which of the two — the parent or the daughter nucleus – would have higher binding energy per nucleon? (CBSE AI 2018, Delhi 2018)
Answer:
Daughter nucleus.

Question 13.
Why is nuclear fusion not possible In the laboratory?
Answer:
Because temperature as high as 10⁷ K cannot be sustained in the laboratory.

Question 24.
Why is the penetrating power of gamma rays very large?
Answer:
Because they have high energy and are neutral.

Question 25.
Can it be concluded from beta decay that electrons exist inside the nucleus?
Answer:
No, the beta particle although an electron is actually created at the instant of beta decay and ejected at once. It cannot exist inside the nucleus as its de-Broglie wavelength is much larger than the dimensions of the nucleus.

Question 26.
Why is the ionizing power of α – parties greater than that of γ-rays?
Answer:
Because α – particles are heavy particles and their speed is comparatively small, so they collide more frequently with atoms of the medium and ionize them.

Question 27.
When a nucleus undergoes alpha decay, is the product atom electrically neutral in beta decay?
Answer:
No, in alpha decay, the atomic number decreases by 2 hence the atom is left with 2 extra orbital electrons. It, therefore, has a double negative charge. In beta decay, the atom is left with a net positive charge.

Question 28.
You are given two nuclides ₃⁷X and ₃⁴Y. Are they the isotopes of the same element? Why?
Answer:
Yes, because an atomic number of both nuclides is 3.

Question 29.
The variation of the decay rate of two radioactive samples A and B with time is shown in the figure. Which of the two has a greater decay constant? (NCERT Exemplar)

Answer:
The decay constant of A is greater than that of B but it does not always decay faster than B.

Question 30.
Does the ratio of neutrons to protons in a nucleus increase, decrease, or remain the same after the emission of an alpha particle? (NCERT Exemplar)
Answer:
The ratio of neutrons to protons in a nucleus increases after the emission of an alpha particle.

Question 31.
Which property of nuclear force explains the approximate constancy of binding energy per nucleon with mass number A for nuclei in the range 30 < A < 170? (CBSE2019C)
Answer:
The short-range nature of the nuclear force explains the approximate constancy of binding energy per nucleon with mass number A in the range 30 < A < 170.

Question 32.
Draw a graph showing the variation of decay rate with a number of active nuclei. (NCERT Exemplar)
Answer:
The graph is as shown.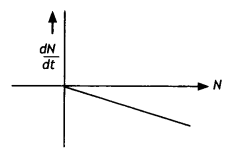

Question 33.
Which sample, A or B, shown in the figure has a shorter mean-life? (NCERT Exemplar)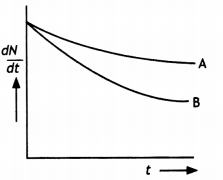

Answer:
B has shorter mean life as λ is greater forB.

Question 34.
Why do stable nuclei never have more protons than neutrons? (NCERT Exemplar)
Answer:
Protons are positively charged and repel one another electrically. This repulsion becomes so great in nuclei with more than 10 protons or so that an excess of neutrons, which produce only attractive forces, is required for stability.

Question 35.
Why does the process of spontaneous nuclear fission occur in heavy nuclei? (CBSE 2019C)
Answer:
Because heavy nuclei contain a large number of protons that exert strong repulsive forces on one another.

Short Answer Type

Question 1.
Draw the curve showing the binding energy/nucleon with a mass number of different nuclei. Briefly state, how nuclear fusion and nuclear fission can be explained on the basis of this graph.
Answer:
The diagram is as shown.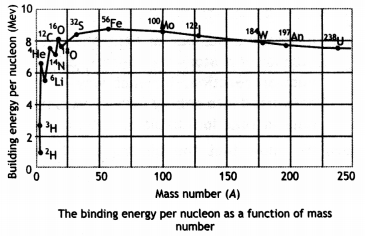

Light nuclei have a small value of binding energy per nucleon, therefore to become more stable they fuse to increase their binding energy per nucleon.

A very heavy nucleus, say A 240, has Lower binding energy per nucLeon compared to that of a nucleus with A = 120. Thus if a nucleus A = 240 breaks into two A = 120 nuclei, nucleons get more tightLy bound. This implies energy would be released in the process.

Question 2.
Define decay constant for a radioactive sample. Which of the following radiations α, β, and γ rays
(i) are similar to X-rays,
(ii) are easily absorbed by matter, and
(iii) are similar in nature to cathode rays?
Answer:
The decay constant is defined as the reciprocal of that time duration for which the number of nuclei of the radioactive sample decays to 1 / e or 37 % of its original value.
(i) Gamma
(ii) Alpha
(iii) Beta

Question 3.
State the law of radioactive decay.
Plot a graph showing the number of undecayed nuclei as a function of time (t) for a given radioactive sample having a half-life T_1/2.
Depict In the plot the number of undecayed nuclei at (i) t = 3T_1/2 and (ii) t = 5 T_1/2 (CBSE Delhi 2011)
Answer:
The number of nuclei disintegrating per second is proportional to the number of nuclei present at the time of disintegration and is independent of alt physical conditions like temperature, pressure, humidity, chemical composition, etc.

The plot is as shown.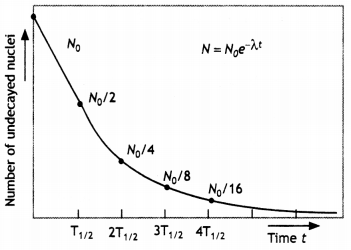

Question 4.
An electron and alpha particle have the same de- Broglie wavelength associated with them. How are their kinetic energies related to each other? (Delhi 2008)
Answer:

Question 5.
State the reason, why heavy water is generally used as a moderator in a nuclear reactor. (Delhi 2008)
Answer:
Neutrons produced during fission get slowed if they collide with a nucleus of the same mass. As ordinary water contains hydrogen atoms (of mass nearly that of neutrons), so it can be used as a moderator. But it absorbs neutrons at a fast rate via reaction :

Here d is deutron. To overcome this difficulty, heavy water is used as a moderator which has negligible cross-section for neutron absorption.

Question 6.
Name the absorbing material used to control the reaction rate of neutrons in a nuclear reactor. (Delhi 2008)
Answer:
Control rod or cadmium rod.

Question 7.
State two characteristic properties of nuclear force. (All India 2008)
Answer:
(i) Nuclear forces are the strongest force in nature.
(ii) They are saturated forces.
(iii) They are charge independent.

Question 8.
Two nuclei have mass numbers in the ratio 1: 2. What is the ratio of their nuclear densities? (Delhi 2008)
Answer: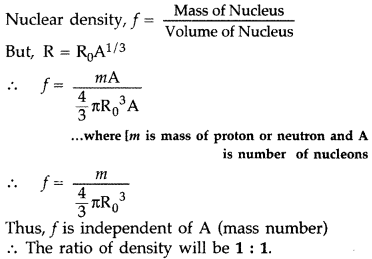

Question 9.
Two nuclei have mass number in the ratio 1 : 3. What is the ratio of their nuclear densities? (Delhi 2008)
Answer:
Since nuclear density is independent of the mass number, the ratio of nuclear densities will be 1:1.

Question 10.
Two nuclei have mass numbers in the ratio 2 : 5. What is the ratio of their nuclear densities? (Delhi 2008)
Answer:
Nuclear density is independent of mass number, so the ratio will be 1 : 1.

Question 11.
Two nuclei have mass numbers in the ratio 1: 8. What is the ratio of their nuclear radii? (All India 2008)
Answer:

Question 12.
Two nuclei have mass numbers in the ratio 8:125. What is the ratio of their nuclear radii? (All India 2008)
Answer: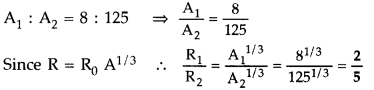

Question 13.
Two nuclei have mass numbers in the ratio 27:125. What is the ratio of their nuclear radii?
Answer: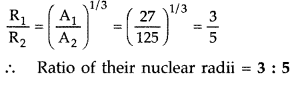

Question 14.
Write any two characteristic properties of nuclear force. (All India 2008)
Answer:
1. Nuclear forces are strongest forces in nature.
2. Nuclear forces are charge independent.

Question 15.
What is the relationship between decay constant and mean life of a radioactive nucleus? (Comptt. All India 2012)
Answer:
Relationship between decay constant and mean life of a radioactive nucleus is

Question 16.
Write the relationship between the size and the atomic mass number of a nucleus. (Comptt. All India 2012)
Answer:
Relationship between the size and the atomic mass number of a nucleus is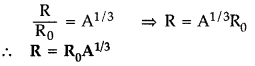

Question 16.
Define the activity of a given radioactive substance. Write its S.I. unit. (All India 2012)
Answer:
The activity of a radioactive substance is defined as the rate of disintegration of the substance. The SI unit for activity is becquerel (Bq).

Question 17.
How is the radius of a nucleus related to its mass number A? (Comptt. All India 2012)
Answer:

Question 18.
Why is it found experimentally difficult to detect neutrinos in nuclear P-decay? (All India 2012)
Answer:
It is found experimentally difficult to detect neutrinos in nuclear P-decay, because of two reasons :
(i) mass of neutrino is extremely small;
(ii) its charge is negligibly small.
Also, neutrinos interact very weakly with matter.

Question 19.
Name and define, the SI unit for the ‘activity’, of a given sample of radioactive nuclei. (Comptt. All India 2012)
Answer:
(i) becquerel is the SI unit of ‘activity’ of a nuclear sample.
(ii) One becquerel activity corresponds to ‘one decay/disintegration per second’.

Question 20.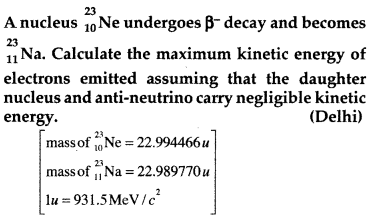

Answer: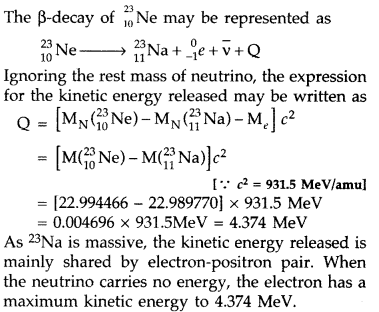

Question 21.
Calculate the energy released in MeV in the following nuclear reaction:
 (All India 2012)
Answer: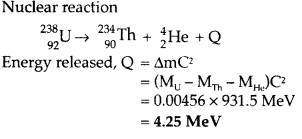

Question 22.
A radioactive nucleus ‘A’ undergoes a series of decays according to the following scheme:

The mass number and atomic number of A are 190 and 75 respectively. What are these numbers for A₄? (Delhi 2016)
Answer:

So, the Mass number of A₄ → 69
and Atomic number of A₄ → 172

Question 23.
A radio active nucleus ‘A’ undergoes a series of decays according to the following scheme:

The mass number and atomic number of A are 180 and 72 respectively. What are these numbers for A₄?
Answer:
The series can be shown as below:

So, the Mass number of A₄ is 182
and Atomic number of A₄ is 72

Question 24.
(a) The mass of a nucleus in its ground state is always less than the total mass of its constituents – neutrons and protons. Explain.
(b) Plot a graph showing the variation of potential energy of a pair of nucleons as a function of their separation. (All India 2016)
Answer:
(a) When nucleons approach each other to form a nucleus, they strongly attract each other. Their potential energy decreases and becomes negative. It is this potential energy which holds the nucleons together in the nucleus. The decrease in’ potential energy results in the decrease in the mass of the nucleons inside the nucleus.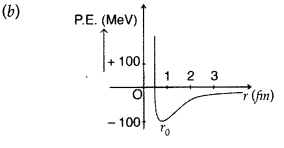

Question 25.
A heavy nucleus X of mass number 240 and binding energy per nucleon 7.6 MeV is split into two fragments Y and Z of mass numbers 110 and 130. The binding energy of nucleons in Y and Z is 8.5 MeV per nucleon. Calculate the energy Q released per fission in MeV. (Delhi 2016)
Answer:

∴ Gain in binding energy for nucleon = 8.5 – 7.6 = 0.9 MeV
Hence total gain in binding energy per nucleus fission = 240 × 0.9 = 216 MeV

Question 26.
Draw a plot of potential energy of a pair of nucleons as a function of their separation. Write two important conclusions which you can draw regarding the nature of nuclear forces. (All India 2016)
Answer:
Two important conclusions :
(i) Nuclear force between two nucleons falls rapidly to zero as their distance is more than a few femtometres. This explains constancy of the binding energy per nucleon for large-size nucleus.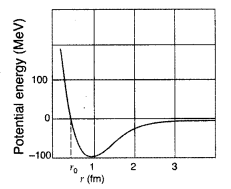

(ii) Graph explains that force is attractive for distances larger than 0.8 fin and repulsive for distances less than 0.8 fm.

Question 27.
Draw a plot of the binding energy per nucleon as a function of mass number for a large number of nuclei, 2 ≤ A ≤ 240. How do you explain the constancy of binding energy per nucleon in the range 30 < A < 170 using the property that nuclear force is short-ranged? (All India 2016)
Answer:
(a) The constancy of the binding energy in the range 30 < A < 170 is a consequence of the fact that the nuclear force is short ranged.
If a nucleon can have a maximum of p neighbours within the range of nuclear force, its binding energy would be proportional to p. Since most of the nucleons in a large nucleus reside inside it and not on the surface, the change in binding energy per nucleon would be small. The binding energy per nucleon is a constant and is approximately equal to pk. The property that a given nucleon influences only nucleons close to it, is referred to as saturation property of the nuclear force.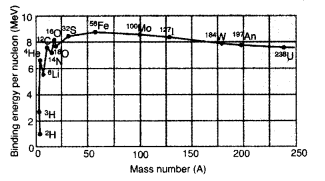

(b) Nuclear force is short-ranged for a sufficiently large nucleus. A nucleon is under the influence of only some of its neighbours, which come within the range of the nuclear force. If a nucleon can have maximum of P neighbours within the range of nuclear force, its binding energy would be proportional to ‘P’ Thus on increasing ‘A’ by adding nucleons binding energy will remain constant.

Question 28.
Using the curve for the binding energy per nucleon as a function of mass number A, state clearly how the release of energy in the processes of nuclear fission and nuclear fusion can be explained. (All India 2011)
Answer:
1. Nuclear fission : Binding energy per nucleon is smaller for heavier nuclei than the middle ones i.e. heavier nuclei are less stable. When a heavier nucleus splits into the lighter nuclei, the B.E./nucleon changes (increases) from about 7.6 MeV to 8.4 MeV. Greater binding energy of the product nuclei results in the liberation of energy. This is what happens in nuclear fission which is the basis of the atom bomb.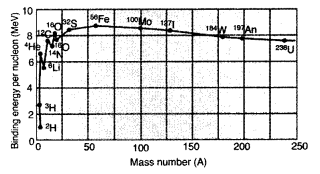

2. Nuclear fusion : The binding energy per nucleon is small for light nuclei, i.e., they are less stable. So when two light nuclei combine to form a heavier nucleus, the higher binding energy per nucleon of the latter results in the release of energy.

Question 29.
Complete the following nuclear reactions :


(Comptt. Delhi 2011)
Answer: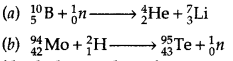

Question 30.
If both the number of protons and neutrons in a nuclear reaction is conserved, in what way is mass converted into energy (or vice verse)? Explain giving one example. (Comptt. Delhi 2011)
Answer:
Explanation for release of energy in a nuclear reaction : Since proton number and neutron number are conserved in a nuclear reaction, the total rest mass of neutrons and protons is the same on either side of the nuclear reaction.
But total binding energy of nuclei on the left side need not be the same as that on the right hand side. The difference in binding energy causes a release of energy in the reaction.
Examples :

Question 31.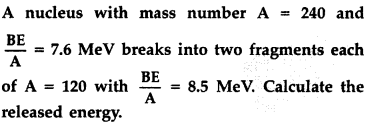

(Delhi 2016)
Answer: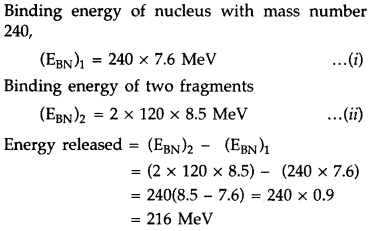

Question 32.
Calculate the energy in fusion reaction :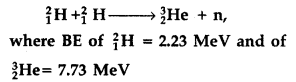

(Delhi 2016)
Answer: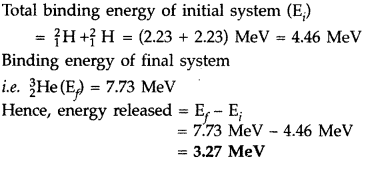

Question 33.
If both the number of protons and the number of neutrons are conserved in each nuclear reaction, in what way is mass converted into energy (or vice-versa) in a nuclear reaction? Explain. (Comptt. All India 2016)
Answer:
The number of protons and neutrons in a nuclear reaction are conserved but the total mass is not conserved.
The total mass of the free protons and neutrons is more than their total mass within the nucleus. The lost mass (= ∆m) known as ‘mass defect’, gets converted into energy as per the relation E = (∆m)c² (c is the velocity of light)

Question 34.
Write two characteristic features of nuclear force.
(b) Draw a plot of potential energy of a pair of nucleons as a function of their separation. (Comptt. All India 2017)
Answer:

(a) Nuclear forces. The strong forces of attraction which hold together the nucleons (neutrons and protons) in the tiny nucleus of an atom are called nuclear forces.
Important properties (characteristics):
1. Nuclear forces are independent of charge (These act between a pair of neutrons, between a pair of protons and between a proton and a neutron).
2. Nuclear forces are the strongest forces in nature.
3. Nuclear forces are very short range forces.
4. Nuclear forces are non-central forces.
5. Nuclear forces are dependent on spin.

(b) A plot of the potential energy between two nucleons as a function of distance is shown in the diagram.

Important conclusions from the graph :
(i) The nuclear force is much stronger than the Coulomb force acting between charges or the gravitational forces between masses. The nuclear binding force has to dominate over the Coulomb repulsive force between protons inside the nucleus. This happens only because the nuclear force is much stronger than the coulomb force. The gravitational force is much weaker than even Coulomb force.
(ii) The nuclear force between two nucleons falls rapidly to zero as their distance is more than a few femtometers. This leads to saturation of forces in a medium or a large-sized nucleus, which is the reason for the constancy of the binding energy per nucleon.
(iii) The nuclear force between neutron- neutron, proton-neutron and proton-proton is approximately the same. The nuclear force does not depend on the electric charge.

Question 35.
Write the relation between half life and decay constant of a radioactive nucleus. (Comptt. All India 2017)
Answer: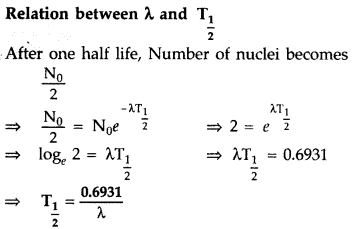

Question 36.
Two radioactive samples, X, Y have the same number of atoms at t = 0. Their half lives are 3h and 4h respectively. Compare the rates of disintegration of the two nuclei after 12 hours. (Comptt All India 2017)
Answer: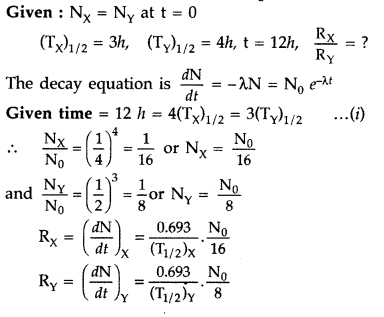

Question 37.
Distinguish between nuclear fission and fusion. Explain how the energy is released in both the processes. (Comptt. All India 2017)
Answer:
In nuclear fission a heavy nucleus breaks up into smaller nuclei accompanied by release of energy; whereas in nuclear fusion two light nuclei combine to form a heavier nucleus accompanied by release of energy.
In both the cases, some mass (= mass defect) gets converted into energy as per the relation :

Question 38.
Draw a plot of the potential energy of a pair of nucleons as a function of their separations. Mark the regions where the nuclear force is (i) attractive and (ii) repulsive. Write any two characteristic features of nuclear forces. (CBSE AI 2012)
Answer:

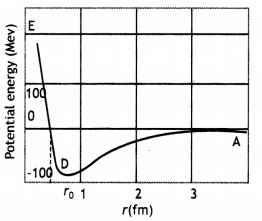
For r > r₀ (attraction), For r < r_o (repulsion)

1. Strong attractive force (stronger than the repulsive electric force between the protons)
2. Are short-range forces.

Question 39.
(a) Write the relation for binding energy (BE) (in MeV) of a nucleus of mass _Z^AM atomic number (Z) and mass number (A) in terms of the masses of its constituents – neutrons and protons.
Answer:
The required expression is
ΔE = (Zm_p + (A – Z)m_n – M) × 931 MeV

(b) Draw a plot of BE/A versus mass number A for 2 ≤ A ≤ 170. Use this graph to explain the release of energy in the process of nuclear fusion of two light nuclei. (CBSE Delhi 2014C)
Answer: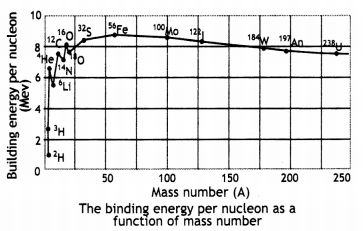

Since the binding energy of the smaller nuclei like hydrogen is less, therefore they fuse together to form helium in order to increase their binding energy per nucleon and become stable. This means that the final system is more tightly bound than the initial system. Again energy would be released in such a process of fusion.

Question 40.
If both the number of neutrons and the number of protons are conserved in each nuclear reaction, in what way is mass converted into energy (or vice versa) in a nuclear reaction? Explain. (CBSE AI2016C)
Answer:
We know that the binding energy of a nucleus gives a negative contribution to the mass of the nucleus (mass defect). Now, since proton number and neutron number are conserved in a nuclear reaction the total rest mass of neutrons and protons is the same on either side of a reaction. But the total binding energy of nuclei on the left side need not be the same as that on the right-hand side.

The difference in these binding energies appears as the energy released or absorbed in a nuclear reaction. Since binding energy contributes to mass, we say that the difference in the total mass of nuclei on the two sides gets converted into energy or vice-versa.

Question 41.
State two properties of nuclear forces. Write the relation between half-life and decay constant of a radioactive nucleus. (CBSE AI 2017C)
Answer:

1. They are saturated forces.
2. They are charge-independent.

The required relation is
T = \(\frac{\ln 2}{\lambda}=\frac{2.303 \log 2}{\lambda}=\frac{0.693}{\lambda}\)

Question 42.
(a) Draw a graph showing the variation of binding energy per nucleon (BE/A) vs mass number A for the nuclei in 20 ≤ A ≤ 170.
Answer:

Since the binding energy of the smaller nuclei like hydrogen is less, therefore they fuse together to form helium in order to increase their binding energy per nucleon and become stable. This means that the final system is more tightly bound than the initial system. Again energy would be released in such a process of fusion.

(b) A nucleus of mass number 240 and having binding energy/nucleon 7.6 MeV splits into two fragments Y, 1 of mass numbers 110 and 130 respectively. If the binding energy/ nucleon of Y, 1 is equal to 8.5 MeV each, calculate the energy released in the nuclear reaction. (CBSE Al 2017C)
Answer:
Energy released per fission
= (110 + 130) × 8.5 – 240 × 7.6
= 240 × (8.5 – 7.6) MeV
= 240 × 0.9
= 216.0 MeV

Question 43.
Explain with the help of an example, whether the neutron-proton ratio in a nucleus increases or decreases due to beta decay.
Answer:
Consider the following decay

Number of neutrons before beta decay
= 234-90 = 144
Number of neutrons after beta decay
= 234-91 =143
Number of protons before beta decay
= 90
Number of protons after beta decay
= 91
Neutron-proton ratio before beta decay
= \(\frac{144}{90}\) = 1.6

Neutron-proton ratio after beta decay
= \(\frac{143}{91}\) = 1.57

Thus neutron-proton ratio decreases during beta decay.

Question 44.
How is the size of a nucleus experimentally determined? Write the relation between the radius and mass number of the nucleus. Show that the density of the nucleus is independent of its mass number. (CBSE Delhi 2011C)
Answer:
The size of the nucleus can be determined by the Rutherford experiments on alpha particles scattering. The distance of the nearest approach is approximately the size of the nucleus. Here it is assumed that only coulomb repulsive force caused scattering. With alpha rays of 5.5 MeV, the size of the nucleus was found to be less than 4 × 10^-14 m. By doing scattering experiments with fast electrons bombarding targets of different elements, the size of the nuclei of various elements determined accurately.

The required relation is
R = R_oA₁/3, where R_o = 1.2 × 10^-15 m

The density of a nucleus of mass number A and radius R is given by

which is independent of the mass number A.

Question 45.
(a) What characteristic property of nuclear force explains the constancy of binding energy per nucleon (BE/A) in the range of mass number ‘A’ lying 30 < A < 170?
Answer:
The nuclear force between two nucleons falls rapidly to zero as their distance is more than a few femtometres. This leads to the saturation of forces in a medium or a large-sized nucleus, i.e. nuclei for which A is 30 < A < 170, which is the reason for the constancy of the binding energy per nucleon.

(b) Show that the density of nucleus over a wide range of nuclei is constant- independent of mass number A. (CBSE AI 2012)
Answer:
The size of the nucleus can be determined by the Rutherford experiments on alpha particles scattering. The distance of the nearest approach is approximately the size of the nucleus. Here it is assumed that only coulomb repulsive force caused scattering. With alpha rays of 5.5 MeV, the size of the nucleus was found to be less than 4 x 10^-14 m. By doing scattering experiments with fast electrons bombarding targets of different elements, the size of the nuclei of various elements determined accurately.

The required relation is
R = R_oA₁/3, where R_o = 1.2 × 10^-15 m

The density of a nucleus of mass number A and radius R is given by

which is independent of the mass number A.

Question 46.
A radioactive nucleus ‘A’ decays as given below:
.
If the mass number and atomic number of A₁ are 180 and 73 respectively, find the mass number and an atomic number of A and A₂.
Answer:
For A : Z = 72 and A = 180
For A₂: Z = 71 and A = 176

Question 47.
The sequence of stepwise decay of a radioactive nucleus is. If the nucleon number and atomic number for D₂ are 176 and 71 respectively, what are the corresponding values of D and D₃? Justify your answer in each case.
Answer:
For D: A = 180, Z = 72
For D₃: A = 172, Z = 69

During alpha decay mass number decreases by 4 and the atomic number decreases by 2, while during beta decay the mass number remains the same, and the atomic number increases by 1.

Question 48.
Write symbolically the nuclear β⁺ decay process of ₆¹¹C. Is the decayed product X an isotope or isobar of₆¹¹C?
Given the mass values m (₆¹¹C) = 11.011434 u and m (X) = 11.009305 u. (CBSE AI 2015)
Estimate the Q – value in this process.
Answer:
The required equation is

X is an isobar
Mass defect = m(C) – m(X)
= (11.011434- 11.009305) u = 0.002129 u

Therefore Q = Δm × 931.5 MeV
= 0.002129 × 931.5 = 1.98 MeV

Question 49.
Two radioactive samples, X, Y have the same number of atoms at t = 0. Their half¬lives are 3 h and 4 h respectively. Compare the rates of disintegration of the two nuclei after 12 hours. (CBSE AI 2017C)
Answer:
Let N0 be the nuclei present in X and Y at t = 0. Given T_x = 3 h and T_y = 4 h, t = 12 h.
The number of nuclei present in X and Y after 12 hours is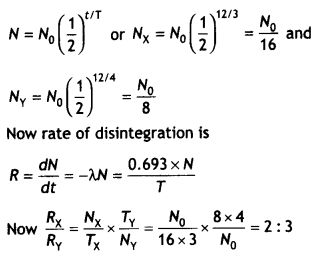

Question 50.
A radioactive sample has the activity of 10,000 disintegrations per second after 20 hours. After the next 10 hours, its activity reduces to 5,000 dis. sec^-1. Find out its half-life and initial activity. (CBSE Delhi 2017C)
Answer:
Since activity reduces to half in 10 hours from 10000 dis. sec^-1 to 5000 dis. sec^-1, therefore half-life of the sample will be 10 years.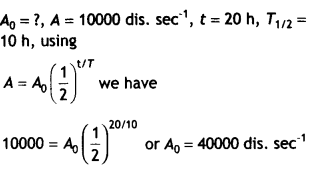

Question 51.
Why is the energy of the beta particles emitted during beta decay continuous?
Answer:
The phenomenon of beta decay arises due to the conversion of a neutron in the nucleus into a proton, electron, and an anti-neutrino. Because the energy available in beta decay is shared by the electron and the anti-neutrino in all possible ratios as they come out of the nucleus, therefore the beta ray energy spectrum is continuous in nature.

Question 52.
Explain, how radioactive nuclei can emit β-particles even though atomic nuclei do not contain these particles. Hence explain why the mass number of a radioactive nuclide does not change during β-decay.
Answer:
Beta-particles (or electrons) as such are not present inside a nucleus. However, in the case of a radioactive nuclide, sometimes a neutron decays into a proton, an electron, and an antineutrino as given by the following equation:

where mass and charge of antineutrino particle is zero. Out of the particles formed, the proton remains within the nucleus itself but electron along with antineutrino comes out of the nucleus. It is this electron that is being emitted as a beta-particle.

As in the process of β-emission, one proton is produced in the nucleus at the expense of a neutron and the mass number of both is the same, hence the mass number of the nuclide remains unchanged during p-decay.

Question 53.
Consider a radioactive nucleus A which decays to a stable nucleus C through the following sequence: A → B → C. Here B is an intermediate nucleus that is also radioactive. Considering that there are N_o atoms of A initially, plot the graph showing the variation of the number of atoms of A and B versus time. (NCERT Exemplar)
Answer:
At t = 0, N_A = N_o while N_B = 0. As time increases, N_A falls off exponentially, while the number of atoms of B increases, becomes maximum, and finally decays to zero  ∞ (following exponential decay law).

Hence the graph is as shown.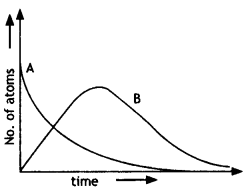

Question 54.
Draw a plot showing the variation of binding energy per nucleon versus the mass number (A). Explain with the help of this plot the release of energy in the processes of nuclear fission and fusion. (All India 2009)
Answer:
1. Nuclear fission : Binding energy per nucleon is smaller for heavier nuclei than the middle ones i.e. heavier nuclei are less stable. When a heavier nucleus splits into the lighter nuclei, the B.E./nucleon changes (increases) from about 7.6 MeV to 8.4 MeV. Greater binding energy of the product nuclei results in the liberation of energy. This is what happens in nuclear fission which is the basis of the atom bomb.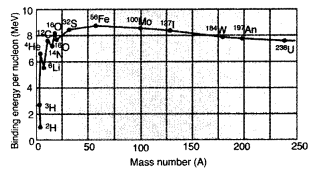

2. Nuclear fusion : The binding energy per nucleon is small for light nuclei, i.e., they are less stable. So when two light nuclei combine to form a heavier nucleus, the higher binding energy per nucleon of the latter results in the release of energy.

Question 55.
Define the activity of a radionuclide. Write its S.I. unit. Give a plot of the activity of a radioactive species versus time.
How long will a radioactive isotope, whose half life is T years, take for its activity to reduce to 1/8^th of its initial value? (All India 2009)
Answer:
Activity: It is defined as the total decay rate of a sample of one or more radionuclide.
Its S.I. unit is bequerel
I bequerel = 1 decay per second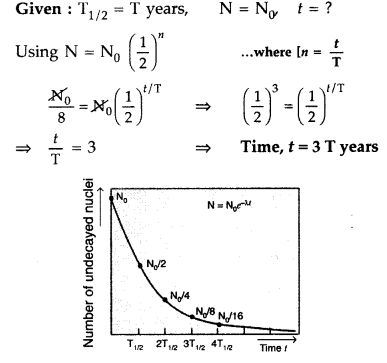

Question 56.
(i) Define ‘activity’ of a radioactive material and write its S.I. unit.
(ii) Plot a graph showing variation of activity of a given radioactive sample with time.
(iii) The sequence of stepwise decay of a radioactive nucleus is

If the atomic number and mass number of D₂, are 71 and 176 respectively, what are their corresponding values for D?
Answer:
Activity: It is defined as the total decay rate of a sample of one or more radionuclide.
Its S.I. unit is bequerel
I bequerel = 1 decay per second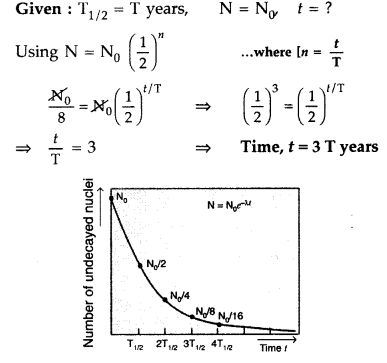

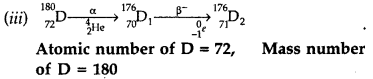
Question 57.
(a) Write symbolically the P“ decay process of \(\begin{array}{l}{32} \\ {15}\end{array}\).
(b) Derive an expression for the average life of a radionuclide. Give its relationship with the half-life. (All India 2009)
Answer:

(b) Suppose a radioactive sample contains N0 nuclei at time t = 0. After time this number reduces to N. Furthermore, suppose dN nuclei disintegrates in time t to t + dt. As dt is small so the life of each of the dN nuclei can be approximately taken equal to t
∴ Total life of dN nuclei = tdN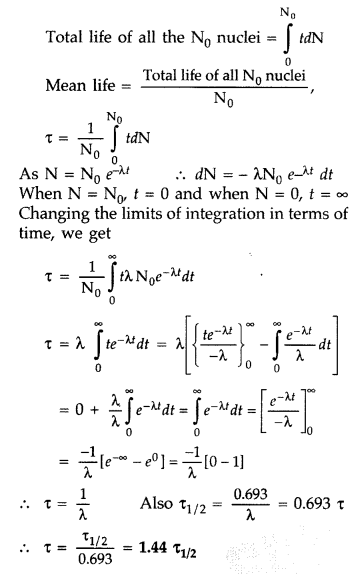

Question 58.
State the law of radioactive decay.
Plot a graph showing the number (N) of undecayed nuclei as a function of time (t) for a given radioactive sample having half life T_1/2. Depict in the plot the number of undecayed nuclei at
(i) t = 3 T_1/2 and
(ii) t = 5_1/2.(DeIhi 2009)
Answer:
The number of nuclei undergoing decay per unit time, at any instant is proportional to number of nuclei in the sample at that instant. The given figure shows a graph between the number of undecayed nuclei as a function of time.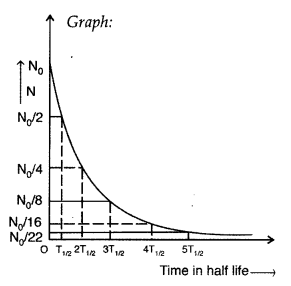

Question 59.
(i) What characteristic property of nuclear force explains the constancy of binding energy per nucleon (BE/A) in the range of mass number ‘A’ lying 30 < A < 170?
(ii) Show that the density of nucleus over a wide range of nuclei is constant- independent of mass number A. (Delhi 2009)
Answer:
(i) Saturation is the Short range nature of nuclear forces
(ii) Let A be the mass number and R be the radius of a nucleus
If m is the average mass of a nucleon, then
Mass of nucleus = mA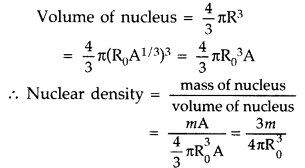

Clearly, nuclear density is independent of mass number A or the size of the nucleus.

Question 60.
Draw a plot of potential energy of a pair of nucleons as a function of their separations. Mark the regions where the nuclear force is
(i) attractive and
(ii) repulsive. Write any two characteristic features of nuclear forces.
Answer:
The graph indicates that the attractive force between the two nucleons is strongest at a separation r₀ = 1 fm. For a separation greater than the force is attractive and for separation less than r₀, the force is strongly repulsive.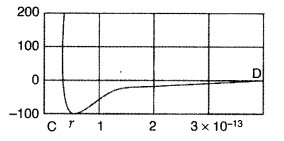

Two characteristic features of nuclear forces :
1. Strongest interaction
2. Short-range force
3. Charge independent character (any two)

Question 61.
Answer the following, giving reasons:
(i) Why is the binding energy per nucleon found to be constant for nuclei in the range of mass number (A) lying between 30 and 170?
(ii) When a heavy nucleus with mass number A = 240 breaks into two nuclei, A = 120, energy is released in’ the process.
(iii) In β-decay, the experimental detection of neutrinos (or antineutrinos) is found to be extremely difficult. (Comptt. All India 2011)
Answer:
(i) Nuclear forces are short ranged. For a particular nucleon inside a sufficiently large nucleus will be under the influence of some of its neighbours which come within the range of the nuclear force. The property that a given nucleon influences only nucleons close to it is also referred to as saturation property of the nuclear force.
(ii) The binding energy per nucleon of the parent nucleus is less than those of the two daughter nuclei. It is this increased binding energy that gets released in this process.
(iii) Neutrinos are chargeless and massless particles, whose interaction with other particles is almost negligible. Hence, they can pass through very large quantity of matter with-out getting detected.

Question 62.
(a) In a typical nuclear reaction, e.g.

although number of nucleons is conserved, yet energy is released. How? Explain.
(b) Show that nuclear density in a given nucleus is independent of mass number A. (Delhi 2011)
Answer:
(a) In all types of nuclear reactions, the law of conservation of number of nucleons is followed. But during the reaction, the mass of the final product is found to be slightly less than the sum of the masses of the reactant components. This difference in mass of a nucleus and its constituents is called mass defect. So, as per mass energy relation E = (∆M)c², energy is released. In the given reaction the sum of the masses of two deutrons is more than the mass of helium and neutron. Energy equivalent of mass defect is released.

Question 63.

though the conserved on both sides of the reaction, yet the energy is released. How? Explain.
(b) Draw a plot of potential energy between a pair of nucleons as a function of their separation. Mark the regions where potential energy is
(i) positive and
(ii) negative. (Delhi 2011)
Answer:
(a) Since the total binding energy of nuclei on the left side of the reaction is not the same as the total binding energy of nucleus on the right hand side, this difference of binding energy appears as the energy released.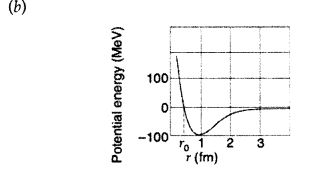

For separation (r) ≤ 0.8 fermi
Force is repulsive
For r > 0.8 fermi force will be attractive.

Question 64.
(a) The number of nuclei of a given radioactive sample at time t = 0 and t = T are N₀ and N₀/n respectively. Obtain an expression for the half-life (T_1/2) of the nucleus in terms of n and T.
(b) Write the basic nuclear process underlying β-decay of a given radioactive nucleus. (Comptt. Delhi 2013)
Answer:
(a) Decay constant. It is the reciprocal of the time interval in which the number of active nuclei in a radioactive sample reduces to 1/e times of its initial nuclei.
Half life period. It is the time during which half the total number of atoms in radioactive elements (N₀) disintegrates. It is denoted by t_1/2.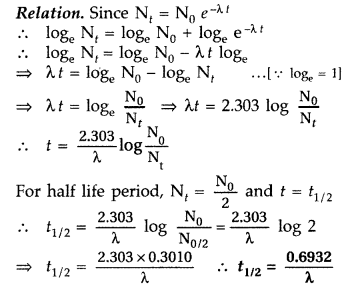

(b) β-decay. It is the phenomenon of emission of an electron from a radioactive nucleus. In Beta-minus decay, a neutron transforms into a proton within the nucleus. According to

The emission of β-particle from an atom will change it into a new atom whose atomic number is increased by one without changing its mass number.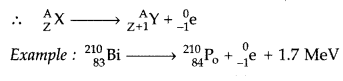

Question 65.


Answer:
(a) Radioactive decay law. It states that “the number of atoms disintegrated per second at any instant is directly proportional to the number of radioactive atoms actually present at that time.” Let N₀ be the total number of atoms present at time t = 0 (initially)
and N be the total number of atoms present at time t, then
According to radioactive decay law, the rate of disintegration at any time t is directly proportional to the number of atoms present at that time t.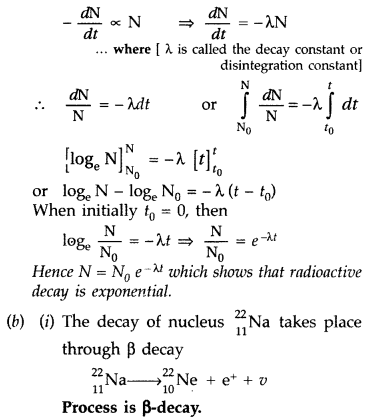

(ii) Since new nucleus has the same mass number, hence it would be an Isobar.

Question 66.
Write the relation for binding energy (BE) (in MeV) of a nucleus of \(_{Z}^{A} N\), atomic number (Z) and mass number (A) in terms of the masses of its constituents – neutrons and protons.
(b) Draw a plot of BE/A versus mass number A for 2 ≤ A ≤ 170. Use this graph to explain the release of energy in the process of nuclear fusion of two light nuclei. (Comptt. Delhi 2014)
Answer:
(a) Mass defect. The difference between the sum of the masses of neutrons and protons forming a nucleus and mass of the nucleons is called mass defect.

(b)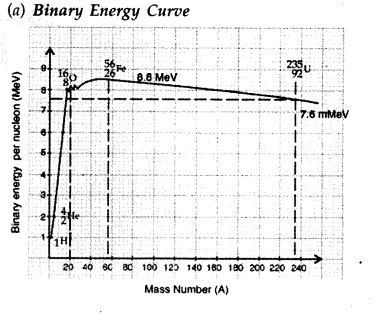

Conclusions :
(i) The force is attractive and sufficiently strong to produce a binding energy of a few MeV per nucleon.

(b) (i) When we move from heavy nuclei region to middle region, we find that there will be a gain in overall binding energy and hence release of energy. This indicates that energy can be released when a heavy nucleus breaks into two roughly equal fragments/nuclear fission.
(ii) Similarly, when we move from lighter nuclei to heavier nuclei, we find that there will be gain in overall binding energy and hence release of energy. This indicates that energy can be released when two lighter
nuclei fuse together to form heavy nucleus/nuclear fusion.
(c) In Beta decay a neutron breaks into a proton, electron and neutrino as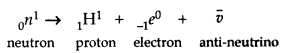

Detection of neutrinos is difficult because they are chargeless and have either no or low mass.

Question 67.
(a) Define the term ‘activity of a sample of radioactive nucleus. Write its S.I. unit.

(Comptt. All India 2014)
Answer:
(a) The activity of a radioactive nucleus equals its decay rate (or number of nuclei decaying per unit time)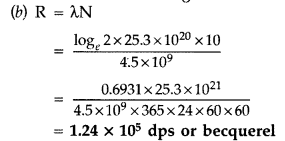

Question 68.
(i) Write the relation between ‘average life’ and ‘half-life’ of a radioactive nucleus.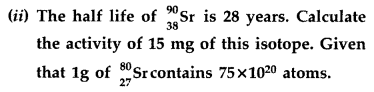


Answer: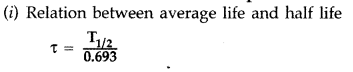

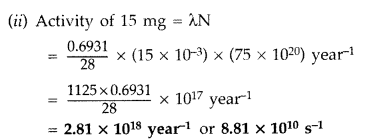
Question 69.
(i) Define the term ‘mass defect’ of a nucleus. How is it related with its binding energy?
(ii) Determine the Q-value of the following reaction: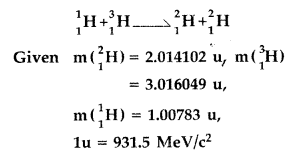

(Comptt. All India 2014)
Answer:
(i) (a) The mass defect of a nucleus equals the difference between the total mass of its constituents and the mass of the nucleus itself.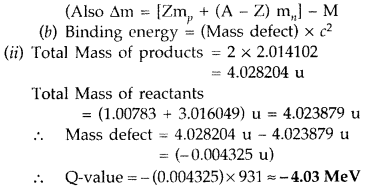

Question 70.
Distinguish between nuclear fission and fusion. Show how in both these processes energy is released.
Calculate the energy release in MeV in the deuterium-tritium fusion reaction :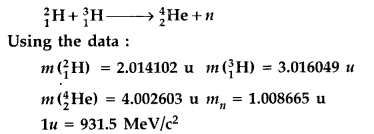

(Delhi 2015)
Answer:
(a) The breaking of heavy nucleus into smaller fragments is called nuclear fission; while the joining of lighter nuclei to form a heavy nucleus is called nuclear fusion.
(b) Binding energy per nucleon of the daugher nuclei, in both processess, is more than that of the parent nuclei. The difference in binding energy is released in the form of energy. In both processes some mass gets converted into energy.
(c) Energy released

Question 71.
(a) Write three characteristic properties of nuclear force.
(b) Draw a plot of potential energy of a pair of nucleons as a function of their separation. Write two important conclusions that can be drawn from the graph. (All India 2015)
Answer:
(a) Nuclear forces. The strong forces of attraction which hold together the nucleons (neutrons and protons) in the tiny nucleus of an atom are called nuclear forces.
Important properties (characteristics):
1. Nuclear forces are independent of charge (These act between a pair of neutrons, between a pair of protons and between a proton and a neutron).
2. Nuclear forces are the strongest forces in nature.
3. Nuclear forces are very short range forces.
4. Nuclear forces are non-central forces.
5. Nuclear forces are dependent on spin.

(b) A plot of the potential energy between two nucleons as a function of distance is shown in the diagram.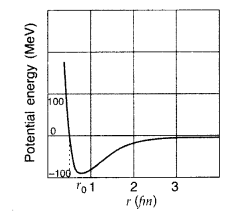

Important conclusions from the graph :
(i) The nuclear force is much stronger than the Coulomb force acting between charges or the gravitational forces between masses. The nuclear binding force has to dominate over the Coulomb repulsive force between protons inside the nucleus. This happens only because the nuclear force is much stronger than the coulomb force. The gravitational force is much weaker than even Coulomb force.
(ii) The nuclear force between two nucleons falls rapidly to zero as their distance is more than a few femtometers. This leads to saturation of forces in a medium or a large-sized nucleus, which is the reason for the constancy of the binding energy per nucleon.
(iii) The nuclear force between neutron- neutron, proton-neutron and proton-proton is approximately the same. The nuclear force does not depend on the electric charge.

Question 72.
Complete the following nuclear reactions :

(c) Why is it found experimentally difficult to detect neutrinos? (Comptt. All India 2017)
Answer:

It is found experimentally difficult to detect neutrinos in nuclear P-decay, because of two reasons :
(i) mass of neutrino is extremely small;
(ii) its charge is negligibly small.
Also, neutrinos interact very weakly with matter.

Question 73.
(a) Write the basic nuclear process involved in the emission of β⁺ in a symbolic form, by a radioactive nucleus.
(b) In the reactions given below :

Find the values of x, y, and z and a, b and c. (All India 2017)
Answer: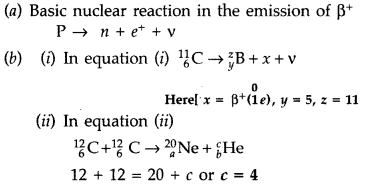

Question 74.
Obtain the relation \(\mathbf{N}=\mathbf{N}_{0} e^{-\lambda t}\) for a sample of radioactive material having decay constant λ, where N is the number of nuclei present at constant λ. Hence obtain the relation between decay constant λ and half life \(\mathbf{T}_{\frac{1}{2}}\) of the sample. (Comptt. Delhi 2017)
Answer:
(i)
(a) Radioactive decay law. It states that “the number of atoms disintegrated per second at any instant is directly proportional to the number of radioactive atoms actually present at that time.” Let N0 be the total number of atoms present at time t = 0 (initially)
and N be the total number of atoms present at time t, then
According to radioactive decay law, the rate of disintegration at any time t is directly proportional to the number of atoms present at that time t.

(ii) Relation between X and \(\mathbf{T}_{\frac{1}{2}}\)
After one half life, Number of nuclei becomes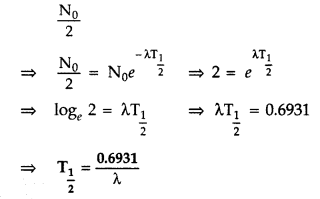

Question 75.
(i) A radioactive nucleus ‘A’ undergoes a series of decays as given below:

The mass number and atomic number of A₂ are 176 and 71 respectively.
Determine the mass and atomic numbers of A₄ and A.


Answer: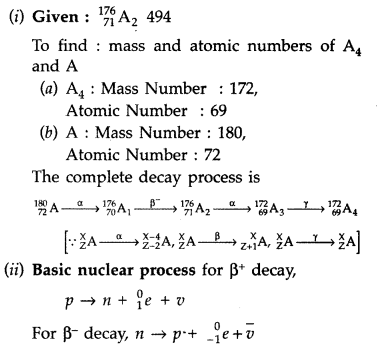

Question 76.
(a) Draw a graph showing the variation of binding energy per nucleon (BE/A) vs mass number A for the nuclei in 20 ≤ A ≤ 170.
(b) A nucleus of mass number 240 and having binding energy/nucleon 7.6 MeV splits into two fragments Y, Z of mass numbers 110 and 130 respectively. If the binding energy/ nucleon of Y, Z is equal to 8.5 MeV each, calculate the energy released in the nuclear reaction. (Comptt. All India 2017)
Answer: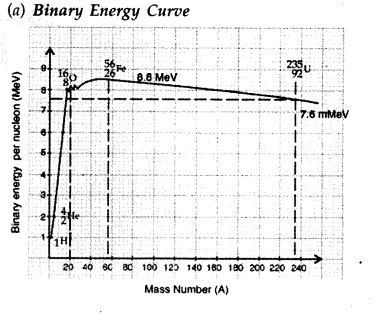

Conclusions :
(i) The force is attractive and sufficiently strong to produce a binding energy of a few MeV per nucleon.

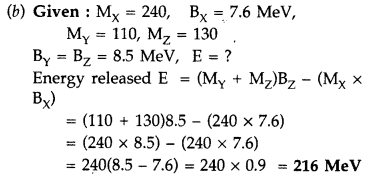
Long Answer Type

Question 1.
Define the terms: half-life period and decay constant of a radioactive sample. Derive the relation between these terms.
Answer:
The half-life is the time required for the number of radioactive nuclei to decrease to one-half the original number.

The decay constant is defined as the reciprocal of that time duration for which the number of nuclei of the radioactive sample decays to 1 / e or 37% of its original value.

To get the relation for half life T and decay constant λ we set N = \(\frac{N_{0}}{2}\) and t = T in the equation N = No e^-λt, obtaining \(\frac{1}{2}\) = e^-λt

Taking the logarithm of both sides and solving for T we have
T = \(\frac{\ln 2}{\lambda}=\frac{2.303 \log 2}{\lambda}=\frac{0.693}{\lambda}\)

Question 2.
(a) Draw a graph showing the variation of the potential energy of a pair of nucleons as a function of their separation. Indicate the regions in which nuclear force is (i) attractive, and (ii) repulsive.
Answer:
Graph showing the variation of potential energy U (in MeV) of a pair of nucleons as a function of their separation r (in fm) is shown here.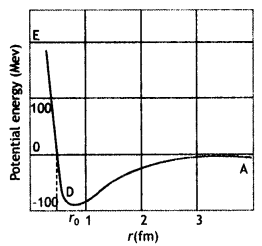

1. In the graph region AD (r > r_o) shows the region where nuclear force is strongly attractive.
2. The region DE (r < r_o) shows the region where nuclear force is strongly repulsive.

(b) Write two characteristic features of nuclear force which distinguish it from the Coulomb force.
Answer:
Two characteristics of nuclear forces which distinguish it from Coulomb’s force are

• It is charge Independent.
• It is an extremely short-range force and does not obey the inverse square law.

Question 3.
Prove that the Instantaneous rate of change of the activity of a radioactive substance is Inversely proportional to the square of Its half-life.
Answer:
The activity of a radioactive substance is
A = \(\frac{dN}{dt}\).

We know that the number of nuclei of a radioactive substance Left behind after time t is given by N = N_oe^-λt

Differentiating the above relation with respect to time we have
A = \(\frac{d N}{d t}=\frac{d}{d t}\)N_oe^-λt = – N_oλe^-λt

Differentiating the above equation with respect to time we have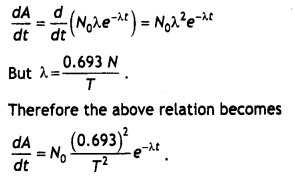

Therefore the Instantaneous rate of change of the activity of a radioactive substance is inversely proportional to the square of Its half Life.

Question 4.
(a) Deduce the expression N = N_oe^-λt the law of radioactive decay.
(b) (i) Write symbolically the process expressing the β⁺ decay of, ₁₁²²Na Also write the basic nuclear process underlying this decay.
(ii) Is the nucleus formed in the decay of the nucleus ₁₁²²Na an Isotope or isobar? (CBSE Delhi 2014)
Answer:
(a) Let N0 be the number of nuclei present in a freshly separated sample of a radioactive substance. Let after time t the number of nuclei left behind be N. Let dN number of nuclei disintegrate in a small time interval dt. Then by the – decay law,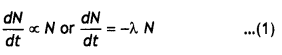

where λ  is a constant of proportionality.

Question 5.
(a) Complete the following nuclear reactions: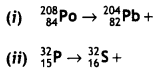

Answer:

(b) Write the basic process Involved in nuclei responsible for (i) β^– and (ii) β⁺ decay.
Answer:
The basic nuclear process underlying β^– decay is the conversion of the neutron to proton
n → p + e^– + v^–
while for v+ decay, it is the conversion of a proton into a neutron
p → n + e⁺ + v

(c) Why is it found experimentally difficult to detect neutrinos? (CBSE AI 2015 C)
Answer:
Neutrinos are neutral particles with very small (possibly, even zero) mass compared to electrons. They have only weak interaction with other particles. They are, therefore, very difficult to detect, since they can penetrate a large quantity of matter (even earth) without any interaction.

Question 6.
(a) Explain the processes of nuclear fission and nuclear fusion by using the plot of binding energy per nucleon (B.E./A) versus the mass number A.
Answer:
For the graph

From the plot, we note that

• During nuclear fission: A heavy nucleus in the larger mass region (A > 200) breaks into two middle-level nuclei, resulting in an increase in B.E./ nucleon. This results in the release of energy,
• During nuclear fusion: Light nuclei in the lower mass region (A < 20) fuse to form a nucleus having higher B.E. / nucleon. Hence Energy gets released.

(b) A radioactive Isotope has a half-life of 10 years. How long will It take for the activity to reduce to 3.125%? (CBSE AI2018)
Answer;
3.125% means that the number of nuclei decays to 1/32 of its original value.
Therefore,
\(\frac{N}{N_{0}}=\frac{1}{32}=\left(\frac{1}{2}\right)^{5}\)

Now we know that
N = N0\(\left(\frac{1}{2}\right)^{t / T}\)

Therefore we have
\(\left(\frac{1}{2}\right)^{5}=\left(\frac{1}{2}\right)^{t / T}\)

Therefore
t = 5T = 5 × 10 = 50 years

Question 7.
Group the following six nuclides into three pairs of (?) isotones, (ii) isotopes, and (iii) isobars: ₆¹²C, ₂³He, ₈₀¹⁹⁸Hg, ₁³H, ₇₉¹⁹⁷Au ₆¹⁴C. How does the size of the nucleus depend on its mass number? Hence explain why the density of nuclear matter should be independent of the size of the nucleus.
Answer:
(a) Isotones: ₈₀¹⁹⁸Hg, ₇₉¹⁹⁷Au
(6) Isotopes: ₆¹²C , ₆¹⁴C
(c) Isobars: ₂³He, ₁³H

The size of a nucleus depends upon its mass number as R = R₀ A^1/3

The nuclear density is given by the expression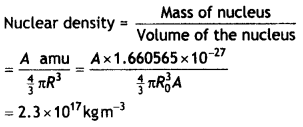

The calculations show that the nuclear density is independent of the mass number.

Question 8.
Define the term ‘decay constant’ of a radioactive sample. The rate of disintegration of a given radioactive nucleus is 10,000 disintegrations/s and 5,000 disintegration/s after 20 hr and 30 hr respectively from start. Calculate the half-life and an initial number of nuclei at t = 0. (CBSE Delhi 2019)
Answer:
The decay constant of a radioactive element is the reciprocal of the time in which the number of its nuclei reduces to 1 /e of its original number.

We have R = λN
R(20hrs) = 100o0 = λN₂₀
R(30hrs) = 5000 = λN₃₀
\(\frac{N_{20}}{N_{30}}\) = 2

This means that the number of nuclei, of the given radioactive nucleus, gets halved in a time of (30 – 20) hours = 10 hours
Half-life = 10 hours

This means that in 20 hours (= 2 half-Lives), the original number of nuclei must have gone down by a factor of 4.
Hence rate of decay at t = 0
λ N₀ = 4 λ N₂₀
R₀ = 4 × 10,000 = 40,000 disintegration per second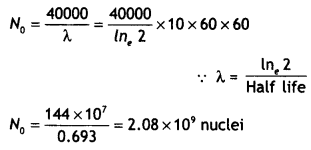

Question 9.
(a) Write the relation between half-life and an average life of a radioactive nucleus.
Answer:
The relation is τ = 1 .44T^1/2

(b) In a given sample two isotopes A and B are initially present in the ratio of 1:2. Their half-lives are 60 years and 30 years respectively. How long will it take so that the sample has these isotopes in the ratio of 2:1? (CBSE Delhi 2019)
Answer:


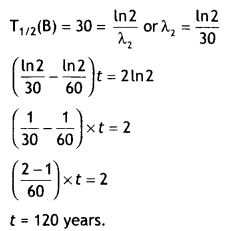
Question 10.
Distinguish between nuclear fission and fusion. Show how in both these processes energy is released. Calculate the energy release in MeV in the deuterium-tritium fusion reaction:

Using the data m(21H) = 2.014102 u,
m(₁³H) = 3.016949u, m(₂⁴He) = 4.002603 u,
m_n = 1.008665 u,1 u = 931.5 MeV/c² (CBSE Delhi 2015)
Answer:
The distinction is shown in the table below.

Nuclear Fission	Nuclear Fusion
1. It is the splitting of a heavy nucleus into two or tighter unstable nuclei.	1. It is the combining of two light nuclei into a heavier nucleus.
2. It may or may not be a chain reaction.	2. It is always a chain reaction.
3. It is independent of temperature.	3. It is temperature-dependent.
4. It can be controlled.	4. It can’t be controlled.
5. Tremendous amount of energy is released.	5. Energy released per unit mass is seven times the energy released during fission.
6. By-products are harmful.	6. By-products are not harmful.
7. Example of reaction – The atom bomb.	7. Example of reaction – Reaction in stars, hydrogen born

In both reactions, there is a mass defect that is converted into energy.
Now energy released in the reaction

Question 11.
(a) Draw a plot showing the variation of the potential energy of a pair of nucleons as a function of their separation. Mark the regions where the nuclear force is (a) attractive and (b) repulsive.
Answer: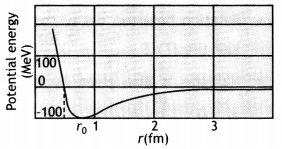

For r > r_o, the force is attractive For r < r_o, the force is repulsive

(b) In the nuclear reaction

determine the values of a and b. (CBSE Delhi 2018 C)
Answer:
We have,
1 + 235 = a + 94 + 2 × 1
∴ a = 236 – 96 = 140

Also
0 + 92 = 54+ 6 + 2 × 0
∴ b = 92 – 54 = 38

Question 12.
Binding energy per nucleon versus mass number curve is as shown. _Z^AS, _Z1^A1w, _Z2^A2X, and _Z3^A3Y, are four nuclei indicated on the curve.

Based on the graph:
(а) Arrange X, W, and S in the increasing order of stability.
Answer:
(a) S, W, and X

(b) Write the relation between the relevant A and Z values for the following nuclear reaction. S → X + W
Answer:
The equation is
Z = Z₁ + Z₂ and A = A₁ + A₂

(c) Explain why binding energy for heavy nuclei is low. (CBSE Sample Paper 2018-19)
Answer:
Reason for low binding energy: For heavier nuclei, the Coulomb repulsive force between protons increases considerably and offsets the attractive effects of the nuclear forces. This can result in such nuclei being unstable.

Question 13.
(a) Derive the law of radioactive decay,
viz. N = Noe-λt
Answer:
Let N0 be the number of nuclei present in a freshly separated sample of a radioactive substance. Let after time t the number of nuclei left behind be N. Let dN number of nuclei disintegrate in a small time interval dt. Then by the – decay law,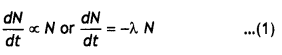

where λ is a constant of proportionality.

(b) Explain, giving necessary reactions, how energy is released during
(i) fission
Answer:
Nuclear Fission: It is a process in which a heavy nucleus splits up into two Lighter nucLei of nearly equal masses. It is found that the sum of the masses of the product nuclei and particles is less than the sum of the masses of the reactants, i.e. there is some mass defect. This mass defect appears as energy. One such fission reaction is given below: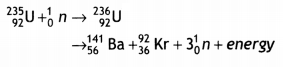

The Q value of the above reaction is about 200 MeV. The sum of the masses of Ba, Kr, and 3 neutrons is less than the sum of the masses of U and one neutron.

(ii) fusion
Nuclear Fusion: It is the process in which two light nuclei combine together to form a heavy nucleus. For fusion very high temperature of l is required. One such fusion reaction is given below:

The Q value of this nuclear reaction is 24 MeV. It is the energy equivalent of the mass defect in the above reaction. The energy released in fusion is much less than in fission but the energy released per unit mass infusion is much greater than that released in fission.

Question 14.
(a) Distinguish between isotopes and isobars, giving one example for each.
(b) Why is the mass of a nucleus always less than the sum of the masses of its constituents? Write one example to justify your answer.
Or
(a) Classify the following six nuclides into (i) isotones, (ii) Isotopes, and (iii) isobars: (CBSEAI2019)

(b) How does the size of a nucleus depend on its mass number? Hence explain why the density of nuclear matter should be independent of the size of the nucleus.
Answer:
(a) Isotopes have the same atomic number while isobars have the same mass number
Examples of isotopes ₆¹²C, ₆¹⁴C
Examples of isobars ₂³He, ₁³H

(b) Mass of a nucleus is less than its constituents because in the bound state some mass is converted into binding energy which is energy equivalent of mass defect e.g., the mass of 1860 nucleus is less than the sum of masses of 8 protons and 8 neutrons
Or
(a) Isotones: ₈₀¹⁹⁸Hg, ₇₉¹⁹⁷Au
(6) Isotopes: ₆¹²C , ₆¹⁴C
(c) Isobars: ₂³He, ₁³H

(b) The radius of the nucleus is given by
R = R_oA^1/3

Volume of the nucleus \(\frac{4}{3}\)πR³ = \(\frac{4}{3}\)πR_o³ A

If m is the average mass of the nucleon then the mass of the nucleus M = mA
Hence nuclear density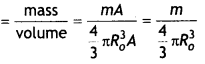

Which is independent of the A i.e., the size of the nucleus.

Numerical Problems:

• Radius of the nucleus R = R_oA^1/3
• Mass defect, Δm = Z m_p + (A – Z) m_n– M
• Energy released ΔE = Δm × 931 MeV
  or
  ΔE = [Z m_p + (A – Z) m_n – M] × 931 MeV
• Binding energy per nucleon BE/N = ΔE/A
• Relation between original nuclei (N) and nuclei left (N_o) after time t N = N_oe^-λt
• Relation between decay constant (λ) and half-life (T) = \(\frac{\ln 2}{\lambda}=\frac{2.303 \log 2}{\lambda}=\frac{0.693}{\lambda}\)
• Half-life is also given by the expression N = N_o\(\left(\frac{1}{2}\right)^{n}\) where n = t/T
• The average life is given by
  τ = \(\frac{1}{\lambda}=\frac{T}{\ln 2}=\frac{T}{0.693}\) = 1.44 T
• Activity is given by A = -λN = \(\frac{0.693N}{T}\)

Question 1.
Calculate the binding energy per nucleon of Fe⁵⁶₂₆ Given m_Fe = 55.934939 u, m_n = 1.008665 u and m_p = 1.007825 u
Answer:
Number of protons Z = 26
Number of neutrons (A – Z) = 30
Now mass defect is given by
Δm = Z m_p + (A – Z)m_n – M
Δm = 26 × 1.007825 + 30 × 1.008665 – 55.934939
= 0.528461 u

Therefore binding energy
BE = Δm × 931 MeV = 0.528461 × 931
= 491.99 MeV

BE/nucleon = 491.99/56 = 8.785 MeV

Question 2.
The activity of a radioactive element drops to one-sixteenth of its initial value in 32 years. Find the mean life of the sample.
Answer: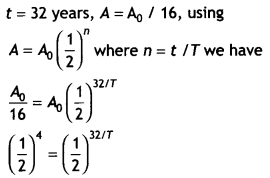

Or
32/T = 4 or 7 = 32 / 4 = 8 years.
Therefore mean life of the sample is τ = 1.44 7 = 1.44 × 8 = 11.52 years.

Question 3.
A radioactive sample contains 2.2 mg of pure 116C which has a half-life period of 1224 seconds. Calculate (i) the number of atoms present initially and (ii) the activity when 5 pg of the sample will be left.
Answer:
Mass of sample = 2.2 pg
Now 11 g of the sample contains 6.023 × 10²³ nuclei, therefore the number of nuclei in 2.2 mg = 2.2 × 10^-3 g are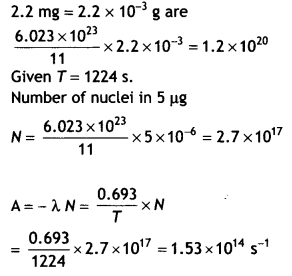

Question 4.
The half-life of 238 92U is 4.5 × 10⁹ years. Calculate the activity of 1 g sample of ₉₂²³⁸U.
Answer:
Given T = 4.5 × 10⁹ years.
Number of nuclei of U in 1 g
= N = \(\frac{6.023 \times 10^{23}}{238}\) = 2.5 × 10²¹

Therefore activity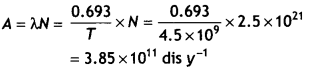

Question 5.
The decay constant for a given radioactive sample is 0.3456 per day. What percentage of this sample will get decayed in a period of 4 days?
Answer:
Given λ = 0.3456 day^-1
or
T_1/2 = 0.693/λ = 0. 693/ 0.3456 = 2.894 days, t = 4 days.

Let N be the mass left behind, then N = N_oe^-λt
or
N = N_o e^{-0 3456 × 4}
or
N = N₀ e^{-1 3824} = N_o × 0.25

Therefore the percentage of undecayed is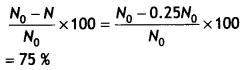

Question 6.
It is observed that only 6.25 % of a given radioactive sample is left undecayed after a period of 16 days. What is the decay constant of this sample per day?
Answer:
Given N/N_o = 6.25 %, t = 16 days, λ = ?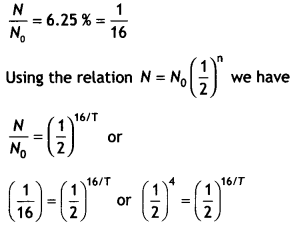

Or
16/ T = 4 or T = 4 days.

Therefore λ = 1/T = 1/4 = 0.25 day^-1

Question 7.
A radioactive substance decays to 1/32^th of its initial value in 25 days. Calculate its half-life.
Answer:
Given t = 25 days, N = N_o / 32, using

Or
25/7= 5 or T= 25 / 5 = 5 days.

Question 8.
The half-life of a radioactive sample is 30 s.
Calculate
(i) the decay constant, and
Answer:
Given T₁/2 = 30 s, N = 3N_o / 4, λ = ?, t = ?
(i) Decay constant
λ = \(\frac{0.693}{T_{1 / 2}}=\frac{0.693}{30}\) = 0.0231 s^-1

(ii) time taken for the sample to decay to 3/4 th of its initial value.
Answer:
Using N = N_oe^-λt we have

Question 9.
The half-life of 14 6C is 5700 years. What does it mean?
Two radioactive nuclei X and Y initially contain an equal number of atoms. Their half-lives are 1 hour and 2 hours respectively. Calculate the ratio of their rates of disintegration after 2 hours.
Answer:
It means that in 5700 years the number of nuclei of carbon decay to half their original value.
Given N_ox = N_oY, T_X = 1 h, T_Y = 2 h, therefore
\(\frac{\lambda_{X}}{\lambda_{Y}}=\frac{2}{1}\) = 2

Now after 2 hours X will reduce to one- fourth and Y will reduce to half their original value.
If activities at t = 2 h are R_x and R_y respectively, then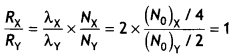

Thus their rate of disintegration after 2 hours is the same.

Question 10.
A star converts all its hydrogen to helium achieving 100% helium composition. It then converts helium to carbon via the reaction.

The mass of the star is 5 × 10³² kg and it generates energy at the rate of 5 × 10³⁰ watt. How long will it take to convert all the helium to carbon at this rate?

As 4 × 10^-3 kg of He consists of 6.023 × 10²³ He nuclei so 5 × 10³² kg He will contain
\(\frac{6.023 \times 10^{23} \times 5 \times 10^{32}}{4 \times 10^{-3}}\) = 7.5 × 10⁵⁸ nuclei

Now three nuclei of helium produce 7.27 × 1.6 × 10^-13 J of energy
So all nuclei in the star will produce
E = \(\frac{7.27 \times 1.6 \times 10^{-13}}{3}\) × 7.5 × 10⁵⁸
= 2.9 × 10⁴⁶ J

As power generated is P = 5 × 10³⁰ W, therefore time taken to convert all He nuclei into carbon is
t = \(\frac{E}{P}=\frac{2.9 \times 10^{46}}{5 \times 10^{30}}\) = 5.84 × 10¹⁵ s
or
1.85 × 10⁸ years

Question 11.
Radioactive material is reduced to (1/16)^th of its original amount in 4 days. How much material should one begin with so that 4 × 10^-3 kg of the material is left after 6 days?
Answer:
N = N_o / 16, t = 4 days,
N = 4 × 10^-3 kg,
t = 6 days

To calculate half-life of the material we have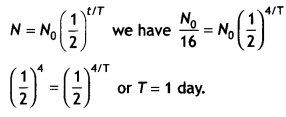

Now using the expression 4 × 10^-3 = No\(\left(\frac{1}{2}\right)^{6 / 1}\)
Solving we have N_o = 0.256 kg

Question 12.
Two different radioactive elements with half-lives T₁ and T₂ have N₁ and N₂ (undecayed) atoms respectively present at a given instant. Determine the ratio of their activities at this instant.
Answer:
The activity of a radioactive sample is given by the relation
A = – λN

Therefore the ratio of activity of these two radioactive elements is
\(\frac{A_{1}}{A_{2}}=\frac{-\lambda_{1} N_{1}}{-\lambda_{2} N_{2}}=\frac{T_{2} N_{1}}{T_{1} N_{2}}\)

Question 13.
Given the mass of the iron nucleus as 55.85 u and A = 56. Find the nuclear density? (NCERT)
Answer:
Given m_Fe = 55.85 u = 9.27 × 10^-26kg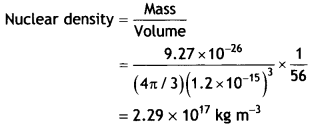

The density of matter in neutron stars (an astrophysical object) is comparable to this density. This shows that matter in these Neutron stars has been compressed to such an extent that they resemble a big nucleus.

Question 14.
We are given the following atomic masses: ₉₂²³⁸U = 238.05079 u, ₂⁴He = 4.00260 u, ₉₀²³⁴Th = 234.04363 u ₁¹H = 1.00783 u, ₉₁²³⁷Pa =237.05121 u Here the symbol Pa is for the element protactinium (Z = 91). (a) Calculate the energy released during the alpha decay of ₉₂²³⁸U. (b) Show that cannot spontaneously emit a proton. (NCERT)
Answer:
(i) The alpha decay of ₉₂²³⁸Uis given by

The energy released in this process is given by
Q= (M_u – M_Th – M_He) × 931.5 MeV

Substituting the atomic masses as given in the data we find that
Q = (238.05079 – 234.04363 – 4.00260) × 931.5 MeV ⇒ Q = 4.25 MeV.

(ii) If 29®U spontaneously emits a proton, the decay process would be

The Q for this process to happen is Q = (M_u – M_pa – M_H) × 931.5 MeV
Q = (238.05079 – 237.05121 – 1.00783) × 931.5 MeV ⇒ Q = – 7.68 MeV

Thus the Q of the process is negative and therefore it cannot proceed spontaneously. We will have to supply energy of 7.68 MeV to the ₉₂²³⁸U nucleus to make it emit a proton.

Question 15.
The half-life of 90Sr is 28 years. What is the disintegration rate of 15 mg of this isotope? (NCERT)
Answer:
Given T_1/2 = 28 years, m = 15 mg
Now the rate of disintegration is given by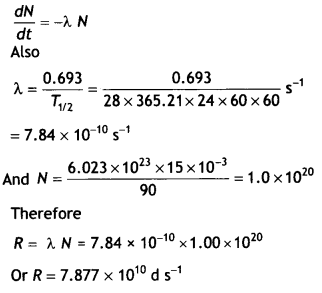

Question 59.
(a) Define the term ‘activity’ of a given sample of radionuclide. Write the expression for the law of radioactive decay in terms of the activity of a given sample.
(b) A radioactive isotope has a half life of T years. How long will it take the activity to reduce to 3.125% of its original value?
(c) When a nucleus (X) undergoes β-decay, and transforms to the nucleus (Y), does the pair (X, Y) form isotopes, isobars or isotones? Justify your answer. (Comptt. Delhi 2012)
Answer:
(a) The activity of a radioactive source is measured by the rate of disintegration of the source. It is denoted by ‘A’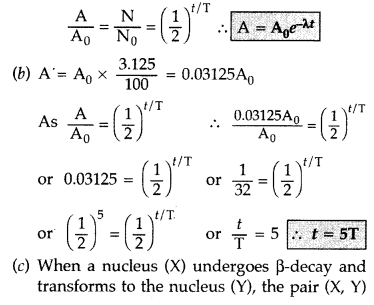

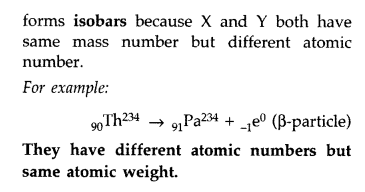
Question 60.
(a) Draw the plot of binding energy per nucleon (BE/A) as a function of mass number A. Write two important conclusions that can be drawn regarding the nature of nuclear force.
(b) Use this graph to explain the release of energy in both the processes of nuclear fusion and fission.
(c) Write the basic nuclear process of neutron undergoing β-decay. Why is the detection of neutrinos found very difficult?
Answer: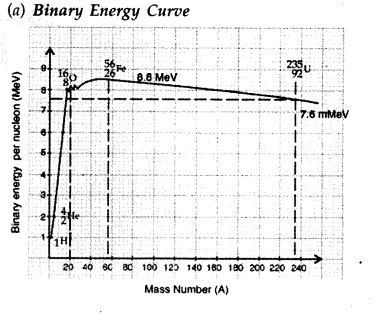

Conclusions :
(i) The force is attractive and sufficiently strong to produce a binding energy of a few MeV per nucleon.

(b) (i) When we move from heavy nuclei region to middle region, we find that there will be a gain in overall binding energy and hence release of energy. This indicates that energy can be released when a heavy nucleus breaks into two roughly equal fragments/nuclear fission.
(ii) Similarly, when we move from lighter nuclei to heavier nuclei, we find that there will be gain in overall binding energy and hence release of energy. This indicates that energy can be released when two lighter
nuclei fuse together to form heavy nucleus/nuclear fusion.
(c) In Beta decay a neutron breaks into a proton, electron and neutrino as

Detection of neutrinos is difficult because they are chargeless and have either no or low mass.