Dual Nature of Radiation and Matter

Class 12 Physics Chapter 11 Important Extra Questions Dual Nature of Radiation and Matter

Very Short Answer

Question 1.
An electron and alpha particle have the same de-Broglie wavelength associated with them. How are their kinetic energies related to each other? (Delhi 2008)
Answer: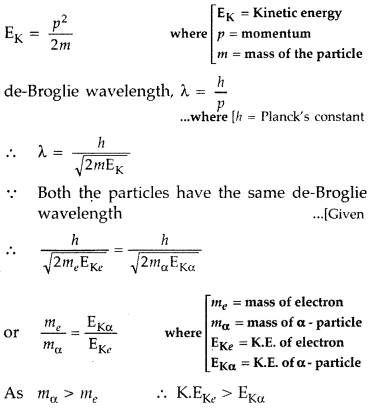

Question 2.
Two lines, A and B, in the plot given below show the variation of de-Broglie wavelength, λ versus \(\frac{1}{\sqrt{\mathbf{V}}}\), Where V is the accelerating potential difference, for two particles carrying the same charge. Which one of two represents a particle of smaller mass ? (All India 2008)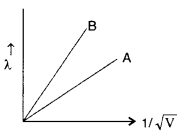

Answer:

Question 3.
The figure shows a plot of three curves a, b, c, showing the variation of photocurrent vs. collector plate potential for three different intensities I₁, I₂ and I₃ having frequencies V₁, v₂ and v₃ respectively incident on a photosensitive surface.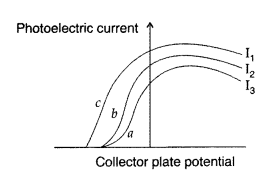

Point out the two curves for which the incident radiations have same frequency but different intensities.
Answer:
Stopping potential will be same for the same frequency. So its curves ‘a’ and ‘b’ which have same frequency but different intensities. (I₂ > I₃)

Question 4.
The stopping potential in an experiment on photoelectric effect is 1.5 V. What is the maximum kinetic energy of the photoelectrons emitted? (All India 2008)
Answer:
K.E. of the electron e^– = 1.5 eV

Question 5.
The maximum kinetic energy of a photoelectron is 3 eV. What is its stopping potential? (All India 2008)
Answer:

Question 6.
Show graphically, the variation of the de- Broglie wavelength (λ) with the potential (V) through which an electron is accelerated from rest.
Answer: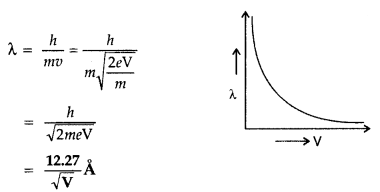

Question 7.
Define the term ‘stopping potential’ in relation to photoelectric effect. (All India 2011)
Answer:
The value of the retarding potential at which the photo electric current becomes zero is called cut off or stopping potential for the given frequency of the incident radiation.

Question 8.
State de-Broglie hypothesis. (Delhi 2011)
Answer:
According to de-Broglie hypothesis, a particle of mass on moving with given velocity v must be associated with a matter waver of wavelength X given by:

Question 9.
A proton and an electron have same kinetic energy. Which one has greater de-Broglie wavelength and why? (All India 2011)
Answer: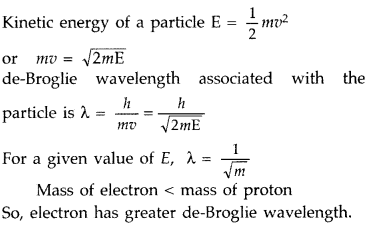

Question 10.
A proton and an electron have same kinetic energy. Which one has smaller de-Broglie wavelength and why? (All India 2011)
Answer:

Question 11.
Define ‘intensity’ of radiation in photon picture of light. (Comptt. Delhi 2011)
Answer:
It is the number of photo electrons emitted per second.

Question 12.
Why is photoelectric emission not possible at all frequencies? (Comptt. All India 2011)
Answer:
Photoelectric emission is possible only if the energy of the incident photon (hv) is greater than the work function (ω₀ = hv₀) of the metal. Hence the frequency v of the incident radiation must be greater than the threshold frequency v₀.

Question 13.
The given graph shows the variation of photo-electric current (I) versus applied voltage (V) for two different photosensitive materials and for two different intensities of the incident radiation. Identify the pairs of curves that correspond to different materials but same intensity of incident radiation. (Delhi 2013)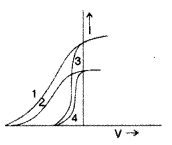

Answer:
The pairs (2, 4) and (1, 3) have same intensity but different material.

Question 14.
Write the expression for the de Broglie wavelength associated with a charged particle having charge ‘q’ and mass ‘m’, when it is accelerated by a potential V. (All India 2013)
Answer:

Question 15.
Show on a plot the nature of variation of photoelectric current with the intensity of radiation incident on a photosensitive surface. (Comptt. Delhi 2013)
Answer: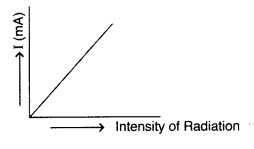

Question 16.
Figure shows a plot of \(\frac{1}{\sqrt{\mathbf{V}}}\), where V is the accelerating potential, vs. the de-Broglie wavelength ‘λ’ in the case of two particles having same charge ‘q’ but different masses m₁ and m₂. Which line (A or B) represents a particle of larger mass? (Comptt. All India 2013)

Answer:
B line represents particle of larger mass because slope \(\propto \frac{1}{\sqrt{m}}\).

Question 17.
Find the ratio of de-Broglie wavelengths associated with two electrons accelerated through 25 V and 36 V. (Comptt. All India 2013)
Answer: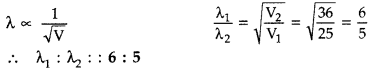

Question 18.
Define intensity of radiation on the basis of photon picture of light. Write its S.I. unit. (All India 2014)
Answer:
It is the number of photo-electrons emitted per second per unit area.
SI unit : m^-2S^-1

Question 19.
The graph shows the variation of stopping potential with frequency of incident radiation for two photosensitive metals A and B. Which one of the two has higher value of work- function? Justify your answer. (All India 2014)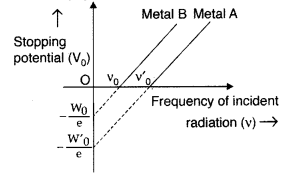

Answer:
Metal ‘A’, because of higher threshold frequency for it.

Question 20.
The graph shows variation of stopping potential V₀ versus frequency of incident radiation v for two photosensitive metals A and B. Which of the two metals has higher threshold frequency and why? (All India 2014)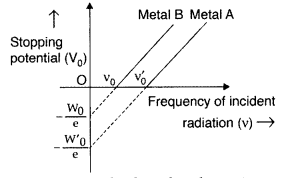

Answer:
Metal ‘A’, because of higher threshold frequency for it.

Question 21.
An electron is revolving around the nucleus with a constant speed of 2.2 × 10⁸ m/s. Find the de-Broglie wavelength associated with it. (Comptt. Delhi 2014)
Answer: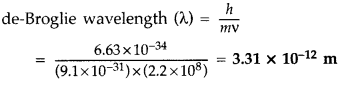

Question 22.
Draw a plot showing the variation of de Broglie wavelength of electron as a function of its K.E.
(Comptt. Delhi 2014)
Answer: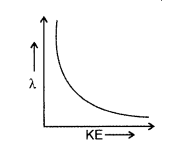

Question 23.
Name the phenomenon which shows the quantum nature of electromagnetic radiation. (Delhi 2014)
Answer:
Photoelectric Effect is the phenomenon which shows the quantum nature of electro-magnetic radiation.

Question 24.
State one factor which determines the intensity of light in the photon picture of light. (Comptt. Delhi 2014)
Answer:
The factor determining the intensity of light is number of electrons emitted per second.

Question 25.
State one reason to explain why wave theory of light does not support photoelectric effect. (Comptt. Delhi 2014)
Answer:
One reason why wave theory of light does not support photoelectric effect is that the kinetic energy of photo electrons does not depend on the intensity of incident light.

Question 26.
If the distance between the source of light and the cathode of a photo cell is doubled, how does it affect the stopping potential applied to the photo cell? (Comptt. Delhi 2014)
Answer:
Stopping potential remains unchanged, if the distance between the light source and cathode is doubled.

Question 27.
If the maximum kinetic energy of electrons emitted by photocell is 4 eV, what is the stopping potential?
Answer:
The stopping potential is 4 V.

Question 28.
Two metals A and B have a work function 4 eV and 10 eV respectively. Which metal has a higher threshold wavelength?
Answer:
The threshold wavelength is inversely proportional to the work function. Therefore metal A has a higher threshold wavelength.

Question 29.
Ultraviolet light is incident on two photo-sensitive materials having work functions W₁ and W₂ (W₁ > W₂). In which case will the kinetic energy of the emitted electrons be greater? Why?
Answer:
The kinetic energy of the emitted photoelectrons is given by 1/2 mv² = hv – W; therefore the lesser the work function for a given frequency, the more is the kinetic energy of the emitted photoelectrons. Since W₂ < W₁, kinetic energy will be more for the metal having work function W₂.

Question 30.
Does the threshold frequency depend on the intensity of light?
Answer:
No, it does not.

Question 30 a.
Name the experiment which establishes the wave nature of a particle.
Answer:
Davison-Germer experiment.

Question 31.
Mention one physical process for the release of electrons from a metal surface.
Answer:
Photoelectric effect.

Question 32.
Name a phenomenon that illustrates the particle nature of light.
Answer:
Photoelectric effect.

Question 33.
Define the work function for a given metallic surface.
Answer:
The minimum amount of energy required to just eject an electron from a given metal surface is called the work function for that metal surface.

Question 34.
What is the value of stopping potential between the cathode and anode of a photocell, if the maximum kinetic energy of the electrons emitted is 5 eV?
Answer:
Stopping potential = 5 V.

Question 35.
Define the term ‘stopping potential’ in relation to the photoelectric effect. (CBSE AI 2011)
Answer:
It is the negative potential of the collector plate for which no photoelectron reaches the collector plate.

Question 36.
Write the relationship of de-Broglie wavelength associated with a particle of mass m in terms of its kinetic energy E. (CBSE Delhi 2011C)
Answer:
The required relation is λ = \(\frac{h}{\sqrt{2 m E}}\)

Question 37.
State de-Broglie hypothesis. (CBSE Delhi 2012)
Answer:
It states that moving particles should possess a wave nature.

Question 38.
Define the term “threshold frequency”, in the context of photoelectric emission. (CBSE Delhi 2019)
Answer:
It is the minimum frequency of incident radiation (light) that can cause photoemission from a given photosensitive surface.
Or
Define the term “Intensity” in the photon picture of electromagnetic radiation. (CBSE Delhi 2019)
Answer:
It is defined as the number of energy quanta (photons) per unit area per unit time.

Question 39.
Write the expression for the de-Broglie wavelength associated with a charged particle having charge ‘q’ and mass ‘m’, when it is accelerated by a potential V. (CBSE AI 2013)
Answer:
The expression is λ = \(\frac{h}{\sqrt{2 m q V}}\)

Question 40.
Define the intensity of radiation on the basis of the photon picture of light. Write its SI unit. (CBSE AI 2014)
Answer:
The intensity of radiation is defined as the number of energy quanta per unit area per unit time. It is measured in W m-2.

Question 41.
Name the phenomenon which shows the quantum nature of electromagnetic radiation. (CBSE Al 2017)
Answer:
Photoelectric effect.

Question 42.
If the distance between the source of light and the cathode of a photocell is doubled how does it affect the stopping potential applied to the photocell? (CBSE Delhi 2017C)
Answer:
No effect.

Question 43.
Draw graphs showing the variation of photoelectric current with applied voltage for two incident radiations of equal frequency and different intensities. Mark the graph for the radiation of higher intensity. (CBSE AI 2018)
Answer:
The graphs are as shown.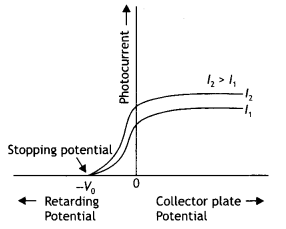

Question 44.
An electron is accelerated through a potential difference V. Write the expression for its final speed if it was initially at rest. (CBSE AI Delhi 2018C)
Answer:
Using eV = \(\frac{1}{2}\)mv² or v = \(\sqrt{\frac{2 e V}{m}}\)

Question 45.
Write the name given to the frequency v0, in the following graph (showing the variation of stopping potential (V_o) with the frequency (v) of the incident radiation) for a given photosensitive material. Also name the constant, for that photosensitive material, obtained by multiplying v_c with Planck’s constant.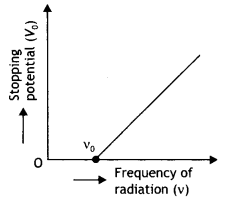

Answer:
The constant obtained by multiplying v0 with Planck’s constant is called the work function of the material. This frequency is called the threshold frequency.

Question 46.
Electrons are emitted from a photosensitive surface when it is illuminated by green light but electron emission does not take place by yellow light. Will the electrons are emitted when the surface is illuminated by
(a) red light
Answer:
No electron will be emitted when illuminated by a red light.

(b) blue light?
Answer:
Electron emission takes place with blue light.

Question 47.
The frequency (v) of incident radiation is greater than the threshold frequency v0 in a photocell. How will the stopping potential vary if frequency (v) is increased keeping other factors constant?
Answer:
On increasing the frequency v of the incident light, the value of stopping potential also increases.

Question 48.
How much time is taken by a photoelectron to come out of a metal surface, when the light of wavelength less than threshold wavelength λ_o is incident on it?
Answer:
It is an instantaneous process. The time required is of the order of a nanosecond (10^-9 s).

Question 49.
The given graphs show the variation of photoelectric current (l) with the applied voltage (V) for two different materials and for two different intensities of the incident radiations. Identify the pairs of curves that correspond to different materials but the same intensity of incident radiations.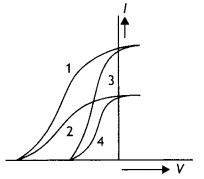

Answer:
Pairs 1 – 2 and pairs 3 – 4

Question 50.
Red light, however bright, cannot cause the emission of electrons from a clean zinc surface. But even weak ultraviolet radiations can do so. Why?
Answer:
This is because the threshold frequency of the given metal is greater than the frequency of red light.

Question 51.
If the intensity of incident radiation on metal is doubled, what happens to the kinetic energy of electrons emitted?
Answer:
The kinetic energy of emitted electrons remains unchanged.

Question 52.
If the intensity of the incident radiation in a photocell is increased, how does the stopping potential vary?
Answer:
Stopping potential remains unaffected.

Question 53.
The work function of aluminium is 4.2 eV. If two photons each of energy 2.5 eV are incident on a surface, will the emission of photoelectron take place?
Answer:
No, emission of photoelectron will not take place.

Question 54.
A photon and an electron have the same de-Broglie wavelength. Which is moving faster?
Answer:
Photon is moving faster with a speed c = 3 × 10⁸ ms^-1. An electron must have a velocity less than the speed of light.

Question 55.
Does the ‘stopping potential’ in photoelectric emission depend upon
(i) the intensity of the incident radiation in a photocell
Answer:
No

(ii) the frequency of the incident radiation?
Answer:
Yes.

Question 56.
The de-Broglie wavelengths, associated with a proton and a neutron, are found to be equal. Which of the two has a higher value for kinetic energy?
Answer:
Proton.

Question 57.
The figure shows a plot of \(\frac{1}{\sqrt{V}}\) where V is the accelerating potential vs the de-Broglie wavelength λ in case of two particles having the same charge q but different masses m₁ and m₂. Which line A or B represents the particle of greater mass? (CBSE AI 2013C)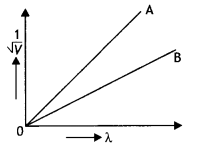

Answer:
Particle A

Question 58.
Do X-rays exhibit the phenomenon of the photoelectric effect?
Answer:
Yes, they do exhibit the phenomenon of the photoelectric effect.

Question 59.
Draw a graph showing the variation of de-Broglie wavelength with the momentum of an electron. (CBSE AI 2019)
Answer:
The graph is shown below.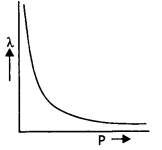

Question 60.
The work function of two metals A and B are 2 eV and 5 eV respectively. Which of these is suitable for a photoelectric cell using visible tight?
Answer:
Metal A having a lower work function of 2 eV is suitable for use with visible light.

Question 61.
Estimate the frequency associated with a Photon of energy 2 eV. (CBSE Delhi 2019C)
Answer:
Since E = hv

Question 62.
For a given photosensitive material and with a source of the constant frequency of incident radiation, how does the photocurrent vary with the intensity of incident light? (CBSE AI 2011C)
Answer:
The photoelectric current increases linearly with the intensity of light.

Question 63.
Draw a graph showing the variation of the de-Broglie wavelength of an electron as a function of its kinetic energy. (CBSE Delhi 2015C)
Answer:
The graph is as shown.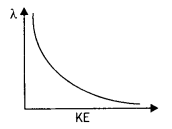

Question 64.
(a) In the explanation of the photoelectric effect, we assume one photon of frequency v collides with an electron and transfers its energy. This leads to the equation for the maximum energy E_max of the emitted electron as E_max = hv – Φ_o where Φ_o is the work function of the metal. If an electron absorbs 2 photons (each of frequency v), what will be the maximum energy for the emitted electron?
Answer:
Emax = 2hv – Φ

(b) Why is this fact (two photons absorption) not taken into consideration in our discussion of the stopping potential? (NCERT Exemplar)
Answer:
The probability of absorbing 2 photons by the same electron is very low. Hence ’ such emissions will be negligible.

Question 65.
Do all the electrons that absorb a photon comes out as photoelectrons? (NCERT Exemplar)
Answer:
No, most electrons get scattered into the metal. Only a few come out of the surface of the metal.

Short Answer Type

Question 1.
An electron is accelerated through a potential difference of 100 volts. What is the de-Broglie wavelength associated with it? To which part of the electromagnetic spectrum does this value of wavelength correspond? (Delhi 2010)
Answer:
Given : V = 100 V
According to de-Broglie ivavelength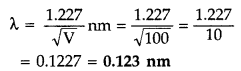

The value of de-Broglie wavelength is 0.123 nm which corresponds to the wavelength of X-rays region of the electromagnetic spectrum.

Question 2.
An electron is accelerated through a potential difference of 64 volts. What is the de-Broglie wavelength associated with it? To which part of the electromagnetic spectrum does this value of wavelength correspond? (Delhi 2010)
Answer:
According to de-Broglie wavelength,

This wavelength is associated with X-rays.

Question 3.
An a-particle and a proton are accelerated from rest by the same potential. Find the ratio of their de-Broglie wavelengths. (All India 2010)
Answer:
de-Broglie wavelength of a charged (q)
Particle accelerated through a potential ‘V’ is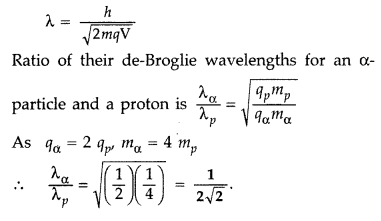

Question 4.
Write Einstein’s photoelectric equation. State clearly the three salient features observed in photoelectric effect, which can be explained on the basis of the above equation. (All India 2010)
Answer:
Einstein’s photoelectric equation is K_max = hv – ϕ₀
(i) We find K_max depends linearly on V only. It is independent of intensity of radiation.

(iii) Greater the number of energy quanta, greater is the number of photoelectrons. So, photoelectric current is proportional to intensity.

Question 5.
Plot a graph showing the variation of stopping potential with the frequency of incident radiation for two different photosensitive materials having work functions W₁ and W₂ (W₁ > W₂). On what factors does the
(i) slope and
(ii) intercept of the lines depend? (Delhi 2010)
Answer: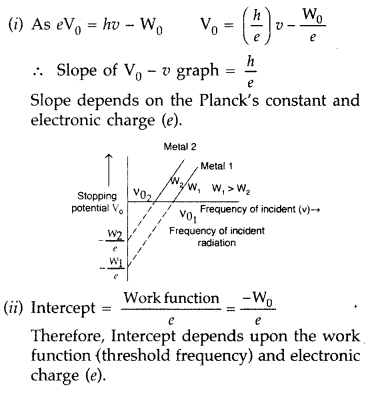

Question 6.
A proton and a deuteron are accelerated through the same accelerating potential. Which one of the two has
(a) greater value of de-Broglie wavelength associated with it, and
(b) less momentum?
Give reasons to justify your answer. (Delhi 2010)
Answer:
For proton and deuteron, charge (q) is the same, while the mass of deuteron is more than that of proton

Here q and V are the same for both,

∴ Proton will be associated with greater value of de-Broglie wavelength.

Question 7.
A proton and an alpha particle are accelerated through the same potential. Which one of the two has
(i) greater value of de-Broglie wavelength associated with it, and
(ii) less kinetic energy.
Give reasons to justify your answer. (Delhi 2010)
Answer:
Similar to Q. 32, Page 255
[Hint. Proton’s mass is less than that of alpha particle, which contains 2 protons and 2 neutrons.]

Question 8.
A deuteron and an alpha particle are accelerated with the same accelerating potential. Which one of the two has
(1) greater value of de-Broglie wavelength, associated with it, and
(2) less kinetic energy? Explain. (Delhi 2010)
Answer:
Similar to Q. 32, Page 255
[Hint. A deuteron (consisting of one proton and one neutron) has less mass than alpha particle (consisting of 2 protons and 2 neutrons)]

Question 9.
(i) Monochromatic light of frequency 6.0 × 10¹⁴ Hz is produced by a laser. The power emitted is 2.0 × 10^-3 W. Estimate the number of photons emitted per second on an average by the source.
(ii) Draw a plot showing the variation of photoelectric current versus the intensity of incident radiation on a given photosensitive surface. (Delhi 2010)
Answer:

(ii)

Question 10.
Two monochromatic radiations of frequencies v¹ and v² (V¹ > v²) and having the same intensity are, in turn, incident on a photosensitive surface to cause photoelectric emission. Explain, giving reason, in which case
(i) more number of electrons will be emitted and
(ii) maximum kinetic energy of the emitted photoelectrons will be more. (Comptt. Delhi 2010)
Answer:

Question 11.
X-rays fall on a photosensitive surface to cause photoelectric emission. Assuming that the work function of the surface can be neglected, find the relation between the de-Broglie wavelength (λ) of the electrons emitted to the energy (E₀) of the incident photons. Draw the nature of the graph for X as a function of E_v. (Comptt. Delhi 2010)
Answer: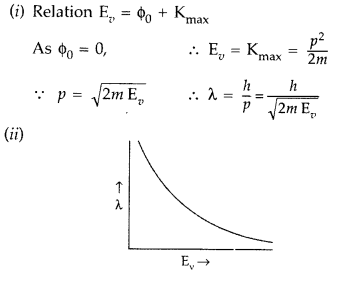

Question 12.
Write three basic properties of photons which are used to obtain Einstein’s photoelectric equation. Use this equation to draw a plot of maximum kinetic energy of the electrons emitted versus the frequency of incident radiation. (Comptt. All India 2010)
Answer:
Properties.
Einstein’s photoelectric equation is K_max = hv – ϕ₀
(i) We find K_max depends linearly on V only. It is independent of intensity of radiation.

(iii) Greater the number of energy quanta, greater is the number of photoelectrons. So, photoelectric current is proportional to intensity.

Plot of maximum kinetic energy vs. frequency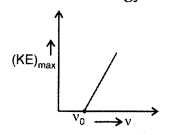

Question 13.
(i) Define the term ‘threshold frequency’ as used in photoelectric effect.
(ii) Plot a graph showing the variation of photoelectric current as a function of anode potential for two light beams having the same frequency but different intensities I₁ and I₂ (I₁ > I₂). (Comptt. All India 2010)
Answer:
(i) Threshold frequency. The minimum frequency v₀ which the incident light must possess so as to eject photoelectrons from a metal surface, is called threshold frequency of the metal.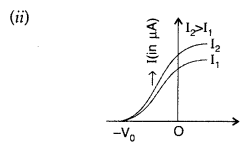

Question 14.
A proton and an a-particle have the same de- roglie wavelength. Determine the ratio of
(i) their accelerating potentials
(ii) their speeds. (Delhi 2015)
Answer:
The de-Broglie wavelength for a proton is,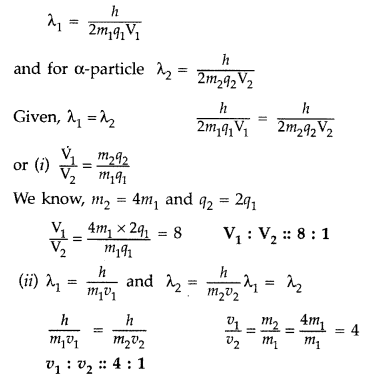

Question 15.
Using the graph shown in the figure for stopping potential v/s the incident frequency of photons, calculate Planck’s constant. (Comptt. Delhi 2015)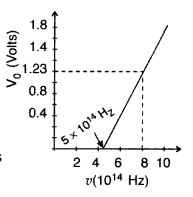

Answer: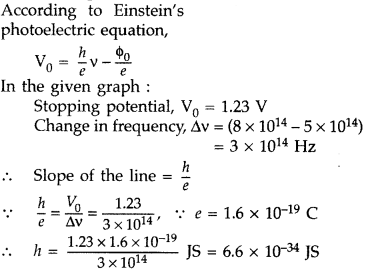

Question 16.
Plot a graph showing variation of de-Broglie wavelength λ versus \(\frac{1}{\sqrt{\mathbf{V}}}\), where V is accelerating potential for two particles A and B carrying same charge but of masses m₁, m₂ (m₁ > m₂). Which one of the two represents a particle of smaller mass and why?
Answer:

Hence, particle with lower mass (m₂) will have greater slope and is represented by the graph ‘B’.

Question 17.
Calculate the de-Broglie wavelength of the electron orbitting in the n = 2 state of hydrogen atom. (All India 2015)
Answer:
Given : n = 2 of hydrogen atom X = ?
Kinetic energy for the second state,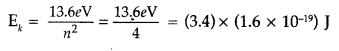

Question 18.
The work function (W), of a metal X, equals 3 × 10^-19 J. Calculate the number (N) of photons, of light of wavelength 26.52 nm, whose total energy equals W. (Comptt. Delhi 2015)
Answer: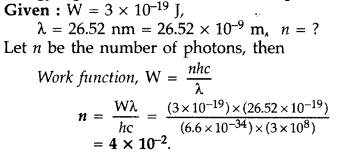

Question 19.
The Kinetic Energy (K.E.), of a beam of electrons, accelerated through a potential V, equals the energy of a photon of wavelength 5460 nm. Find the de Broglie wavelength associated with this beam of electrons. (Comptt. All India 2015)
Answer: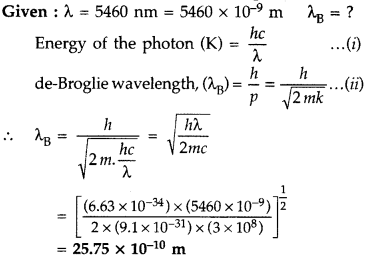

Question 20.
An a-particle and a proton are accelerated through the same potential difference. Find the ratio of their de Broglie wavelengths. (Delhi 2015)
Answer:
From de Broglie equation, we know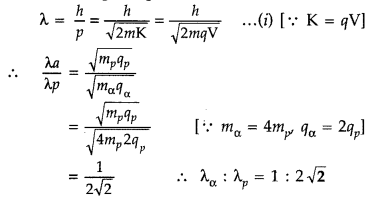

Question 21.
Electrons are emitted from the cathode of a photocell of negligible work function, when photons of wavelength are incident on it. Derive the expression for the de Broglie wavelength of the electrons emitted in terms of the wavelength of the incident light. (Comptt. All India 2015)
Answer:

Question 22.
Derive the Bohr’s quantisation condition for angular momentum of the orbitting of electron in hydrogen atom, using de Broglie’s hypothesis. (Comptt. All India 2015)
Answer: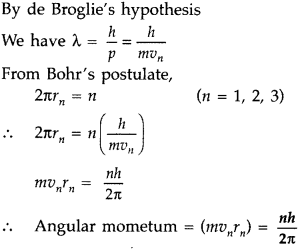

Question 23.
Calculate the kinetic energy of an electron having de Broglie wavelength of 1Å. (Comptt. All India 2015)
Answer: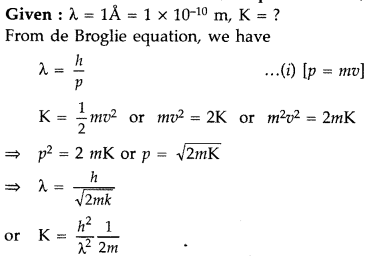

Question 24.
State two properties of photons. For a monochromatic radiation incident on a photosensitive surface, why do all photoelectrons not come out with the same energy? Give reason for your answer.
(Comptt. All India 2017)
Answer:

• Two properties of photons :
  • photon is electrically neutral.
  • photon has an energy equal to hv
• For a monochromatic radiation incident on a photosensitive surface, all photoelectrons do not come out with the same energy, because in addition to the work done to free electrons from the surface, different (emitted) photoelectrons need different amount of work to be done on them to reach the surface.

Question 25.
A photon and a proton have the same de-Broglie wavelength. Show, by actual calculations, which has more total energy.
Answer: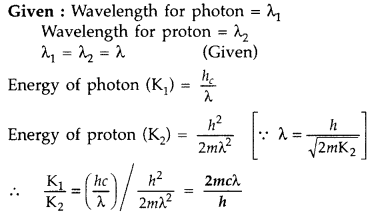

Question 26.
An a-particle and a proton of the same kinetic energy are in turn allowed to pass through a magnetic field B, acting normal to the direction of motion of the particles. Calculate the ratio of radii of the circular paths described by them. (CBSE Delhi 2019)
Answer:
Given q_α = 2e, q_p = e, K_α = K_p, mα = 4m_p, r_α/r_p = ?
Using the expression
r = \(\frac{\sqrt{2 m K}}{q B}\) we have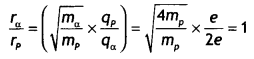

Question 27.
How will the photoelectric current change on decreasing the wavelength of incident radiation for a given photosensitive material?
Answer:
Photoelectric current is independent of the wavelength of the incident radiation. Therefore there will be no change in the photoelectric current.

Question 28.
Estimate the ratio of the wavelengths associated with the electron orbiting around the nucleus in the ground and first excited states of a hydrogen atom. (CBSE Delhi 2019C)
Answer:
Since De Brogue’s hypothesis is related to
Bohr’s atomic model as

Question 29.
Show graphically how the stopping potential for a given photosensitive surface varies with the frequency of the incident radiation.
Answer:
The required graph is as shown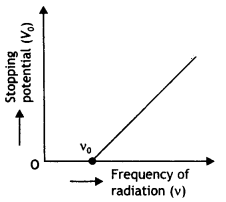

Question 30.
the de-Broglie wavelength associated with an electron accelerated through a potential difference V is λ. What will be its wavelength when accelerating potential is increased to 4 V?
Answer:
The de-BrogLie wavelength is inversely proportional to the square root of potential, therefore = \(\frac{\lambda_{2}}{\lambda_{1}}=\frac{\sqrt{V}}{\sqrt{4 V}}=\frac{1}{2}\) . Thus wavelength
wilt become half of its previous value.

Question 31.
Plot a graph showing the variation of de Brogue wavelength (λ) associated with a charged particle of mass m, versus \(\frac{1}{\sqrt{V}}\) where V is the potential difference through which the particle is accelerated. How does this graph give us information regarding the magnitude of the charge of the particle? (CBSE Dethi 2019)
Answer:
The plot is as shown.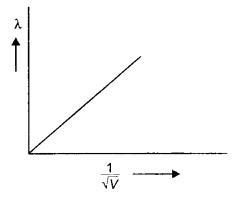


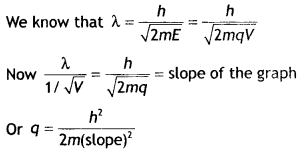
Question 32.
X-rays of wavelength ‘λ’ fall on a photosensitive surface, emitting electrons. Assuming that the work function of the surface can be neglected, prove that the de-Broghe wavelength of the electrons emitted will be \(\sqrt{\frac{h \lambda}{2 m c}}\)
Answer:
The energy possessed by X-rays of wavelength λ is given by E=hc / λ.
Consider an electron of mass charge e to be accelerated the potential difference of V volts the velocity gained by it.

Then kinetic energy of electron is
E = \(\frac{1}{2} m v^{2}\) = eV
or
v = \(\sqrt{\frac{2 \mathrm{eV}}{\mathrm{m}}}=\sqrt{\frac{2 E}{m}}\)

If λ is the de-Broglie wavelength associated with an electron, then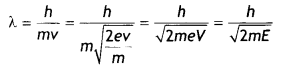

Substituting for e, we have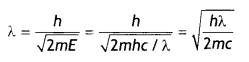

Question 33.
Explain with the help of Einstein’s photoelectric equation any two observed features in the photoelectric effect. cannot be explained by the wave theory. (CBSE Delhi 2019)
Answer:
According to Einstein’s equation, we have
\(\frac{1}{2} m v_{\max }^{2}\) = h(v – vo)

Two features
(a) Maximum energy is directly proportional to the frequency
(b) Existence of threshold frequency Explanation of two features:

1. The energy of the photon is directly proportional to the frequency
2. No photoelectric emission is possible if hv < hv_o

Question 34.
Why is the wave theory of electromagnetic radiation not able to explain the photoelectric effect? How does the photon picture resolve this problem? (CBSE Delhi 2019)
Answer:
According to the wave theory, the more intense a beam, more is the kinetic energy it will impart to the photoelectron. This does not agree with the experimental observations (max K.E. of the emitted photoelectron is independent of intensity) on the photoelectric effect. Also according to the wave theory photoemission can occur at all frequencies.

The photon picture resolves this problem by saying that light in interaction with matter behaves as if it is made of quanta or packets of energy, each of energy hv. This picture enables us to get a correct explanation of all the observed experimental features of the photoelectric effect.

Question 35.
(a) Define the terms,
(i) threshold frequency and
(ii) stopping potential in the photoelectric effect.
(b) Plot a graph of photocurrent versus anode potential for radiation of frequency v and intensities l₁ and l₂. (l₁ < l₂). (CBSE Delhi 2019)
Answer:
(a) Threshold frequency: It is the frequency of the incident radiation below which photoelectric effect does not take place.
Stopping potential: It is the minimum negative (retarding) potential, given to the anode (collector plate) for which the photocurrent stops or becomes zero.

(b) The plot is as shown.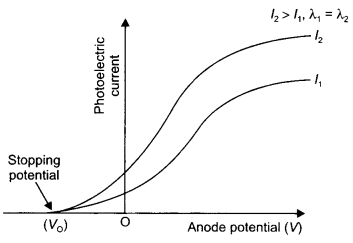

Question 36.
A proton and a particle are accelerated through the same potential difference. Which one of the two has
(i) greater de-Broglie wavelength, and
(ii) less kinetic energy? Justify your answer. (CBSE AI 2016)
Answer:
(i) We know that λ = \(\frac{h}{\sqrt{2 m q V}}\), therefore
we have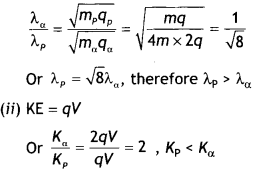

Question 37.
Plot a graph showing the variation of de-Broglie wavelength λ versus \(\frac{1}{\sqrt{V}}\) where V is the accelerating potential for two particles A and B carrying the same charge but masses m1 m2 (m1 > m2). Which one of the two represents a particle of smaller mass and why? (CBSE Delhi 2016)
Answer:
The graph is as shown.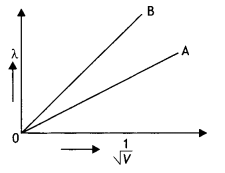

we know that λ = \(\frac{h}{\sqrt{2 m q V}}\)

In graphs, charge of both particles is the same, but the slope of graph B is more. It means that mass of particle B is less (since slope ∝ \(\frac{1}{\sqrt{m}}\)

Question 38.
For a photosensitive surface, the threshold wavelength is λo. Does photoemission occur if the wavelength λ of the incident radiation is
(i) more than λ_o and
(ii) less than λ_o? Justify your answer.
Answer:
The photoelectric effect occurs and hence photoelectrons are ejected when the wavelength of the incident radiation is lesser than the threshold wavelength.
(i) When λ > λ_o, photoemission does not take place.
(ii) When λ < λ_o, photoemission takes place.

Question 39.
Two monochromatic radiations of frequencies v₁ and v₂ (v₁ > v₂) and having the same intensity is, in turn, an incident on a photosensitive surface to cause photoelectric emission. Explain, giving a reason, in which case (i) more number of electrons will be emitted and (ii) maximum kinetic energy of the emitted photoelectrons will be more. (CBSE Delhi 2014C)
Answer:
The number of photoelectrons emitted depends upon the intensity of radiation and the kinetic energy of photoelectrons depends upon the frequency of radiation, therefore

1. The same number of electrons will be emitted.
2. Photoelectrons will have more kinetic energy for radiation of frequency v₁.

Question 40.
A proton and an a-particle are accelerated, using the same potential difference. How are the de-Broglie wavelengths λP and λα related to each other? (NCERT Exemplar)
Answer:

Question 41.
There are materials that absorb photons of shorter wavelength and emit photons of longer wavelength. Can there be stable substances that absorb photons of larger wavelength and emit light of shorter wavelength? (NCERT Exemplar)
Answer:
In the first case energy given out is less than the energy supplied. In the second case, the material has to supply energy as the emitted photon has more energy. This cannot happen for stable substances.

Question 42.
Two monochromatic beams A and B of equal intensity l hit a screen. The number of photons hitting the screen by beam A is twice that by beam B. Then what inference can you make about their frequencies? (NCERT Exemplar)
Answer:

The frequency of beam B is twice that of beam A.

Question 43.
An electromagnetic wave of wavelength X is incident on a photosensitive surface of negligible work function. If the photoelectrons emitted from this surface have the de-Broglie wavelength λ₁, prove that \(\lambda=\left(\frac{2 m c}{h}\right) \lambda_{1}^{2}\) .(Delhi 2008)
Answer:

Question 44.
The following graph shows the variation of stopping potential V₀ with the frequency v of the incident radiation for two photosensitive metals X and Y :

(i) Which of the metals has larger threshold wavelength? Give reason.
(ii) Explain, giving reason, which metal gives out electrons, having larger kinetic energy, for the same wavelength of the incident radiation.
(iii) If the distance between the light source and metal X is halved, how will the kinetic energy of electrons emitted from it change? Give reason. (All India 2008)
Answer: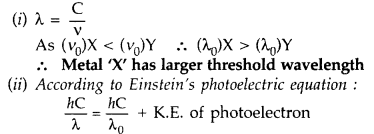

For the same λ of incident radiation, L.H.S. is constant. So metal X with higher value of λ₀ will emit photoelectrons of larger K.E.
(iii) Kinetic energy will not change. On reducing the distance only intensity of light changes, frequency remains same. K.E. of emitted photoelectrons depends on frequency.

Question 45.
A proton and an alpha particle are accelerated through the same potential. Which one of the two has
(i) greater value of de-Broglie wavelength associated with it, and
(ii) less kinetic energy? Justify your answers. (Delhi 2008)
Answer: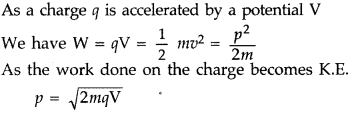


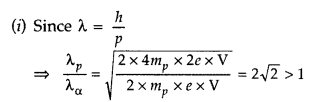
∴ Proton will have greater de-Broglie wavelength
(ii) Energy E = qV. So one proton having lesser charge in coulomb will have less K.E.

Question 46.
An electron and a proton are accelerated through the same potential. Which one of the two has
(i) greater value of de-Broglie wavelength associated with it and
(ii) less momentum? Justify your answer. (Delhi 2008)
Answer:

Question 47.
An electron and a photon each have a wavelength of 1.50 nm. Find
(i) their momenta,
(ii) the energy of the photon and
(iii) kinetic energy of the electron. (Delhi 2011)
Answer: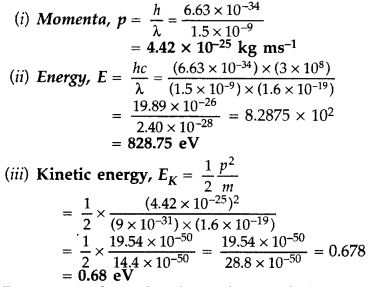

Question 48.
Draw a plot showing the variation of photoelectric current with collector plate potential for two different frequencies, v₁ > v₂, of incident radiation having the same intensity. In which case will the stopping potential be higher? Justify your answer. (All India 2011)
Answer:
Stopping potential is directly proportional to the frequency of incident radiation. The stopping potential is more negative for higher frequencies of incident radiation. Therefore, stopping potential is higher in v₁.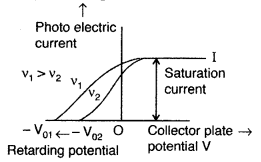

Question 49.
(a) Using de-Broglie’s hypothesis, explain with the help of a suitable diagram, Bohr’s second postulate of quantization of energy levels in a hydrogen atom.
(b) The ground state energy of hydrogen atom is -13.6 eV. What are the kinetic and potential energies of the state?
Answer: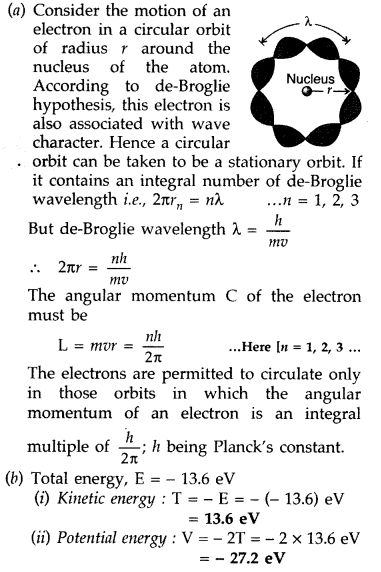

Question 50.
Write Einsten’s photoelectric equation. State clearly how this equation is obtained using the photon picture of electromagnetic radiation. Write the three salient features observed in photoelectric effect which can be explained using this equation. (Delhi 2011)
Answer:

This is Einstein’s photoelectric equation. Photoelectric emission is the result of interaction of two particles—one a photon of incident radiation and other an electron of photo sensitive metal. The free electrons are bound within the metal due to restraining forces on the surface. The minimum energy required to liberate an electron from the metal surface is called work function ϕ₀ of the metal. Each photon interacts with one electron. The energy hv of the incident photon is used up in two parts:
(a) a part of the energy of the photon is used in liberating the electron from the metal surface, which is equal to the work function ϕ₀ of the metal and
(b) the remaining energy of the photon is used in imparting K.E. of the ejected electron.
By the conservation of energy Energy of the inefficient photon = maximum K.E. of photoelectron + Work function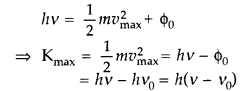

Three salient features are :
Three salient features observed in photoelectric effect on the basis of Einstein’s Photoelectric equation :

Question 51.
Define the terms
(i) ‘cut-off voltage’ and
(ii) ‘threshold frequency’ in relation to the pheno-menon of photoelectric effect.
Using Einstein’s photoelectric equation show how the cut-off voltage and threshold frequency for a
given photosensitive material can be determined with the help of a suitable plot/graph. (All India 2011) Answer:
(i) Cut-off voltage : The value of the retarding potential at which the photo electric current becomes zero is called cut-off or stopping potential for the given frequency of the incident radiation.
(ii) Threshold frequency : The minimum value of the frequency of incident radiation below which the photoelectric emission stops altogether is called threshold frequency.
According to Eisntein’s photo electric equation,

Question 52.
Draw a graph showing the variation of stopping potential with frequency of incident radiation for two photosensitive materials having work functions W₁ and W₂ (W₁ > W₂).
Write two important conclusions that can be drawn from the study of these plots. (Comptt. All India 2011)
Answer: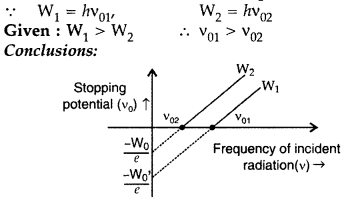

(i) Threshold frequency of material having work function W₁ is more than that of material of work function W₂.
(ii) The slopes of the straight line graphs, in both the cases, have the same value.
(iii) For the same frequency of incident radiation (> v₀₁), the maximum kinetic energy of the electrons, emitted from the material of work function W₁ is < that of electrons emitted from material of work function W₂.(any two)

Question 53.
(a) Why photoelectric effect can not be explained on the basis of wave nature of light? Give reasons.
(b) Write the basic features of photon picture of electromagnetic radiation on which Einstein’s photoelectric equation is based. (Delhi 2013)
Answer:
(a) (i) The maximum kinetic energy of the emitted electron should be directly proportional to the intensity of incident radiations but it is not observed experimentally. Also maximum kinetic energy of the emitted electrons should not depend upon incident frequency according to wave theory, but it is not so.
(ii) According to wave theory, threshold frequency should not exist. Light of all frequencies should emit electrons provided intensity of light is sufficient for electrons to eject.
(iii) According to wave theory, photoelectric effect should not be instantaneous. Energy of wave cannot be transferred to a particular electron but will be distributed to all the electrons present in the illuminated portion. Hence, there has to be a time lag between incidence of radiation and emission of electrons.

(b) Basic features of photon picture of electromagnetic radiation :
(i) Radiation behaves as if it is made of particles like photons. Each photon has energy E = hv and momentum p = h/λ.
(ii) Intensity of radiation can be understood in terms of number of photons falling per second on the surface. Photon energy depends only on frequency and is independent of intensity.
(iii) Photoelectric effect can be understood as the result of one to one collision between an electron and a photon.
(iv) When a photon of frequency
(v) is incident on a metal surface, a part of its energy is used in overcoming the work function and other part is used in imparting kinetic energy, so KE = h(v – v₀).

Question 54.
Write Einstein’s photoelectric equation and point out any two characteristic properties of photons on which this equation is based. Briefly explain the three observed features which can be explained by this equation. (All India, Comptt. All India 2013)
Answer:

Two characteristics properties of photons on which equation is based.
1. Photoelectric emission will take place only if frequency of incident radiation is greater than or equal to threshold frequency.
2. When a photon collides with an electron, it gives all its energy to electron.
Three features :

Question 55.
(a) State three important properties of photons which describe the particle picture of electromagnetic radiation.
(b) Use Einstein’s photoelectric equation to define the terms
(i) stopping potential and
(ii) threshold frequency. (Comptt. Delhi 2013)
Answer:
(a)
Basic features of photon picture of electromagnetic radiation :
(i) Radiation behaves as if it is made of particles like photons. Each photon has energy E = hv and momentum p = h/λ.
(ii) Intensity of radiation can be understood in terms of number of photons falling per second on the surface. Photon energy depends only on frequency and is independent of intensity.
(iii) Photoelectric effect can be understood as the result of one to one collision between an electron and a photon.
(iv) When a photon of frequency
(v) is incident on a metal surface, a part of its energy is used in overcoming the work function and other part is used in imparting kinetic energy, so KE = h(v – v₀).

(b) (i) Stopping potential or cut-off potential. The minimum value of the negative potential ‘V₀‘, which should be applied to the anode in a photo cell so that the photo electric current becomes zero, is called stopping potential.
The maximum kinetic energy (K_max) of photoelectrons is given by,

(ii) Threshold frequency. The minimum frequency V₀, which the incident light must possess so as to eject photoelectrons from a metal surface, is called threshold frequency of the metal.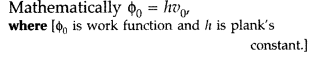

Question 56.
An electron microscope uses electrons accelerated by a voltage of 50 kV. Determine the de-Broglie wavelength associated with the electrons. Taking other factors, such as numerical aperture etc. to be same, how does the resolving power of an electron microscope compare with that of an optical microscope which uses yellow light? (All India 2014)
Answer: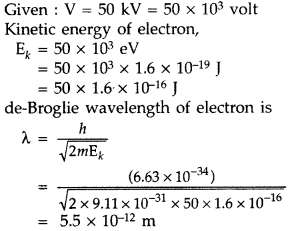

For yellow light, wavelength X = 5.9 × 10^-7 m Since resolving power (R.P.) is inversely proportional to wavelength, therefore, R.P. of an electron microscope is about 10⁵ times more than optical microscope.

Question 57.
Write Einstein’s photoelectric equation and mention which important features in photoelectric effect can be explained with the help of this equation.
The maximum kinetic energy of the photoelectrons gets doubled when the wavelength of light incident on the surface changes from λ₁ to λ₂. Derive the expressions for the threshold wavelength λ₀ and work function for the metal surface. (Delhi 2014)
Answer: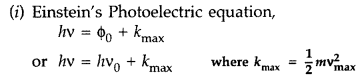

(ii) Important features of photoelectric effect:
(a) Radiation behaves as if it is made of particles like photons. Each photon has energy E = hv and momentum p = h/λ.
(b) Intensity of radiation can be understood in terms of number of photons falling per second on the surface. Photon energy
depends only on frequency and is independent of intensity.
(c) Photoelectric effect can be understood as the result of the one to one collision between an electron and a photon.
(d) When a photon of frequency
(v) is incident on a metal surface, a part of its energy is used in overcoming the work function and other part is used in imparting kinetic energy, so KE = h(v – v₀)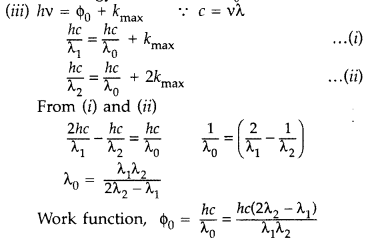

Question 58.
(a) Describe briefly three experimentally observed features in the phenomenon of photoelectric effect.
(b) Discuss briefly how wave theory of light cannot explain these features. (All India 2014)
Answer:
(a) Experimental features and observations of photoelectric effect :
(i) For a given photosensitive material and frequency of incident radiation (above the threshold frequency), the photoelectric current is directly proportional to the intensity of incident light.
(ii) For a given photosensitive material and frequency of incident radiation, saturation current is found to be proportional to the intensity of incident radiation whereas the stopping potential is independent of its intensity.
(iii) For a given photosensitive material, there exists a certain minimum cut-off frequency of the incident radiation, called the threshold frequency, below which no emission of photoelectrons takes place, no matter how intense the incident light is. Above the threshold frequency, the stopping potential or equivalently the maximum kinetic energy of the emitted photoelectrons increases linearly with the frequency of the incident radiation, but is independent of its intensity.
(iv) The photoelectric emission is an instantaneous process without any apparent time lag (~10^-9 s or less), even when the incident radiation is made exceedingly dim.

(b) Wave theory cannot explain photoelectric effect:
(i) According to the wave picture of light, the free electrons at the surface of the metal (over which the beam of radiation falls) absorb the radiant energy continuously. The greater the intensity of radiation, the greater are the amplitude of electric and magnetic fields. Consequently, the greater the intensity, the greater should be the energy absorbed by each electron. In this picture, the maximum kinetic energy of the photoelectrons on the surface is then expected to increase with increase in intensity. Also, no matter what the ‘ frequency of radiation is, a sufficiently intense beam of radiation (over sufficient time) should be able to impart enough energy to the electrons, so that they exceed the minimum energy needed to escape from the metal surface. A threshold frequency, therefore, should not exist. These expectations of the wave theory directly contradict observations (a) (i), (ii) and (iii) given above.

(ii) In the wave picture, the absorption of energy by electrons takes place continuously over the entire wavefront of the radiation. Since a large number of electrons absorb energy, the energy absorbed per electron per unit time turns out to be small. Explicit calculations estimate that it can take hours or more for a single electron to pick up sufficient energy to overcome the work function and come out of the metal. This conclusion is again in striking contrast to observation (iv) that the photoelectric emission is instantaneous.
In short, the wave picture is unable to explain the most basic features of photoelectric emission.

Question 59.
(a) Write the important properties of photons which are used to establish Einstein’s photoelectric equation.
(b) Use this equation to explain the concept of
(i) threshold frequency and
(ii) stopping potential. (All India 2014)
Answer:
(a) Important properties of Photons :
(i) In interaction of radiation with matter, radiation behaves as if it is made up of particles called photons.
(ii) Each photon has energy E (= hv) and momentum p (= hv/c), and speed c, the speed of light.
(iii) All photons of light of a particular frequency v, or wavelength λ, have the same energy E (= hv = hc/λ) and momentum p (= hv/c = h/λ), whatever the intensity of radiation may be. By increasing the intensity of light of given wavelength, there is only an increase in the number of photons per second crossing a given area, with each photon having the same energy. Thus, photon energy is independent of intensity of radiation.
(iv) Photons are electrically neutral and are not deflected by electric and magnetic fields.
(v) In a photon-particle collision (such as photon-electron collision), the total energy and total momentum are conserved. However, the number of photons may not be conserved in a collision. The photon may be absorbed or a new photon may be created.
(b) Einstein’s photoelectric equation is

This equation shows that the greater the work function ϕ₀, higher the threshold frequency v₀ needed to emit photoelectrons.
Thus, there exists a threshold frequency v₀ (=ϕ₀/h the metal surface, below which no photoelectric emission is possible, no matter how intense the incident radiation may be or how long it falls on the surface.
(ii) Stopping potential. The minimum value of negative potential v₀, which should be applied to the anode in a photocell, so that the photoelectric current becomes zero, is called Stopping potential.

Question 60.
Write three characteristic features in photoelectric effect which cannot be explained on the basis of wave theory of light, but can be explained only using Einstein’s equation. (Delhi 2016)
Answer:
(a) (i) The maximum kinetic energy of the emitted electron should be directly proportional to the intensity of incident radiations but it is not observed experimentally. Also maximum kinetic energy of the emitted electrons should not depend upon incident frequency according to wave theory, but it is not so.
(ii) According to wave theory, threshold frequency should not exist. Light of all frequencies should emit electrons provided intensity of light is sufficient for electrons to eject.
(iii) According to wave theory, photoelectric effect should not be instantaneous. Energy of wave cannot be transferred to a particular electron but will be distributed to all the electrons present in the illuminated portion. Hence, there has to be a time lag between incidence of radiation and emission of electrons.

Question 61.
Sketch the graph showing variation of stopping potential with frequency of incident radiations for two photosensitive materials A and B having threshold frequencies v_A > v_B.
(i) In which case is the stopping potential more and why?
(ii) Does the slope of the graph depend on the nature of the material used? Explain. (All India 2016)
Answer: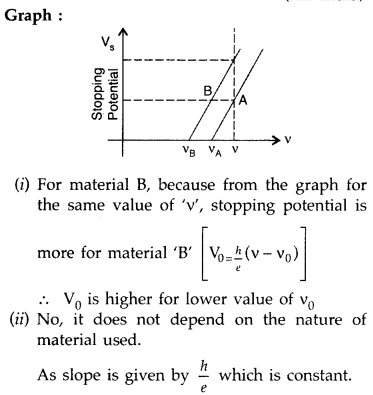

Question 62.
The graphs, drawn here, are for the phenomenon of photoelectric effect.


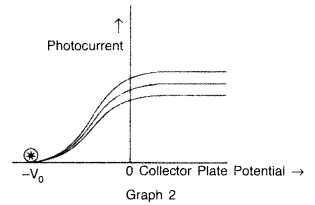
(i) Identify which of the two characteristics (intensity/frequency) of incident light, is being kept constant in each case.

(iii) Justify the existence of a ‘threshold frequency’ for a given photosensitive surface. (Comptt. Delhi 2016)
Answer:
(i) (a) In graph 1 : intensity is being kept constant.
(b) In graph 2 : frequency is being kept constant.
(ii) (a) In graph 1 : Saturation current
(b) In graph 2 : Stopping potential.
(iii) For a given photo-sensitive surface electrons need a minimum energy to be emitted, this is called work function of the surface W.
∴ Photons energy hv should be greater/ equal to the work function.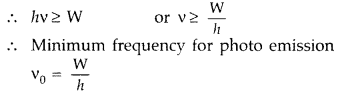

which is justified to be called as threshold frequency.

Question 63.
Point out two distinct features observed experimentally in photoelectric effect which’ cannot be explained on the basis of wave theory of light. State how the ‘photon picture’ of light provides an explanation of these features. (Comptt. All India 2016)
Answer:
(a) (i) The maximum kinetic energy of the emitted electron should be directly proportional to the intensity of incident radiations but it is not observed experimentally. Also maximum kinetic energy of the emitted electrons should not depend upon incident frequency according to wave theory, but it is not so.
(ii) According to wave theory, threshold frequency should not exist. Light of all frequencies should emit electrons provided intensity of light is sufficient for electrons to eject.
(iii) According to wave theory, photoelectric effect should not be instantaneous. Energy of wave cannot be transferred to a particular electron but will be distributed to all the electrons present in the illuminated portion. Hence, there has to be a time lag between incidence of radiation and emission of electrons.

(b) Basic features of photon picture of electromagnetic radiation :
(i) Radiation behaves as if it is made of particles like photons. Each photon has energy E = hv and momentum p = h/λ.
(ii) Intensity of radiation can be understood in terms of number of photons falling per second on the surface. Photon energy depends only on frequency and is independent of intensity.
(iii) Photoelectric effect can be understood as the result of one to one collision between an electron and a photon.
(iv) When a photon of frequency
(v) is incident on a metal surface, a part of its energy is used in overcoming the work function and other part is used in imparting kinetic energy, so KE = h(v – v₀).

Question 64.
(i) How does one explain the emission of electrons from a photosensitive surface with the help of Einstein’s photoelectric equation?
(ii) The work function of the following metals is given : Na = 2.75 eV, K = 2.3 eV, Mo = 4.17 eV and Ni 5.15 eV. Which of these metals will not cause photoelectric emission for radiation of wavelength 3300 A from a laser source placed 1 m away from these metals? What happens if the laser source is brought nearer and placed 50 cm away? (Delhi 2017)
Answer: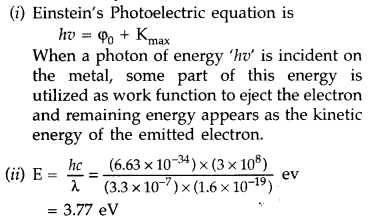

Because the work function of Mo and Ni is more than the energy of the incident photons; so photoelectric emission will not take place from these two metals Mo and Ni. When the laser source is brought nearer and placed 50 cm away, the kinetic energy of photo-electrons will not change, only photoelectric current will change.

Question 65.
In the study of a photoelectric effect the graph between the stopping potential V and frequency v of the incident radiation on two different metals P and Q is shown here:
(i) Which one of the two metals has higher threshold frequency?
(ii) Determine the work function of the metal which has greater value.
(iii) Find the maximum kinetic energy of electron emitted by light of frequency 8 × 10¹⁴ Hz for this metal. (Delhi 2017)
Answer: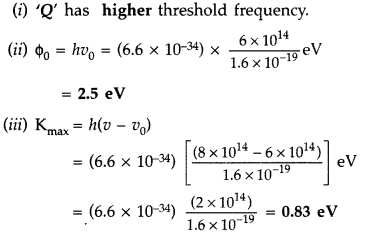

Question 66.
(i) State two important features of Einstein’s photoelectric equation.
(ii) Radiation of frequency 1015 Hz is incident on two photosensitive surfaces P and Q. There is no photoemission from surface P. Photoemission occurs from surface Q but photoelectrons have zero kinetic energy. Explain these observations and find the value of work function for surface Q. (Delhi 2017)
Answer:
(i)
Important features of photoelectric effect:
(a) Radiation behaves as if it is made of particles like photons. Each photon has energy E = hv and momentum p = h/λ.
(b) Intensity of radiation can be understood in terms of number of photons falling per second on the surface. Photon energy
depends only on frequency and is independent of intensity.
(c) Photoelectric effect can be understood as the result of the one to one collision between an electron and a photon.
(d) When a photon of frequency
(v) is incident on a metal surface, a part of its energy is used in overcoming the work function and other part is used in imparting kinetic energy, so KE = h(v – v₀)

(ii) Since no photoelectric emission takes place from P, it means frequency of incident radiation (10¹⁵ Hz) is less than its threshold frequency (v₀)_p.
Photo emission takes place from Q but kinetic energy of photoelectrons is zero. This implies that frequency of incident radiation is just equal to the threshold frequency of Q.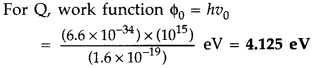

Question 67.
Using photon picture of light, show how Einstein’s photoelectric equation can be established. Write two features of photoelectric effect which cannot be explained by wave theory. (All India 2017)
Answer:
1st part :

This is Einstein’s photoelectric equation. Photoelectric emission is the result of interaction of two particles—one a photon of incident radiation and other an electron of photo sensitive metal. The free electrons are bound within the metal due to restraining forces on the surface. The minimum energy required to liberate an electron from the metal surface is called work function ϕ₀ of the metal. Each photon interacts with one electron. The energy hv of the incident photon is used up in two parts:
(a) a part of the energy of the photon is used in liberating the electron from the metal surface, which is equal to the work function ϕ₀ of the metal and
(b) the remaining energy of the photon is used in imparting K.E. of the ejected electron.
By the conservation of energy Energy of the inefficient photon = maximum K.E. of photoelectron + Work function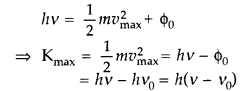

Three salient features are :
Three salient features observed in photoelectric effect on the basis of Einstein’s Photoelectric equation :

2nd part :
(a) (i) The maximum kinetic energy of the emitted electron should be directly proportional to the intensity of incident radiations but it is not observed experimentally. Also maximum kinetic energy of the emitted electrons should not depend upon incident frequency according to wave theory, but it is not so.
(ii) According to wave theory, threshold frequency should not exist. Light of all frequencies should emit electrons provided intensity of light is sufficient for electrons to eject.
(iii) According to wave theory, photoelectric effect should not be instantaneous. Energy of wave cannot be transferred to a particular electron but will be distributed to all the electrons present in the illuminated portion. Hence, there has to be a time lag between incidence of radiation and emission of electrons.

Question 68.
Explain giving reasons for the following:
(a) Photoelectric current in a photocell increases with the increase in the intensity of the incident radiation.
(b) The stopping potential (V₀) varies linearly with the frequency (v) of the incident radiation for a given photosensitive surface with the slope remaining the same for different surfaces.
(c) Maximum kinetic energy of the photoelectrons is independent of the intensity of incident radiation. (All India 2017)
Answer:
(a) The collision of a photon can cause emission of a photoelectron (above the threshold frequency). As the intensity increases, number of photons increases. Hence, the current increases.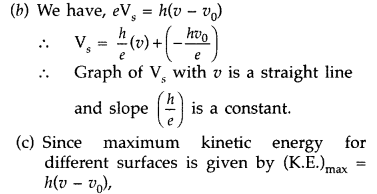

hence, it depends on the frequency and not on the intensity of the incident radiation.

Question 69.
The given graph shows the variation of photocurrent for a photosensitive metal:

(a) Identify the variable X on the horizontal axis.
(b) What does the point A on the horizontal axis represent?
(c) Draw this graph for three different values of frequencies of incident radiation v₁ v₂ and v₃ (v₁ > v₂ > v₃) for same intensity.
(d) Draw this graph for three different values of intensities of incident radiation I₁ I₂ and I₃ (I₁ > I₂ > I₃) having same frequency. (All India 2017)
Answer:
(a) ‘X’ is a collector plate potential.
(b) ‘A’ represents the stopping potential.
(c) Graph for different frequencies :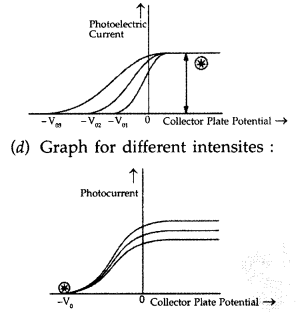

Question 70.
Draw a graph showing the variation of de Broglie wavelength of a particle of charge q and mass m with the accelerating potential. Proton and deuteron have the same de Broglie wavelengths. Explain which has more kinetic energy. (Comptt. Delhi 2017)
Answer: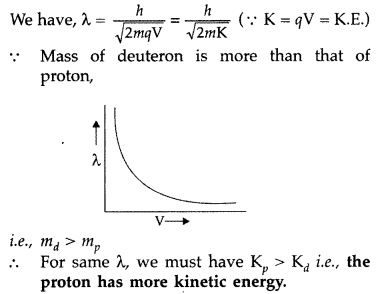

Question 71.
(a) Draw the graph showing the variation of de Broglie wavelength of a particle of charge q and mass m with the accelerating potential.
(b) An electron and proton have the same de Broglie wavelengths. Explain, which of the two has more kinetic energy. (Comptt. Delhi 2017)
Answer:
(a) For Graph :


Since the mass of electron is less than that of proton, hence electron will have more kinetic energy.

Question 72.
Draw a graph showing the variation of de Broglie wavelength λ of a particle of charge q and mass m, with the accelerating potential V. An α-particle and a proton have the same de-Broglie wavelength equal to 1Å. Explain with calculations, which of the two has more kinetic energy. (Comptt. Delhi 2017)
Answer:
For Graph

Question 73.
The photon emitted during the de-excitation from the 1st excited level to the ground state of hydrogen atom is used to irradiate a photo cathode of a photocell, in which stopping potential of 5 V is used. Calculate the work function of the cathode used. (Comptt. All India 2017)
Answer: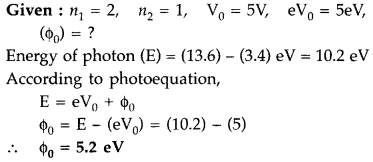

Question 74.
An electron microscope uses electrons accelerated by a potential difference 50 kV. Calculate the de Broglie wavelength of the electrons. Compare the resolving power of an electron microscope with that of an optical microscope, which uses visible light of wavelength 550 nm. Assume the numerical aperture of the objective lens of both microscopes are the same. (Comptt. All India 2017)
Answer: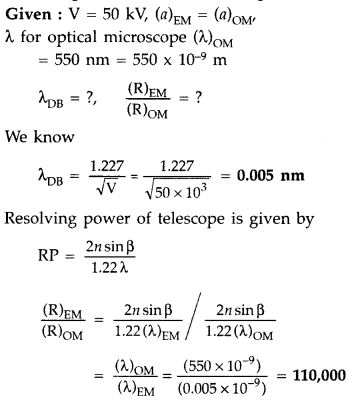

Question 75.
Two particles A and B of de Broglie wavelengths λ₁ and λ₂ combine to form a particle C. The process conserves momentum. Find the de Broglie wavelength of the particle C. (The motion is one dimensional.) (NCERJExemplar)
Answer:
The motion is one dimension, therefore
(i) If the particles move in the same direction

(ii) If the particles move in the opposite direction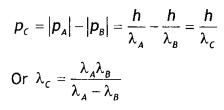

Question 76.
Find the frequency of light that ejects electrons from a metal surface, fully stopped by a retarding potential of 3.3 V. If photoelectric emission begins in this metal at a frequency of 8 × 10¹⁴ Hz, calculate the work function (in eV) for this metal.
Or
Monochromatic light of frequency 6.0 × 10¹⁴ Hz is produced by a laser. The power emitted is 2.0 × 10^-3W. Calculate the (i) energy of a photon in the light beam and (ii) the number of photons emitted on an average by the source. (CBSE Delhi 2018C)
Answer:
We have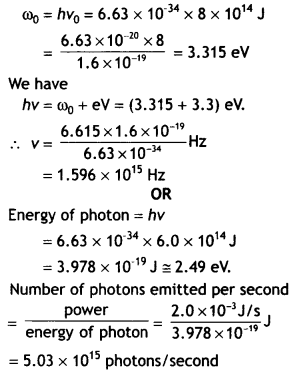

Long Answer Type

Question 1.
(a) Write three observed features of photoelectric effect which cannot be explained by wave theory of light.
Explain how Einstein’s photoelectric equation is used to describe these features satisfactorily.
(b) Figure shows a plot of stopping potential (v₀) with frequency (v) of incident radiation for two photosensitive materials M₁ and M₂.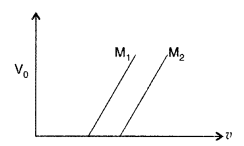

Explain
(i) why the slope of both the lines is same?
(ii) for which material emitted electrons have greater kinetic energy for the same frequency of incident radiation? (Comptt. All India 2017)
Answer:
(a)
(i) The maximum kinetic energy of the emitted electron should be directly proportional to the intensity of incident radiations but it is not observed experimentally. Also maximum kinetic energy of the emitted electrons should not depend upon incident frequency according to wave theory, but it is not so.
(ii) According to wave theory, threshold frequency should not exist. Light of all frequencies should emit electrons provided intensity of light is sufficient for electrons to eject.
(iii) According to wave theory, photoelectric effect should not be instantaneous. Energy of wave cannot be transferred to a particular electron but will be distributed to all the electrons present in the illuminated portion. Hence, there has to be a time lag between incidence of radiation and emission of electrons.

(b)
(i) The slope (V₀/v) of both the lines is the same and represents the universal constant known as ‘Planck’s constant’ (h) = 6.62 × 10^-34JS
(ii) For the same frequency of incident radiations, M₁ will have greater kinetic energy, because the value of V₀ is greater for M₁ material. It can be easily seen by drawing a vertical line (frequency being the same) and intersecting M₁ and M₂ at different points (V₀ for M₁ is higher)

Question 2.
(a) Describe briefly how wave nature of moving electrons was established experimentally.
(b) Estimate the ratio of de-Broglie wavelength associated with deuterons and a-particles when they are accelerated from rest through the same accelerating potential V. (Comptt. All India 2017)
Answer:
(a) Davisson and Germer experiment for wave nature of moving electrons : The experimental arrangement used by Davisson and Germer is schematically shown in the fig. It consists of an electron gun which comprises of . a tungsten filament F, coated with barium oxide and heated by a low voltage power supply (L.T. or battery). Electrons emitted by the filament are accelerated to a desired velocity by applying suitable potential/voltage from a high voltage power supply (H.T. or battery). They are made to pass through a cylinder with fine holes along its axis, producing a fine collimated beam.

The beam is made to fall on the surface of a nickel crystal. The electrons are scattered in all directions by the atoms of the crystal. The intensity of the electron beam, scattered in a given direction, is measured by the electron detector (collector)/ The detector can be moved on a circular scale and is connected to a sensitive galvanometer, which records the current. The deflection of the galvanometer is proportional to the intensity of the electron beam entering the collector. The apparatus is enclosed in an evacuated chamber. By moving the detector on the circular scale at different positions, the intensity of the scattered electron beam is measured for different values of angle of scattering 0 which is the angle between the incident and the scattered electron beams. The variation of the intensity (I) of the scattered electrons with the angle of scattering θ is obtained for different accelerating voltages.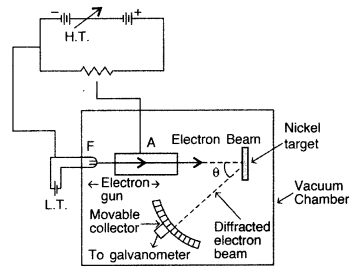

The experiment was performed by varying the accelarating voltage from 44 V to 68 V. It was noticed that a strong peak appeared in the intensity (I) of the scattered electron for an accelarating voltage of 54V at a scattering angle θ = 50°.

The appearance of the peak in a particular direction is due to the constructive interference of electrons scattered from different layers of the regularly spaced atoms of the crystals. From the electron diffraction measurements, the wavelength of matter waves was found to be 0.165 nm.

The de Broglie wavelength λ. associated with electrons, is given by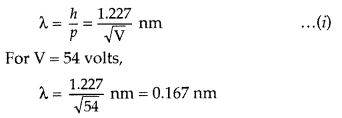

Thus, there is an excellent agreement between the theoretical value and the experimentally obtained value of de Broglie wavelength. Davisson-Germer experiment thus strikingly confirms the wave nature of electrons and the de Broglie relation.

Question 3.
What is the photoelectric effect? Write Einstein’s photoelectric equation and use it to explain (a) independence of maximum energy of emitted photoelectrons from the intensity of incident light and
(b) existence of a threshold frequency for the emission of photoelectrons.
Answer:
The election of photoelectrons from a metal surface when Light of suitabLe frequency is incident on it is catted photoelectric effect.

Einstein’s equation of photoelectric effect is \(\frac{1}{2}\)mv² = hv – ω₀
(a) In accordance with Einstein’s equation, the kinetic energy of the photoelectrons is independent of the intensity of the incident radiation.
(b) In accordance with Einstein’s equation, the kinetic energy will be positive and hence photoelectrons will be ejected if v > v₀. Thus below a certain frequency called threshold frequency, photoelectrons are not ejected from a metal surface (if v < v₀).

Question 4.
An electron of mass m and charge q is accelerated from rest through a potential difference of V. Obtain the expression for the de-Broglie wavelength associated with it. If electrons and protons are moving with the same kinetic energy, which one of them will have a larger de-Broglie wavelength associated with it? Give reason.
Answer:
Consider an electron of mass m and charge e to be accelerated through a potential difference of V volts. Let v be the velocity gained by it. Then kinetic energy of the electron is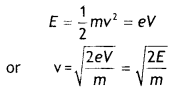

If λ is the de-Broglie wavelength associated with an electron, then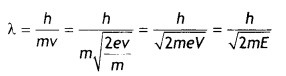

Since de-Broglie wavelength is inversely proportional to the square root of mass, the lesser the mass, the more is the de- Broglie wavelength. Since the mass of an electron is lesser than that of the proton, the electron has a greater de-Broglie wavelength than a proton.

Question 5.
Sketch the graphs showing the variation of stopping potential with the frequency of incident radiations for two photosensitive materials A and B having threshold frequencies v₀ > v’₀ respectively.
(a) Which of the two metals A or B has a higher work function?
(b) What information do you get from the slope of the graphs?
(c) What does the value of the intercept of graph ‘A’ on the potential axis represent?
Answer:
The graphs are as shown below.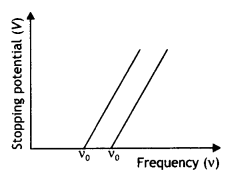

(a) The work function is directly proportional to the threshold frequency. The threshold frequency of metal A is greater than that of metal B; therefore A has a greater work function than B.
(b) The slope of the graphs gives the value of Planck’s constant.
(c) The intercept on the potential axis is negative (-W₀/e) w.r.t. stopping potential, i.e. Work function = e × magnitude of the intercept on the potential axis. We may infer it to give the voltage which, when applied with opposite polarity to the stopping voltage, will just pull out electrons from the metallic atom’s outermost orbit.

Question 6.
When a given photosensitive material is irradiated with light of frequency v, the maximum speed of the emitted photoelectrons equals Vmax. The graph shown in the figure gives a plot of V²max varying with frequency v.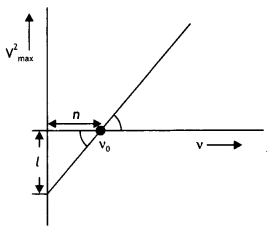

Obtain an expression for
(a) Planck’s constant, and
Answer:
By Einstein’s photoelectric equation we have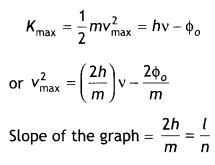

(b) The work function of the given photosensitive material in terms of the parameters T, ‘n’ and the mass ‘m’ of the electron.
Answer:
The intercept on V²_max axis is = \(\frac{2 \phi_{o}}{m}\) = l
Therefore, work function Φ₀ = \(\frac{ml}{2}\)

(c) How is threshold frequency determined from the plot? (CBSE AI 2019)
Answer:
The threshold frequency is the intercept on the v axis i.e. v₀ = n

Question 7.
X-rays fall on a photosensitive surface to cause photoelectric emission. Assuming that the work function of the surface can be neglected, find the relation between the de-Broglie wavelength (λ) of the electrons emitted to the energy (E_v) of the incident photons. Draw the nature of the graph for λ as a function of E_v. (CBSE Delhi 2014C)
Answer:
Consider an electron of mass m and charge e to be accelerated through a potential difference of V volt. Let v be the velocity gained by it. Then kinetic energy of the electron is

If λ is the de-Broglie wavelength associated with an electron, then
λ = \(\frac{h}{m v}=\frac{h}{\sqrt{2 m E_{v}}}\)

The nature of the graph is as shown.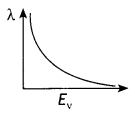

Question 8.
Light of intensity ‘l’ and frequency ‘v’ is incident on a photosensitive surface and causes photoelectric emission. What will be the effect on anode current when
(a) the intensity of light is gradually increased,
(b) the frequency of incident radiation is increased and
(c) the anode potential is increased?
In each case, all other factors remain the same. Explain giving justification in each case. (CBSE AI 2015)
Answer:
(a) Anode current will increase with the increase of intensity as the more the intensity of light, the more is the number of photons and hence more number of photoelectrons are ejected.
(b) No effect as the frequency of light affects the maximum K.E. of the emitted photoelectrons.
(c) Anode current will increase with anode potential as more anode potential will accelerate the more electrons till it attains a saturation value and gets them collected at the anode at a faster rate.

Question 9.
The graphs, drawn here, are for the phenomenon of the photoelectric effect.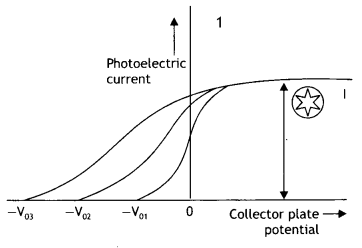


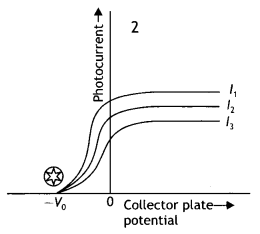
(a) Identify which of the two characteristics (intensity/frequency) of incident light is being kept constant in each case.
Answer:
Graph 1: Intensity, Graph 2: Frequency

(b) Name the quantity, corresponding to themark, in each case.
Answer:
Graph 1: Saturation current, Graph 2: stopping potential

(c) Justify the existence of a ‘threshold frequency’ for a given photosensitive surface. (CBSE Delhi 2016C)
Answer:
The electrons require minimum energy to set themselves free. This is called the work function. As the energy of the photon depends upon its frequency, the photons must possess a minimum frequency so that their energy becomes equal to or greater than the work function. This is called threshold frequency and is given by v₀ = \(\frac{\omega_{0}}{h}\)

Question 10.
Draw a graph showing the variation of de-Broglie wavelength λ of a particle of charge q and mass, with the accelerating potential V. An alpha particle and a proton have the same de-Broglie wavelength equal to 1 Å. Explain with calculations, which of the two has more kinetic energy. (CBSE Delhi 2017C)
Answer:
The graph is as shown.
The de-Broglie wavelength of a particle is given by the expression λ = \(\frac{h}{\sqrt{2 m q V}}\)

Since the alpha particle and the proton have the same de- Broglie wavelength, we have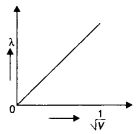

\(\frac{h}{\sqrt{2 m_{a} E_{\alpha}}}=\frac{h}{\sqrt{2 m_{p} E_{p}}}\)

Therefore proton has a greater value of de-Broglie wavelength.
Now kinetic energy is given by the expression
\(\frac{E_{a}}{E_{p}}=\frac{m_{p}}{m_{\alpha}}=\frac{m}{4 m}=\frac{1}{4}\)

Thus proton has more kinetic energy.

Question 11.
(a) Plot a graph showing the variation of photoelectric current with collector plate potential for different frequencies but of the same intensity of incident radiation.
Answer:
The graph between current I and plate potential.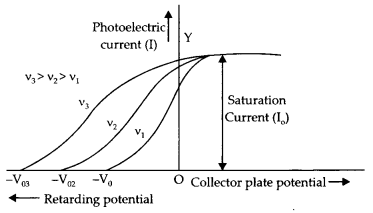

(b) Green and blue light of the same intensity are incident separately on the same photosensitive surface. If both of these cause photoelectric emission, which one will emit photoelectrons
(i) having greater kinetic energy and
Answer:
The blue light will emit photoelectrons having greater kinetic energy because the frequency and hence energy (E = hv) of blue light is more than the frequency and hence the energy of green light.

(ii) producing larger photocurrent? Justify your answer. (CBSE 2019C)
Answer:
Photoelectric current will be nearly the same for the blue and green light because the intensity of saturation current is independent of the frequency of incident light.

Question 12.
Radiation of frequency 10¹⁵ Hz is incident on two photosensitive surfaces P and Q. The following observations were recorded:
(a) Surface P: No photoemission occurs.
(b) Surface Q: Photoemission occurs but photoelectrons have zero kinetic energy. Based on Einstein’s photoelectric equation, explain the two observations.
Answer:
Einstein photoelectric equation is h(v – v₀) = \(\frac{1}{2}\) mv²
(a) As in the case of surface P no photoemission takes place, we conclude that threshold frequency for P has a value greater than the frequency 10¹⁵ Hz of the given radiation.
(b) In the case of surface Q photoemission takes place but the kinetic energy of photoelectrons means that this radiation just overcomes the work function of the metal. In other words, the frequency 10¹⁵ Hz, of this radiation is equal to the threshold frequency.

Question 13.
When the light of frequency v₁ is incident on a photosensitive surface, the stopping potential is V₁ If the frequency of incident radiation becomes v₁/2, the stopping potential changes to V₂. Find out the expression for the threshold frequency for the surface in terms of V₁ and V₂.
If the frequency of incident radiation is doubled, will the maximum kinetic energy of the photoelectrons also be doubled? Give reason. (CBSE AI 2019)
Answer:
By Einstein’s photoelectric equation.
eV₁ = hv₁ – hv₀ …(i).
and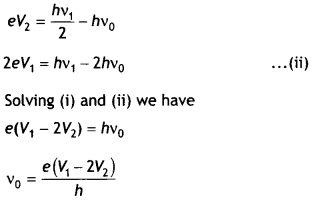

If the frequency is doubled, the maximum kinetic energy will not be doubled.
K_max = hv – hv₀
K_new = 2hv – hv₀ = hv + K_max

Question 14.
(a) Define the terms
(i) threshold frequency and
Answer:
Threshold frequency (v₀) is the minimum value of frequency of incident radiations below which the photoelectric emission stops, altogether.

(ii) stopping potential in the context of the photoelectric effect.
Answer:
Stopping potential is the value of retarding potential at which the photoelectric current becomes zero for the given frequency of incident radiations.

(b) Draw a graph showing the variation of stopping potential (V₀) with frequency (v) of incident radiation for a given photosensitive material. (CBSE 2019C)
Answer:
The graph between v and V₀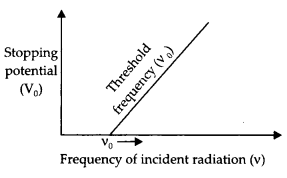

Question 15.
State Einsteins photoelectric equation explaining the symbols used.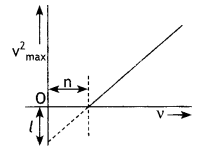

Light of frequency y is incident on a photosensitive surface. A graph of the square of the maximum speed of the electrons (v²_max) vs. y is obtained as shown in the figure. Using Einstein’s photoelectric equation, obtain expressions for (i) Planck’s constant and (ii) the work function of the given photosensitive material in terms of parameters l, n and mass of the electron m. (CBSE Delhi 2018C)
Answer:
Einstein’s photoelectric equation is
hv = hv₀ (= ω₀) = \(\frac{1}{2}\)mv2max
v = frequency of incident Light
ω₀ = threshold frequency of photosensitive A material.
ω₀ = work function.
\(\frac{1}{2}\)mv2max = max. the kinetic energy of the emitted photoelectrons

(Also accept if the student writes)
hv = ω₀ + eV_s
ω₀ = Work function of photosensitive material
V_s = stopping potential)

From Einstein’s photoelectronic equation,
we have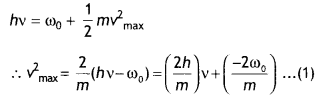

(i) Slope of the given graph = \(\frac{l}{n}\)
∴ \(\frac{2 h}{m}=\frac{l}{n}\)
or
h = \(\frac{m l}{2 n}\)

(ii) Intercept on the y-axis = – l
From equation (1)
– l = \(\frac{-2 \omega_{0}}{m}\) ⇒ ω₀ = \(\frac{ml}{2}\)

Question 16.
(a) In the photoelectric effect, do all the electrons that absorb a photon comes out as photoelectrons irrespective of their location? Explain.
Answer:
No, it is not necessary that if the energy supplied to an electron is more than the work function, it will come out. The electron after receiving energy may lose energy to the metal due to collisions with the atoms of the metal. Therefore, most electrons get scattered into the metal. Only a few electrons near the surface may come out of the surface of the metal for whom the incident energy is greater than the work function of the metal.

(b) A source of light, of frequency greater than the threshold frequency, is placed at a distance be ‘d’ from the cathode of a photocell. The stopping potential is found to be V. If the distance of the light source is reduced to d/n (where n > 1), explains the changes that are likely to be observed in the (a) photoelectric current and (b) stopping potential. (CBSE Sample Paper 2018-19)
Answer:

1. On reducing the distance, intensity increases. Photoelectric current increases with the increase in intensity.
2. Stopping potential is independent of intensity and therefore remains unchanged.

Question 17.
Explain the laws of the photoelectric effect on the basis of Einstein’s photoelectric equation.
Answer:
The laws of the photoelectric effect can be explained on the basis of Einstein’s photoelectric equation, i.e.
\(\frac{1}{2}\)mv² = h (v – v₀)
(a) From the equation we find that the kinetic energy will be positive and hence photoelectrons will be emitted if v > v₀, i.e. the incident frequency is greater than the threshold frequency.

(b) It is clear from the expression that the kinetic energy of the emitted photoelectrons varies linearly with the frequency of the incident radiation.

(c) According to Einstein highly energetic radiation has a high frequency and an intense beam of radiation has more photons. Therefore when a high-intensity beam of radiation is incident on the metal surface, a large number of photoelectrons are emitted as the photoelectric effect is a one-one phenomenon, i.e. one photon can eject one photoelectron. Thus the more the intensity of the incident radiation, the more is the photoelectric current.

(d) As soon as a photon is an incident on a metal surface it is immediately absorbed by an electron, which sets itself free and comes out of the metal surface. The time lag is being negligible. Thus the photoelectric effect is instantaneous.

Question 18.
Define the terms threshold frequency and stopping potential in relation to the phenomenon of the photoelectric effect. How is the photoelectric current affected on increasing the
(i) frequency and
(ii) the intensity of the incident radiations and why?
Answer:
Stopping potential (V₀): The negative potential of the plate at which no photoelectrons reach is called the stopping potential or the cut-off potential.

Threshold frequency (v₀): The minimum frequency of the incident radiation, which can eject photoelectrons from a material, is known as the threshold frequency or cut-off frequency of the material.

1. When the frequency has increased the energy absorbed by a single electron on collision with a photon also increases. E= hv, h is Planck’s constant. This raises the kinetic energy of the emitted photoelectrons but has no effect on current as the number of electrons being liberated remains the same.
2. When the intensity of the incident radiation is increased, the number of photons crossing a unit area per second increases. These photons liberate more electrons and hence current increases.

Question 19.
Write Einstein’s photoelectric equation and mention which important features in the photoelectric effect can be explained with the help of this equation.
The maximum kinetic energy of the photoelectrons gets doubled when the wavelength of light incident on the surface changes from λ₁ to λ₂. Derive the expressions for the threshold wavelength λ₀ and work function for the metal surface. (CBSE Delhi 2015)
Answer:
Einstein’s equation is
E_max = hv – ω or \(\frac{1}{2}\)mv²max = hv – ω

Here m is the mass of the photoelectron and v_max is maximum velocity.
(a) In accordance with Einstein’s equation, the kinetic energy of the photoelectrons is independent of the intensity of the incident radiation.
(b) In accordance with Einstein’s equation, the kinetic energy will be positive and hence photoelectrons will be ejected if v > v_o. Thus below a certain frequency called the threshold frequency, photoelectrons are not ejected from a metal surface.
Given E₁ = E, E₂ = 2E,
By Einstein’s equation we have

Question 20.
Point out two distinct features observed experimentally in the photoelectric effect which cannot be explained on the basis of the wave theory of light. State how the ‘photon picture’ of light provides an explanation of these features. (CBSE AI 2016C)
Answer:
The two features are

1. The intensity of light determines the photoelectric current and
2. Existence of threshold frequency.

According to the wave picture of light, the free electrons at the surface of the metal (over which the beam of radiation falls) absorb the radiant energy continuously. The greater the intensity of radiation, the greater is the amplitude of electric and magnetic fields. Consequently, the greater the intensity, the greater should be the energy absorbed by each electron. In this picture, the maximum kinetic energy of the photoelectrons on the surface is then expected to increase with the increase in intensity.

Also, no matter what the frequency of radiation is, a sufficiently intense beam of radiation (over sufficient time) should be able to impart enough energy to the electrons so that they exceed the minimum energy needed to escape from the metal surface. A threshold frequency, therefore, should not exist, which is against the observations. Thus the photoelectric effect cannot be explained on the basis of the wave nature of light

Question 21.
Using photon picture of light, show how Einstein’s photoelectric equation can be established. Write two features of the photoelectric effect that cannot be explained by the wave theory. (CBSE AI 2017)
Answer:
According to Einstein, the emission of a photoelectron is the interaction of quanta of light with a single electron. The photon is completely absorbed by the electron. The electron, which absorbs the photon, utilises this energy to set itself free. An amount of energy equal to the work function (ω) is required by the electron to set itself free. The remaining energy hv – ω (if hv > ω) is available to the electron as its maximum kinetic energy. Thus according to Einstein, we have

Here m is the mass of the photoelectron and v_max is maximum velocity. Actually, most of the electrons possess kinetic energy less than the maximum kinetic energy because they lose a part of their energy due to collisions with the atoms on their way out from inside the metal. Thus electrons with different kinetic energy are emitted.

Now the work function is given by ω = hv_o, therefore the above equation becomes
\(\frac{1}{2}\)mv²_max = hv – hv_o = h(v – v_o)

(a) Dependence of kinetic energy on the frequency of the incident radiation.
(b) Existence of threshold frequency.

Question 22.
(a) How does one explain the emission of electrons from a photosensitive surface with the help of Einstein’s photoelectric equation?
(b) The work function of the following metals is given: Na = 2.75 eV, K = 2.3 eV, Mo = 4.17eV and Ni = 5.15 eV. Which of these metals will not cause photoelectric emission for radiation of wavelength 3300 Å from a laser source placed 1 m away from these metals? What happens if the laser source is brought nearer and placed 50 cm away? (CBSE Delhi 2017)
Answer:
(a) According to Einstein’s equation we have
\(\frac{1}{2}\)mv² = h (v – v_o)

If the frequency of the incident radiation is above the threshold frequency (v_o), the KE of the photo¬electrons will be positive and hence will be emitted.

Let us calculate the energy possessed by the photon of wavelength 3300 Å.

As the energy of the incident photon is less than the work functions of Mo and Ni, so the metals Mo and Ni will not give photoelectric emission.

If the laser is brought closer, the intensity of radiation increases. This does not affect the result regarding Mo and Ni, but the photoelectric current will increase for Na and K with the increase in intensity.

Question 23.
When a monochromatic yellow coloured light beam is incident on a given photosensitive surface, photoelectrons are not ejected, while the same surface gives photoelectrons when exposed to the green coloured monochromatic beam. What will happen if the same surface is exposed to (i) violet and (ii) red coloured monochromatic beam of light? Justify your answer.
Answer:
Photoelectrons are ejected from a metal surface if the frequency of incident radiation is greater than the threshold frequency of that metal.

Since yellow light does not eject photoelectrons while green light does, the threshold frequency of the given metal is greater than the frequency of yellow light.
(a) Violet light has a greater frequency than yellow light, therefore when violet light is incident on the metal surface photoelectrons will be ejected.
(b) Red light has a smaller frequency than yellow light; therefore when the red light is incident on the metal surface, photoelectrons will not be ejected.

Question 24.
(a) Why photoelectric effect cannot be explained on the basis of the wave nature of light? Give reasons.
Answer:
According to the wave picture of light, the free electrons at the surface of the metal (over which the beam of radiation falls) absorb the radiant energy continuously. The greater the intensity of radiation, the greater is the amplitude of electric and magnetic fields.

Consequently, the greater the intensity, the greater should be the energy absorbed by each electron. In this theory, the maximum kinetic energy of the photoelectrons on the surface is then expected to increase with the increase in intensity.

Also, no matter what the frequency of radiation is, a sufficiently intense beam of radiation (over sufficient time) should be able to impart enough energy to the electrons so that they exceed the minimum energy needed to escape from the metal surface. A threshold frequency, therefore, should not exist, which is against the observations. Thus the photoelectric effect cannot be explained on the basis of the wave nature of light.

(b) Write the basic features of the photon picture of electromagnetic radiation on which Einstein’s photoelectric equation is based. (CBSE Delhi 2013)
Answer:
The basic features are

1. Radiation energy is built up of discrete units.
2. An electron absorbs the quanta of energy.

Question 25.
Obtain the expression for the wavelength of the de-Broglie wave associated with an electron accelerated from rest through a potential difference V.
The two lines A and B as shown in the graph plot the de-Broglie wavelength A. as a function of 1/\(\sqrt{V}\) (V is the accelerating potential) for two particles having the same charge. Which of the two represents the particle of heavier mass?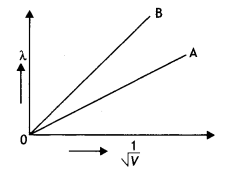

Answer:
Consider an electron of mass m and charge e to be accelerated through a potential difference of V volts. Let v be the velocity gained by it. Then kinetic energy of an electron is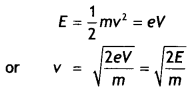

If λ is the de-Broglie wavelength associated with an electron, then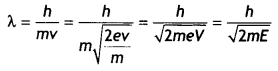

In graphs, the charge of both particLes is the same, but the slope of graph A is Less. It means that mass of particle A is more (since slope = \(\frac{h}{\sqrt{2 m E}}\)).

Question 26.
The given graphs show the variation of the stopping potential Vs with the frequency (v) of the incident radiations for two different photosensitive materials M1 and M2.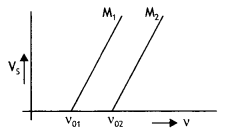

(i) What are the values of work functions for M₁ and M₂?
(ii) The values of the stopping potential for M₁ and M₂ for a frequency (v₃) of the incident radiations greater than v_o2 are V₁ and V₂ respectively. Show that the slope of the lines equals \(\frac{V_{1}-V_{2}}{v_{02}-v_{01}}\)
Answer:
(i) The values of work functions for M₁ and M₂ are ω₁ = hv_o1 and ω₂ = hv_o2.
(ii) The slope of the graphs is given by the variation of quantity along the Y-axis ‘ to the variation in quantity on the X-axis.

Now for M₁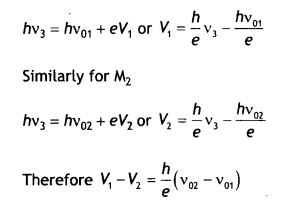

Therefore slope of the graphs is
\(\frac{h}{e}=\frac{V_{1}-V_{2}}{v_{02}-v_{01}}\)

Question 27.
Why are de-Broglie waves associated with a moving football not visible?
The wavelength of a photon and the de-Broglie wavelength of an electron have the same value. Show that energy of the photon is \(\frac{2λmc}{h}\) times the kinetic energy of the electron, where m, c and h have their usual meanings.
Answer:
(i) The de-Broglie wavelength of moving particles is given by the expression
λ = \(\frac{h}{mv}\). For a football the mass is large, hence the wavelength is extremely small and hence is not visible.

(ii) Now energy of a photon of wavelength λ. is E = hv = \(\frac{hc}{λ}\) ….(1)
and kinetic energy of an electron is Ke = \(\frac{1}{2}\)mv²

If de-Broglie wavelength (A) of electron be,
λ = \(\frac{h}{mv}\)
or
v = \(\frac{h}{mλ}\)

Therefore kinetic energy of electron will be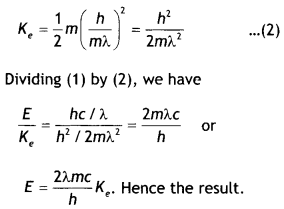

Numerical Problems:

Question 1.
What is the de Brogue wavelength associated with an electron, accelerated through a potential difference of 100 volts? (NCERT)
Answer:
Accelerating potential V = 100 V. The de-Broglie wavelength λ is
λ = \(\frac{1.227}{\sqrt{V}}\)nm = \(\frac{1.227}{\sqrt{100}}\) = 0.123 nm
The de-Broglie wavelength associated with an electron in this case is of the order of X-ray wavetengths.

Question 2.
In an experiment on the photoelectric effect, the slope of the cut-off voltage versus frequency of incident light is found to be 4.12 × 10^-15 V s. Calculate the value of Planck’s constant. (NCERT)
Answer:
Given slope = 4.12 × 10^-15 V s, h = 1

The slope of the voltage versus frequency graph gives the value of h/e, therefore we have
h = Slope × e = 4.12 × 10^-15 × 1.6 × 10^-19
Or
h = 6.59 × 10^-34 J s

Question 3.
The threshold frequency for a certain metal is 3.3 × 10¹⁴ Hz. If the light of frequency 8.2 × 10¹⁴ Hz is incident on the metal, predict the cut-off voltage for the photoelectric emission. (NCERT)
Answer:
Given v_o = 3.3 × 10¹⁴ Hz, v = 8.2 × 10¹⁴ Hz, V_o = ?

Using the relation h (v – v_o) = eV0 we have h(v – v_o)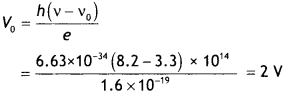

Question 4.
The work function for a certain metal is 4.2 eV. Will this metal give photoelectric emission for incident radiation of wavelength 330 nm? (NCERT)
Answer:
Given ω_o = 4.2 eV
The energy possessed by the incident radiation of wavelength 330 nm is
E = \(\frac{h c}{\lambda}=\frac{6.63 \times 10^{-34} \times 3 \times 10^{8}}{330 \times 10^{-9} \times 1.6 \times 10^{-19}}\) = 3.8 eV

Since this energy is less than the work function of the metal, no photoelectric emission will take place.

Question 5.
Light of frequency 7.21 × 10¹⁴ Hz is incident on a metal surface. Electrons with a maximum speed of 6.0 × 10 m s^-1 are ejected from the surface. What is the threshold frequency for the photoemission of electrons? (NCERT)
Answer:
Given v = 7.21 × 10¹⁴ Hz, v = 6.0 × 10 m s^-1, v_o = ?
Using the relation h (v – v_o) = \(\frac{1}{2}\)mv² We have

Question 6.
Calculate the (a) momentum and (b) de Broglie wavelength of the electrons accelerated through a potential difference of 56 V. (NCERT)
Answer:
Given V = 56 V, p = ?, λ = ?
Using the relation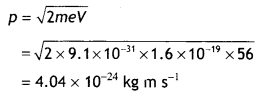

The de-Broglie wavelength is given by
λ = \(\frac{h}{p}=\frac{6.63 \times 10^{-34}}{4.04 \times 10^{-24}}\) = 0.164 nm

Question 7.
A particle is moving three times as fast as an electron. The ratio of the de-Broglie wavelength of the particle to that of the electron is 1.813 × 10^-4. Calculate the particle’s mass and identify the particle. (NCERT)
Answer:
Givenv = 3v_e, λ/λ_e = 1.813 × 10^-4
We know that λ = \(\frac{h}{p}=\frac{h}{m v}\)

therefore we have
m = me\(\frac{\lambda_{e} v_{e}}{\lambda v}=\frac{9.11 \times 10^{-31}}{1.813 \times 10^{-4} \times 3}\)

Solving we have m = 1.675 × 10^-27 kg
This is the mass of a proton or a neutron.

Question 8.
Using the graph shown in the figure for stopping potential v/s incident frequency of photons, calculate Planck’s constant. (CBSE Delhi 2015C)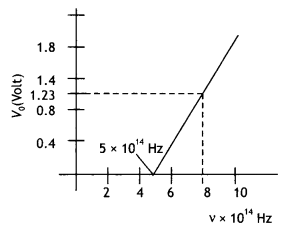

Answer:
By Einstein’s equation we have eV_o = h(v – v_o)

Therefore slope of the graph is \(\frac{V_{0}}{v}=\frac{h}{e}\). Now slope of graph is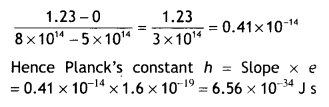

Question 9.
If the light of wavelength 412.5 nm is incident on each of the metals given below, which ones will show photoelectric emission and why? (CBSE AI Delhi 2018)

Metal	Work Function (eV)
Na	1.92
K	2.15
Ca	3.20
Mo	4.17

Answer:
The energy of a photon of the given light is,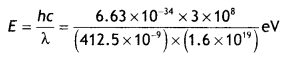

E = 3.01 eV. Thus, metals having a work function less than the energy of a photon of falling light will show the photoelectric effect So, Na and K will show the photoelectric effect.

Question 10.
Two metals A and B have work functions 2 eV and 5 eV respectively. Which metal will emit electrons, when irradiated with light of wavelength 400 nm and why?
Answer:
The energy possessed by radiation of wavelength 400 nm is E = hv = hc/λ or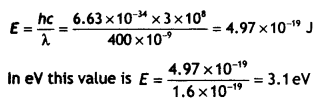

Since this energy is more than the work function of metal A, metal A will emit electrons.

Question 11.
Calculate the maximum kinetic energy of electrons emitted from a photosensitive surface of work function 3.2 eV, for the incident radiation of wavelength 300 nm.
Answer: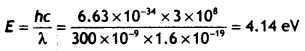

Therefore the kinetic energy of the emitted photoelectrons is
K.E_max = hv – hv_o = 4.14 – 3.2 = 0.94 ev

Question 12.
The work function, for a given photosensitive surface, equals 2.5 eV. When the light of frequency y falls on this surface, the emitted photoelectron is completely stopped by applying a retarding potential of 4.1 V. What is the value of y?
Answer: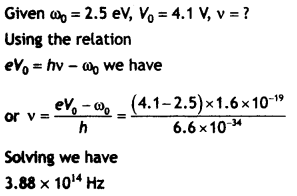

Question 13.
A nucleus of mass M Initially at rest splits into two fragments of masses M’/3 and 2M’/3 (M > M’). Find the ratio of de-Broglie wavelengths of the two fragments.
Answer:
By the principle of conservation of momentum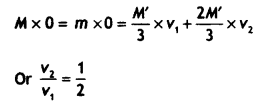

The ratio of de-Broglie wavelength is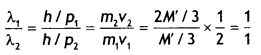

Question 14.
An electron microscope uses electrons accelerated by a voltage of 50 kV. Determine the de-Broglie wavelength associated with the electrons. Taking other factors, such as numerical aperture etc. to be the same, how does the resolving power of an electron microscope compare with that of an optical microscope which uses yellow light? (CBSE AI 2014)
Answer:
Given V = 50 kV, λ = ?
Using the expression λ = \(\frac{1.227}{\sqrt{V}}\) nm we have
λ = \(\frac{1.227}{\sqrt{50 \times 10^{3}}}\) nm = 5.48 × 10^-12 m

Wavelength of yellow light λy = 5.9 × 10^-7 m

Now RP ∝ \(\frac{1}{λ}\)

Therefore we have

Question 15.
A proton and a deuteron are accelerated through the same accelerating potential. Which one of the two has
(a) the greater value of de-Broghe wavelength associated with it and
Answer:
(a) The de-Broglie wavelength is given by the expression λ = \(\frac{h}{\sqrt{2 m q V}}\). Since potential is same, we have

Or
λ_p = \(\sqrt{2} \lambda_{a}\)

Thus proton has a greater value of de-Broglie wavelength.

(b) less momentum? Give reasons to justify your answer. (CBSE Delhi 2014)
Answer:
Now p = h/λ.

As the wavelength of a proton is more than that of a deuteron, the momentum of a proton is lesser than that of a deuteron. Hence, the momentum of the proton is less.

Question 16.
(a) Monochromatic light of frequency 6.0 × 10¹⁴ Hz is produced by a laser. The power emitted is 2.0 × 10^-3 W. Estimate the number of photons emitted per second on an average by the source.
Answer:
Each photon has energy
E = hv = (6.63 × 10^-34) × (6.0 × 10¹⁴)
= 3.98 × 10^-19 J

If N is the number of photons emitted by the source per second, the power P transmitted in the beam equals N times the energy per photon E so that P = NE, then
N = \(\frac{P}{E}=\frac{2.0 \times 10^{-3}}{3.98 \times 10^{-19}}\)
= 5.0 × 10¹⁵ photons per second.

(b) Draw a plot showing the variation of photoelectric current versus the intensity of incident radiation on a given photosensitive surface. (CBSE Delhi 2014)
Answer:
With the increase of intensity of radiations, photocurrent increase.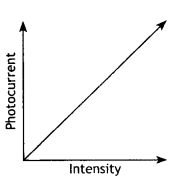

Question 17.
An electron is revolving around the nucleus with a constant speed of 2.2 × 10⁸ m s^-1. Find the de Broglie wavelength associated with it. (CBSE AI 2014C)
Answer:
Given v= 2.2 × 10⁸ m s^-1, λ = ?
Using the expression

Question 18.
Given the ground state energy E0 = – 13.6eV and Bohr radius αo = 0.53 Å. Find out how the de Broglie wavelength associated with the electron orbiting in the ground state would change when it jumps into the first excited state. (CBSE AI 2015)
Answer:
The de-Broglie wavelength is given by 2πr_n = nλ

In ground state, n = 1 and r0 = 0.53 Å, therefore λ_o = 2 × 3.14 × 0.53 = 3.33 Å
In first excited state, n = 2 and
r₁ = 4 × 0.53 Å = 2.12 Å,

therefore λ₁ = (2 × 3.14 × 2.12)/2 = 6.66 Å
Therefore λ₁ – λ_o = 6.66 – 3.33 = 3.33 Å

In other words, the de-Broglie wavelength becomes double.

Question 19.
A proton and an a-particle have the same de-Broglie wavelength. Determine the ratio of (i) their accelerating potentials and (ii) their speeds. (CBSE Delhi 2015)
Answer:
(i) The de Broglie wavelength is given by λ = \(\frac{h}{\sqrt{2 m q V}}\). Since the de-Broglie waveLength is the same (or proton and alpha particle, we have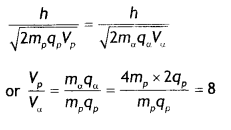

(ii) The de-Broglie wavelength is given by λ = \(\frac{h}{mv}\). Since the de-Broglie wavelength is the same (or proton and alpha particle, we have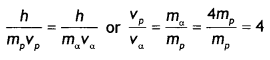

Question 20.
The work function (ω_o), of a metal X, equals 3 × 10^-19 J. Calculate the number (N) of photons, of light of wavelength 26.52 nm, whose total energy equals W. (CBSE Delhi 2016C)
Answer:
Given ωo_o = 3 × 10^-19 J, N = ?, λ = 26.52 nm = 26.52 × 10^-9 m
The energy possessed by one photon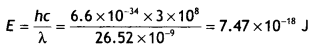

Therefore the number of photons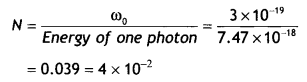

Question 21.
The KE of a beam of electrons accelerated through a potential V, equals the energy of a photon of wavelength 5460 nm. Find the de Broglie wavelength associated with this beam of electrons. (CBSE AI 2016C)
Answer:
The de-Broglie wavelength is given by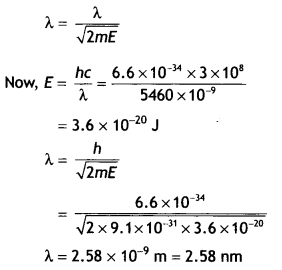

Question 22.
Calculate the kinetic energy of an electron having de Broglie wavelength of 1 A. (CBSE AI 2017C)
Answer:
Using the relation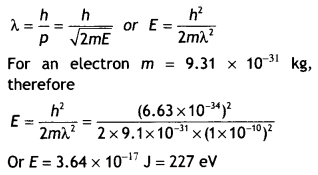

Question 23.
Find the frequency of light that ejects electrons from a metal surface, fully stopped by a retarding potential of 3.3 V. If photoelectric emission begins in this metal at a frequency of 8 × 10¹⁴ Hz, calculate the work function (in eV) for this metal. (CBSE AI 2018C)
Answer:
The work function is given by ω0 = hv0

Question 24.
Monochromatic light of frequency 6.0 × 10¹⁴ Hz is produced by a laser. The power emitted is 2.0 × 10^-3 W. Calculate the (i) energy of a photon in the light beam and
Answer:
Each photon has an energy
E = hv = (6.63 × 10^-34)(6.0 × 10¹⁴)
= 3.98 × 10^-19 J

(ii) a number of photons emitted on an average by the source. (CBSE AI 2018C)
Answer:
If N is the number of photons emitted by the source per second, the power P transmitted in the beam equals N times the energy per photon E so that P = NE. Then
N = \(\frac{P}{E}=\frac{2.0 \times 10^{-3}}{3.98 \times 10^{-19}}\)
= 5.0 × 10¹⁵ photons per second.

Question 25.
The following table gives the values of work function for a few photosensitive metals.

S.No. Metal	Work Function (eV)
1. Na	1.92
2. K	2.15
3. Ca	3.20
4. Mo	4.17

If each of these metals is exposed to radiations of wavelength 300 nm, which of them will not emit photoelectrons and why?
Answer:
The energy of the radiation is
E = \(\frac{h c}{\lambda}=\frac{6.63 \times 10^{-34} \times 3 \times 10^{8}}{300 \times 10^{-9} \times 1.6 \times 10^{-19}}\) = 4.14 eV

This energy is greater than the work functions of Na and K and lesser than the work function of Mo. Hence Mo will not emit photoelectrons.

Question 26.
The work function of caesium is 2.14 eV. Find
(i) the threshold frequency for caesium, and
Answer:
For the cut-off or threshold frequency the energy hv₀ of the incident radiation must be equal to work function Φ₀ so that
v₀ = \(\frac{\phi_{0}}{h}=\frac{2.14 \times 1.6 \times 10^{-19}}{6.63 \times 10^{-34}}\)
= 5.16 × 10¹⁴ Hz
Thus, for frequencies less than this threshold frequency, no photoelectrons are ejected.

(ii) the wavelength of the incident light if the photocurrent is brought to zero by a stopping potential of 0.60 V. (NCERT)
Answer:
Photocurrent reduces to zero when the maximum kinetic energy of the emitted photoelectrons equals the potential energy eV₀ by the retarding potential V₀.
Einstein’s Photoelectric equation is eV₀ = hv – ω₀ = \(\frac{hc}{λ}\) – ω₀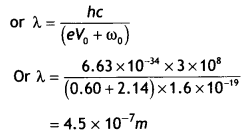

Question 27.
An electron, an a-particle, and a proton have the same kinetic energy. Which of these particles has the shortest de-Broglie wavelength? (NCERT)
Answer:
For a particle, de-Broglie wavelength λ = h/p
Kinetic energy, K = p2/2m.
Then, λ = \(\frac{h}{\sqrt{2 m K}}\)

For the same kinetic energy K, the de-Broglie wavelength associated with the particle is inversely proportional to the square root of their masses. A proton (¹₁H) is 1836 times massive than an electron and a particle (²₄He) four times that of a proton. Hence, a-particle has the shortest de-Broglie wavelength.

Question 28.
A particle is moving three times as fast as an electron. The ratio of the de Broglie wavelength of the particle to that of the electron is 1.813 × 10^-4. Calculate the particle’s mass and identify the particle. (NCERT)
Answer:
the de-Broglie wavelength of a moving particle, having mass m and velocity v
λ = \(\frac{h}{p}=\frac{h}{m v}\)

Mass, m = h/λv
For an electron, mass me = h/λ_e ve
Now, we have v/ve = 3 and λ/λ_e = 1.813 × 10^-4

Then the mass of the particle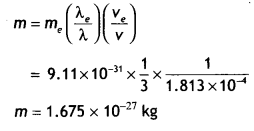

Thus, the particle with this mass could be a proton or a neutron.

Question 29.
What are the (a) momentum, (b) speed, and (c) de Brogue wavelength of an electron with the kinetic energy of 120 eV? (NCERT)
Answer:
Given E = 120 eV, p =?, v =?, λ =?
Using the relation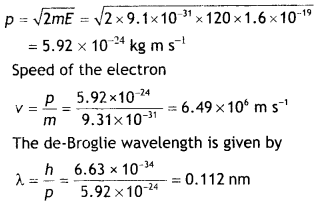

Question 30.
Consider a metal exposed to the light of wavelength 600 nm. The maximum energy of the electron doubles when the light of wavelength 400 nm is used. Find the work function in eV. (NCERT Exemplar)
Answer:
Given λ₁ = 600 nm, E₁ = E, λ₂ = 400 nm,
E₂ = 2E, Φ =?
Now E_max = hv — Φ

According to the question (hc = 1230 eVnm)

Question 31.
A student performs an experiment on the photoelectric effect, using two materials A and B. A plot of vis given In the figure.
(a) Which material A or B has a higher work function?
(b) Given the electric charge of an electron = 1.6 × 10^-19 C, find the value of h obtained from the experiment for both A and B. Comment on whether it is consistent with Einstein’s theory. (NCERT Exemplar)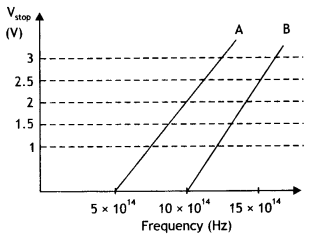

Answer:
(a) The higher the stopping potential, the higher is the work function. The stopping potential of B ís higher than that of A, therefore the work function of B is higher than that of A.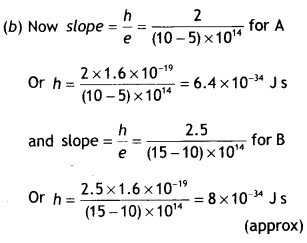

Question 32.
A particle A with a mass m_A is moving with a velocity y and hits a particle B (mass m_B) at rest (one-dimensional motion). Find the change in the de-Broglie wavelength of particle A. Treat the collision as elastic. (NCERT Exemplar)
Answer:
Given U_A = V, U_B = 0
Since the collision is elastic, momentum and kinetic energy wiLt be conserved.