Chapter 9 COUTINUITY

Access RD Sharma Solutions For Class 12 Maths Chapter 9 – Continuity

Exercise 9.1 Page No: 9.16

1. Test the continuity of the following function at the origin:

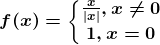
Solution:

Given

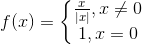
Consider LHL at x = 0

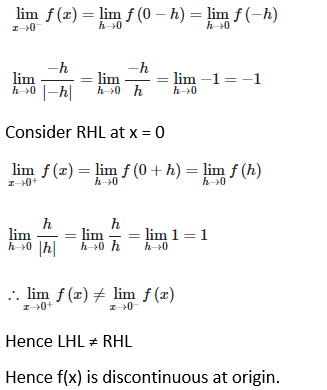
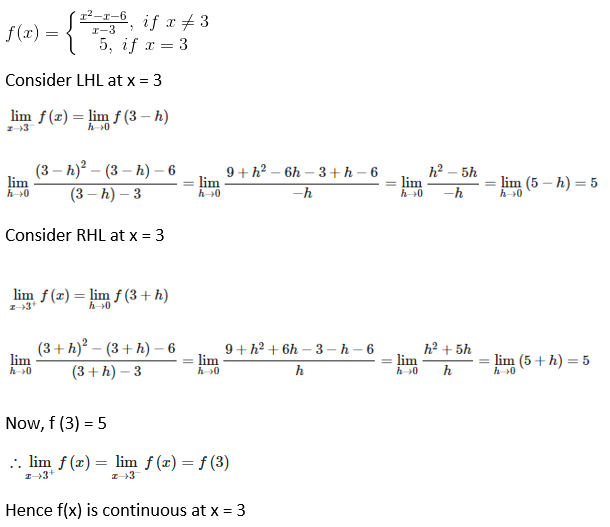
2. A function f(x) is defined as

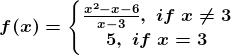
Show that f(x) is continuous at x = 3.

Solution:

Given

3. A function f(x) is defined as

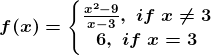
Show that f(x) is continuous at x = 3.

Solution:

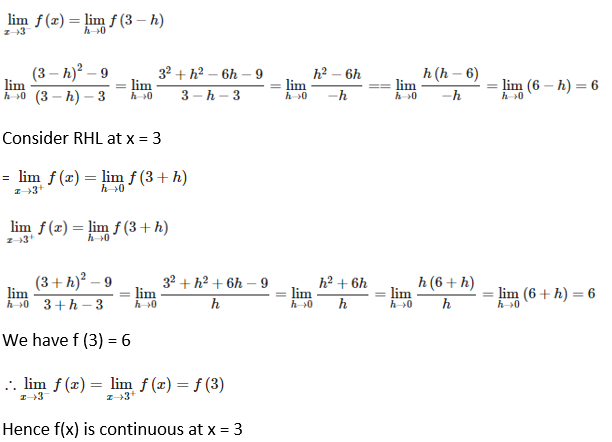
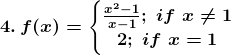
Find whether f(x) is continuous at x = 1.

Solution:

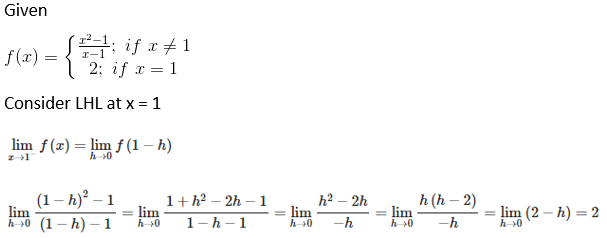
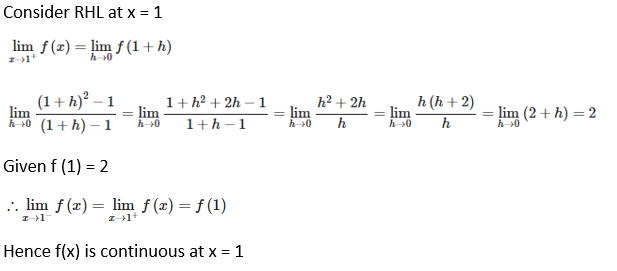
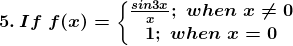
Find whether f(x) is continuous at x = 0.

Solution:

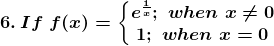
Find whether f(x) is continuous at x = 0.

Solution:

Given

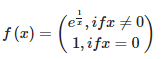
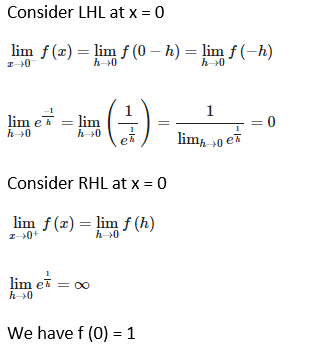
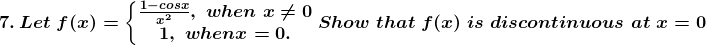
Solution:

Given

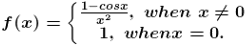
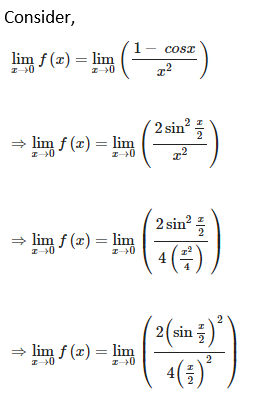
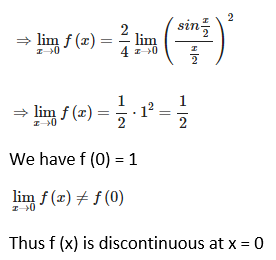
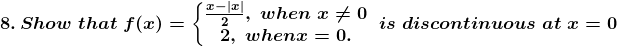
Solution:

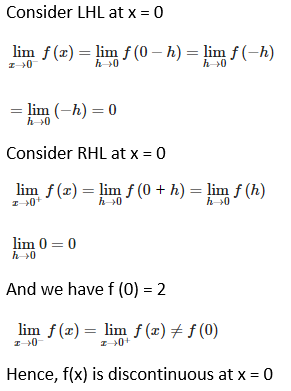
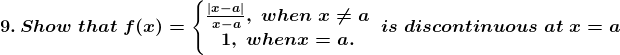
Solution:

10. Discuss the continuity of the following functions at the indicated point(s):

Solution:

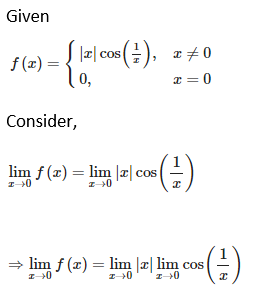
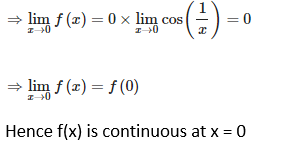
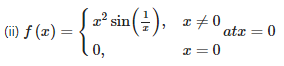
Solution:

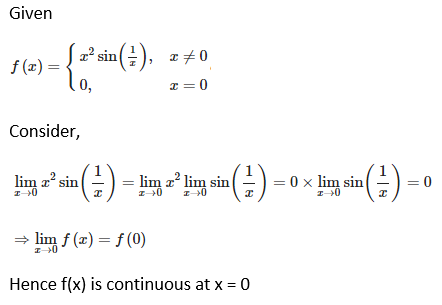
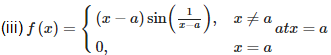
Solution:

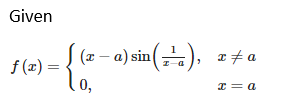
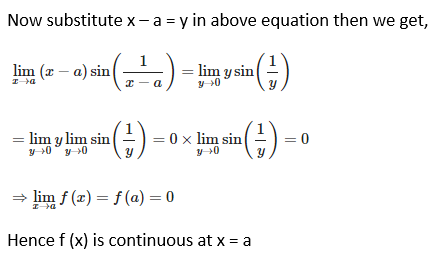
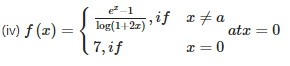
Solution:

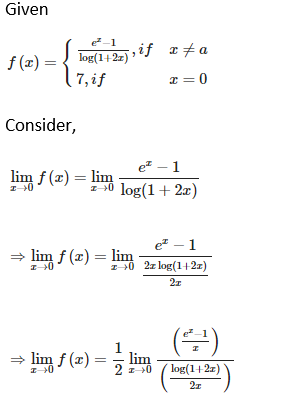
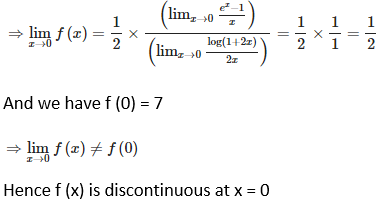
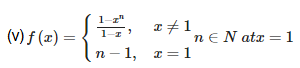
Solution:

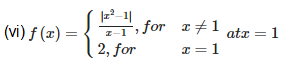
Solution:

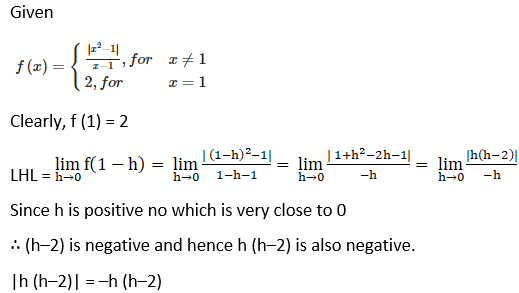
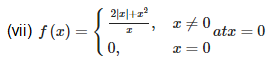
Solution:

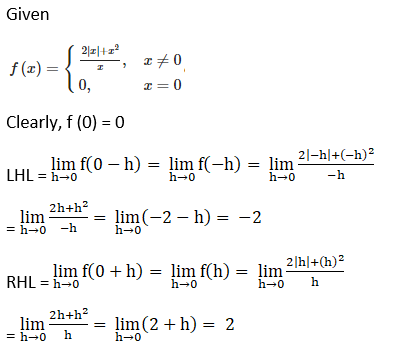
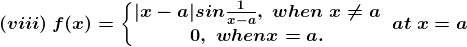
Solution:

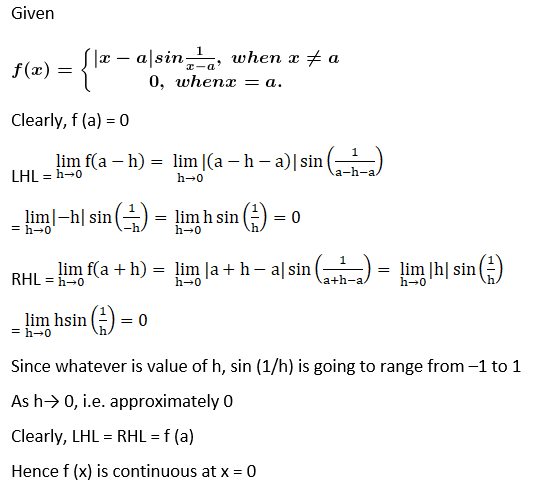
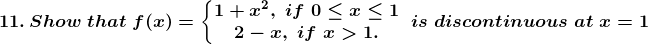
Solution:

Given

Solution:

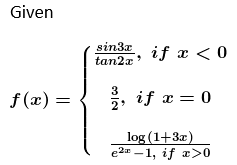
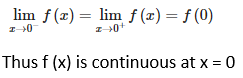
13. Find the value of a for which the function f defined by

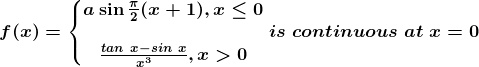
Solution:

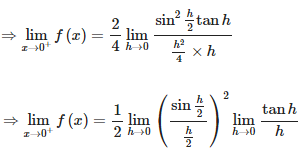
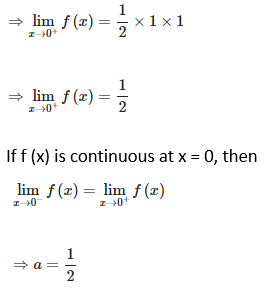
14. Examine the continuity of the function

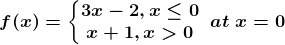
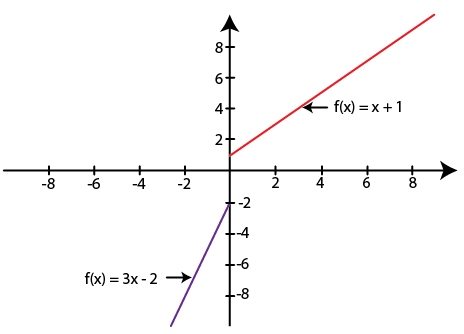
Also sketch the graph of this function.

Solution:

Hence f (x) is discontinuous at x = 0

15. Discuss the continuity of the function

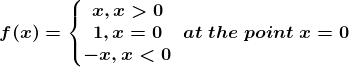
Solution:

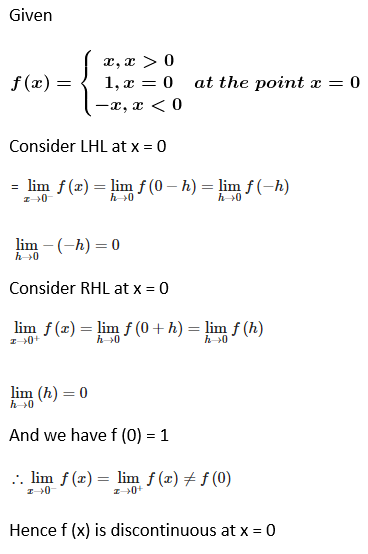
16. Discuss the continuity of the function

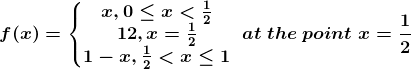
Solution:

17. Discuss the continuity of

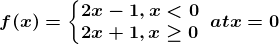
Solution:

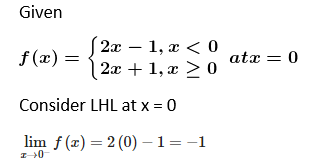
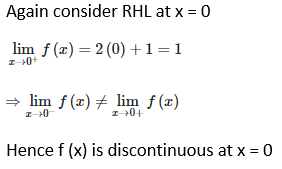
18. For what value of k is the function

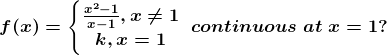
Solution:

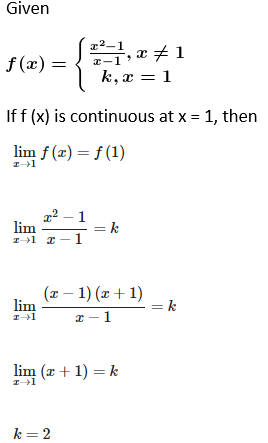
19. Determine the value of the constant k so that the function

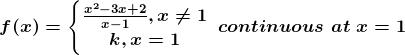
Solution:

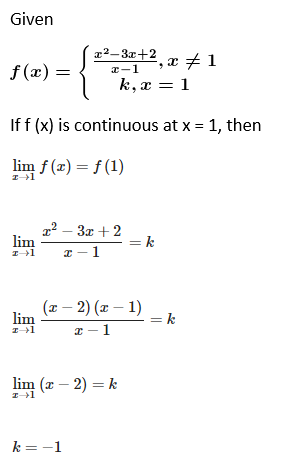
20. For what value of k is the function

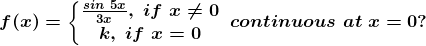
Solution:

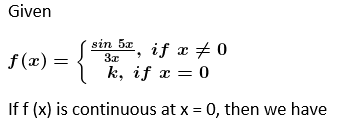
21. Determine the value of the constant k so that the function

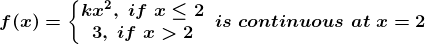
Solution:

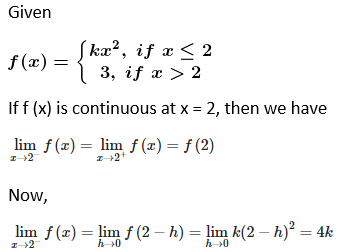
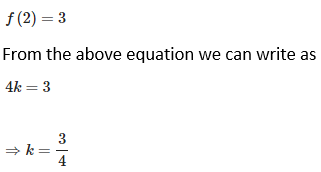
22. Determine the value of the constant k so that the function

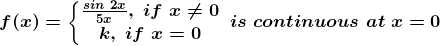
Solution:

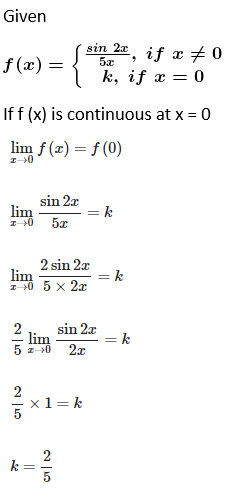
23. Find the values of a so that the function

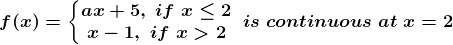
Solution:

Exercise 9.2 Page No: 9.34

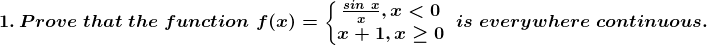
Solution:

A real function f is said to be continuous at x = c, where c is any point in the domain of f if

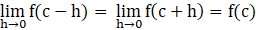
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.

A function is continuous at x = c if

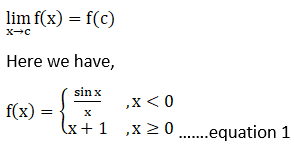
To prove it everywhere continuous we need to show that at every point in the domain of f(x) [domain is nothing but a set of real numbers for which function is defined]

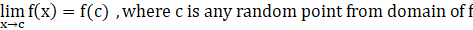
Clearly from definition of f(x), f(x) is defined for all real numbers.

Now we need to check continuity for all real numbers.

Let c is any random number such that c < 0 [thus c being a random number, it can include all negative numbers]

We can say that f(x) is continuous for all x < 0

Now, let m be any random number from the domain of f such that m > 0

Thus m being a random number, it can include all positive numbers]

Therefore we can say that f(x) is continuous for all x > 0

As zero is a point at which function is changing its nature so we need to check LHL, RHL separately

f (0) = 0 + 1 = 1 [using equation 1]

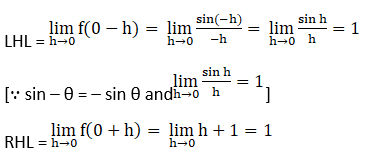
Thus LHL = RHL = f (0).

Therefore f (x) is continuous at x = 0

Hence, we proved that f is continuous for x < 0; x > 0 and x = 0

Thus f(x) is continuous everywhere.

Hence, proved.

Solution:

A real function f is said to be continuous at x = c, where c is any point in the domain of f if

Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.

A function is continuous at x = c if

Here we have,

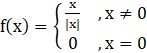 …….equation 1

The function is defined for all real numbers, so we need to comment about its continuity for all numbers in its domain (domain = set of numbers for which f is defined)

Function is changing its nature (or expression) at x = 0, so we need to check its continuity at x = 0 first.

We know that from the definition of mod function we have

f (0) = 0 [using equation 1]

Clearly, LHL ≠ RHL ≠ f (0)

∴ Function is discontinuous at x = 0

Let c be any real number such that c > 0

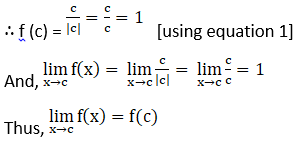
Therefore f (x) is continuous everywhere for x > 0.

Let c be any real number such that c < 0

Therefore f (c) =

[Using equation 1 and idea of mod function]

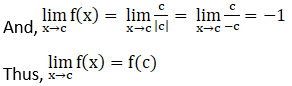
Therefore f (x) is continuous everywhere for x < 0.

Hence, we can conclude by stating that f (x) is continuous for all Real numbers except zero that is discontinuous at x = 0.

3. Find the points of discontinuity, if any, of the following functions:

Solution:

A real function f is said to be continuous at x = c, where c is any point in the domain of f if

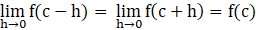
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.

A function is continuous at x = c if

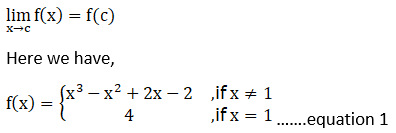
Function is defined for all real numbers so we need to comment about its continuity for all numbers in its domain (domain = set of numbers for which f is defined)

Function is changing its nature (or expression) at x = 1, so we need to check its continuity at x = 1.

Clearly, f (1) = 4 [using equation 1]

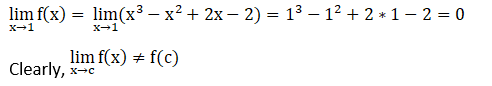
∴ f (x) is discontinuous at x = 1.

Let c be any real number such that c ≠ 0

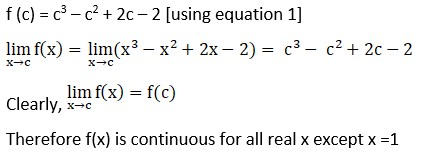
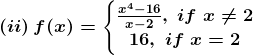
Solution:

A real function f is said to be continuous at x = c, where c is any point in the domain of f if

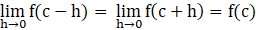
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.

A function is continuous at x = c if

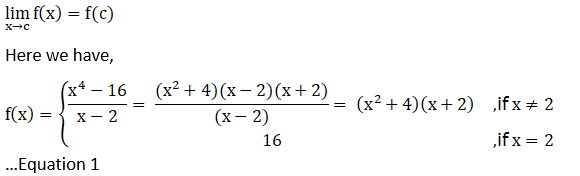
The function is defined for all real numbers, so we need to comment about its continuity for all numbers in its domain (domain = set of numbers for which f is defined)

Function is changing its nature (or expression) at x = 2, so we need to check its continuity at x = 2 first.

Clearly, f (2) = 16 [from equation 1]

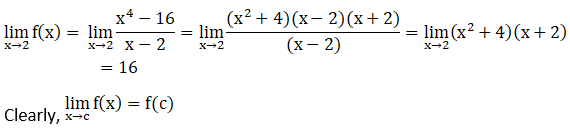
∴ f (x) is continuous at x = 2.

Let c be any real number such that c ≠ 0

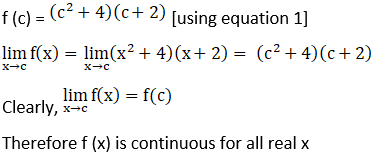
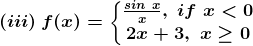
Solution:

A real function f is said to be continuous at x = c, where c is any point in the domain of f if

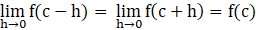
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.

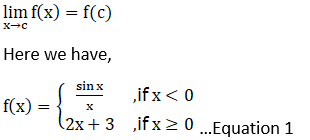
The function is defined for all real numbers, so we need to comment about its continuity for all numbers in its domain (domain = set of numbers for which f is defined)

Let c is any random number such that c < 0 [thus c being a random number, it can include all negative numbers]

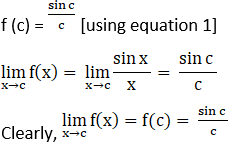
We can say that f(x) is continuous for all x < 0

Now, let m be any random number from the domain of f such that m > 0

Thus m being a random number, it can include all positive numbers]

f (m) = 2m + 3 [from equation 1]

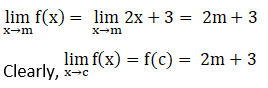
We can say that f(x) is continuous for all x > 0

As zero is a point at which function is changing its nature so we need to check LHL, RHL separately

f (0) = 2 × 0 + 3 = 3 [using equation 1]

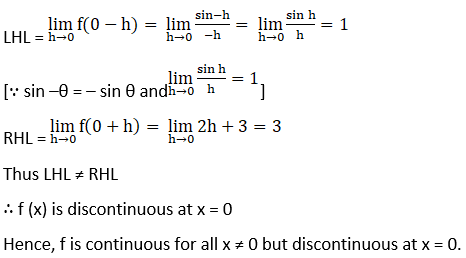
Solution:

A real function f is said to be continuous at x = c, where c is any point in the domain of f if

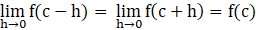
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.

A function is continuous at x = c if

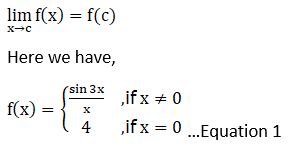
The function is defined for all real numbers, so we need to comment about its continuity for all numbers in its domain (domain = set of numbers for which f is defined)

Let c is any random number such that c ≠ 0 [thus c being a random number, it can include all numbers except 0]

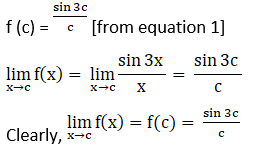
We can say that f(x) is continuous for all x ≠ 0

As zero is a point at which function is changing its nature, so we need to check the continuity here.

f (0) = 4 [using equation 1]

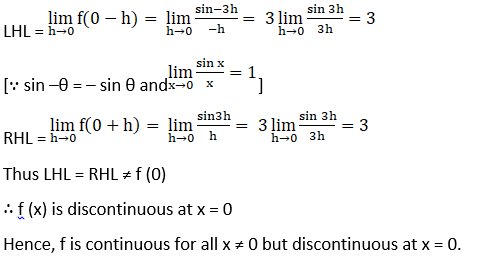
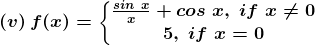
Solution:

A real function f is said to be continuous at x = c, where c is any point in the domain of f if

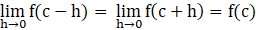
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.

A function is continuous at x = c if

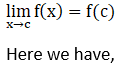
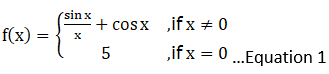
The function is defined for all real numbers, so we need to comment about its continuity for all numbers in its domain (domain = set of numbers for which f is defined)

Let c is any random number such that c ≠ 0 [thus c being a random number, it can include all numbers except 0]

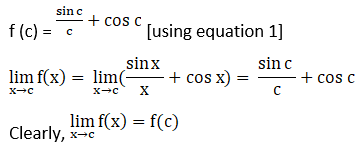
We can say that f(x) is continuous for all x ≠ 0

As zero is a point at which function is changing its nature, so we need to check the continuity here.

f (0) = 5 [using equation 1]

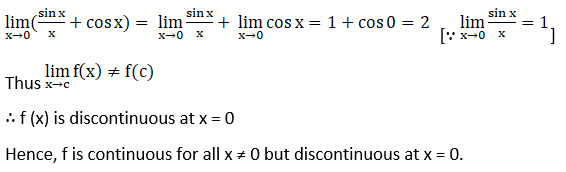
Solution:

A real function f is said to be continuous at x = c, where c is any point in the domain of f if

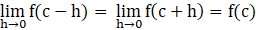
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.

A function is continuous at x = c if

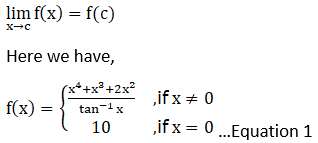
The function is defined for all real numbers, so we need to comment about its continuity for all numbers in its domain (domain = set of numbers for which f is defined)

Let c is any random number such that c ≠ 0 [thus c being a random number, it can include all numbers except 0]

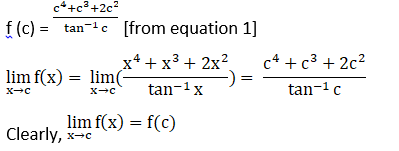
We can say that f(x) is continuous for all x ≠ 0

As zero is a point at which function is changing its nature so we need to check the continuity here.

f (0) = 10 [using equation 1]

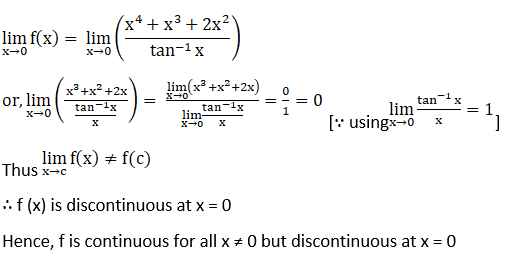
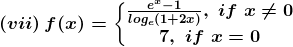
Solution:

A real function f is said to be continuous at x = c, where c is any point in the domain of f if

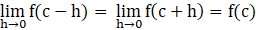
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.

A function is continuous at x = c if

Function is defined for all real numbers so we need to comment about its continuity for all numbers in its domain (domain = set of numbers for which f is defined)

Let c is any random number such that c ≠ 0 [thus c being random number, it is able to include all numbers except 0]

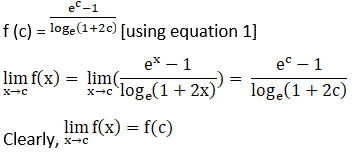
We can say that f(x) is continuous for all x ≠ 0

As x = 0 is a point at which function is changing its nature so we need to check the continuity here.

Since, f (0) = 7 [from equation 1]

Log (1+x) and e^x in its Taylor form.

From sandwich theorem numerator and denominator conditions also hold for this limit

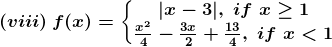
Solution:

A real function f is said to be continuous at x = c, where c is any point in the domain of f if

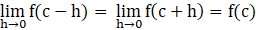
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.

A function is continuous at x = c if

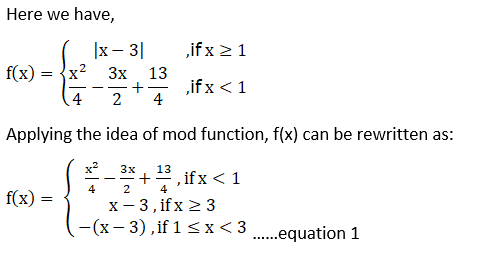
Function is defined for all real numbers so we need to comment about its continuity for all numbers in its domain (domain = set of numbers for which f is defined)

Let c is any random number such that c < 1 [thus c being a random number, it can include all numbers less than 1]

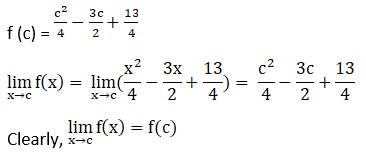
We can say that f(x) is continuous for all x < 1

As x = 1 is a point at which function is changing its nature, so we need to check the continuity here.

f (1) = | 1 – 3 | = 2 [from equation 1]

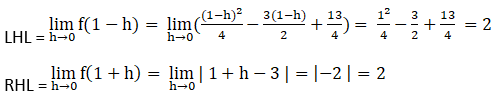
Thus LHL = RHL = f (1)

∴ f (x) is continuous at x = 1

Now, again f(x) is changing its nature at x = 3, so we need to check continuity at x = 3

f (3) = 3 – 3 = 0 [using equation 1]

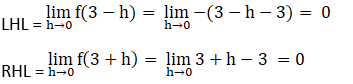
Thus LHL = RHL = f (3)

∴ f (x) is continuous at x = 3

For x > 3; f(x) = x–3 whose plot is linear, so it is continuous for all x > 3

Similarly, for 1 < x < 3, f(x) = 3 – x whose plot is again a straight line and thus continuous for all point in this range.

Hence, f(x) is continuous for all real x.

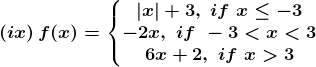
Solution:

A real function f is said to be continuous at x = c, where c is any point in the domain of f if

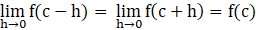
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.

A function is continuous at x = c if

A function is continuous at x = c if

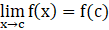
Similarly, we can define it for variable x, if x ≥ 0 |x| = x

If x < 0 |x| = (–x)

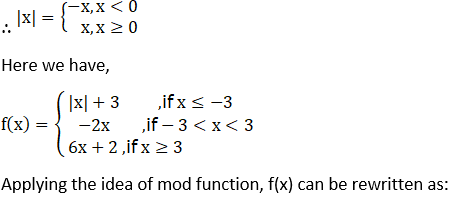
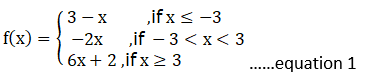
Function is defined for all real numbers so we need to comment about its continuity for all numbers in its domain (domain = set of numbers for which f is defined)

Let c is any random number such that c < –3 [thus c being random number, it is able to include all numbers less than –3]

f (c) = 3 – c [from equation 1]

Thus LHL = RHL = f (–3)

∴ f (x) is continuous at x = –3

Let c is any random number such that –3 < m < 3 [thus c being random number, it is able to include all numbers between –3 and 3]

f (c) = -2m [ using equation 1]

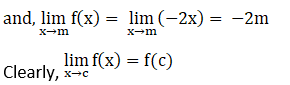
We can say that f(x) is continuous for all –3 < x < 3

Now, again f(x) is changing its nature at x = 3, so we need to check continuity at x = 3

f (3) = 6 × 3 + 2 = 20 [using equation 1]

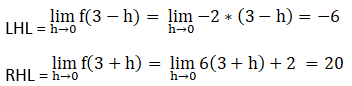
Thus LHL ≠ RHL

∴ f (x) is discontinuous at x = 3

For x > 3; f(x) = 6x + 2 whose plot is linear, so it is continuous for all x > 3

Hence, f(x) is continuous for all real x except x = 3

There is only one point of discontinuity at x = 3

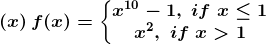
Solution:

A real function f is said to be continuous at x = c, where c is any point in the domain of f if

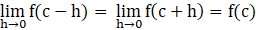
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.

A function is continuous at x = c if

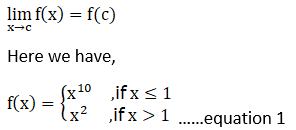
Function is defined for all real numbers so we need to comment about its continuity for all numbers in its domain (domain = set of numbers for which f is defined)

Let c is any random number such that c < 1 [thus c being random number, it is able to include all numbers less than 1]

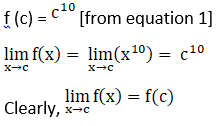
We can say that f(x) is continuous for all x < 1

As x = 1 is a point at which function is changing its nature so we need to check the continuity here.

f (1) = 1¹⁰ = 1 [using equation 1]

Hence, f(x) is continuous for all real x

There no point of discontinuity. It is everywhere continuous

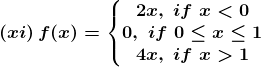
Solution:

A real function f is said to be continuous at x = c, where c is any point in the domain of f if

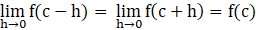
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.

A function is continuous at x = c if

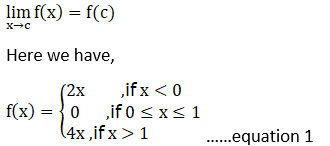
The function is defined for all real numbers, so we need to comment about its continuity for all numbers in its domain (domain = set of numbers for which f is defined)

Let c is any random number such that c < 0 [thus c being a random number, it can include all numbers less than 0]

f (c) = 2c

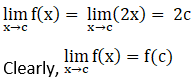
We can say that f(x) is continuous for all x < 0

As x = 0 is a point at which function is changing its nature, so we need to check the continuity here.

f (0) = 0 [using equation 1]

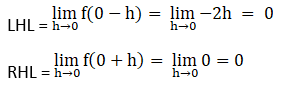
Thus LHL = RHL = f (0)

∴ f (x) is continuous at x = 0

Let m is any random number such that 0 < m < 1 [thus m being a random number, it can include all numbers greater than 0 and less than 1]

f (m) = 0 [using equation 1]

We can say that f(x) is continuous for all 0 < x < 1

As x = 1 is again a point at which function is changing its nature, so we need to check the continuity here.

f (1) = 0

∴ f (x) is discontinuous at x = 1

Let k is any random number such that k > 1 [thus k being a random number, it can include all numbers greater than 1]

f (k) = 4k [using equation 1]

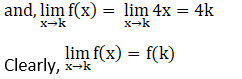
We can say that f(x) is continuous for all x > 1

Hence, f(x) is continuous for all real value of x, except x =1

There is a single point of discontinuity at x = 1

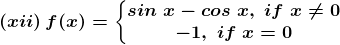
Solution:

A real function f is said to be continuous at x = c, where c is any point in the domain of f if

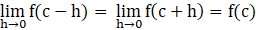
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.

This is very precise, using our fundamental idea of limit from class 11 we can summarise it as, A function is continuous at x = c if

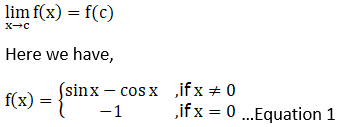
Function is defined for all real numbers so we need to comment about its continuity for all numbers in its domain (domain = set of numbers for which f is defined)

Let c is any random number such that c ≠ 0 [thus c being a random number, it can include all numbers except 0]

f (c) = sin c – cos c [using equation 1]

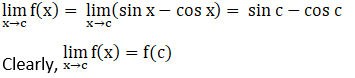
We can say that f(x) is continuous for all x ≠ 0

As zero is a point at which function is changing its nature, so we need to check the continuity here.

f (0) = –1 [using equation 1]

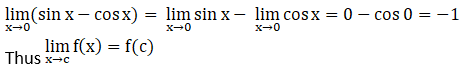
∴ f (x) is continuous at x = 0

Hence, f is continuous for all x.

f (x) is continuous everywhere.

No point of discontinuity.

Solution:

A real function f is said to be continuous at x = c, where c is any point in the domain of f if

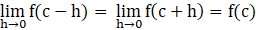
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.

A function is continuous at x = c if

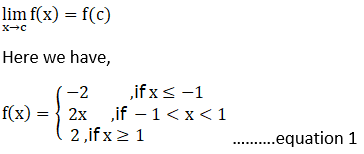
Function is defined for all real numbers so we need to comment about its continuity for all numbers in its domain (domain = set of numbers for which f is defined)

For x < –1, f(x) is having a constant value, so the curve is going to be straight line parallel to x–axis.

So, it is everywhere continuous for x < –1.

Similarly for –1 < x < 1, plot on X–Y plane is a straight line passing through origin.

So, it is everywhere continuous for –1 < x < 1.

And similarly for x > 1, plot is going to be again a straight line parallel to x–axis

∴ it is also everywhere continuous for x > 1

As x = –1 is a point at which function is changing its nature so we need to check the continuity here.

f (–1) = –2

∴ f (x) is continuous at x = –1

Also at x = 1 function is changing its nature so we need to check the continuity here too.

f (1) = 2 [using equation 1]

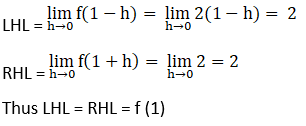
∴ f (x) is continuous at x = 1

Thus, f(x) is continuous everywhere and there is no point of discontinuity.

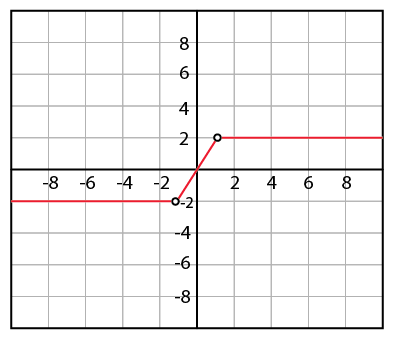
4. In the following, determine the value(s) of constant(s) involved in the definition so that the given function is continuous:

Solution:

A real function f is said to be continuous at x = c, where c is any point in the domain of f if

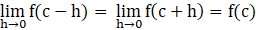
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.

A function is continuous at x = c if

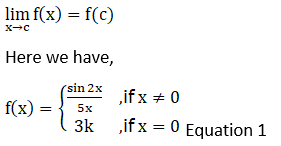
Function is defined for all real numbers and we need to find the value of k so that it is continuous everywhere in its domain (domain = set of numbers for which f is defined)

As, for x ≠ 0 it is just a combination of trigonometric and linear polynomial both of which are continuous everywhere.

As x = 0 is only point at which function is changing its nature so it needs to be continuous here.

f (0) = 3k [using equation 1]

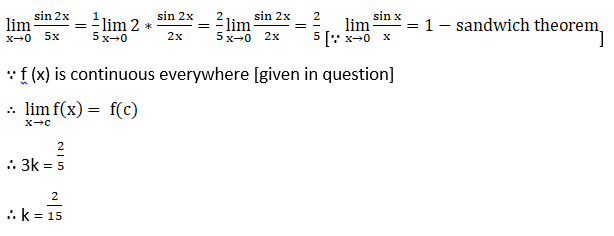
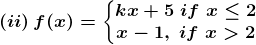
Solution:

A real function f is said to be continuous at x = c, where c is any point in the domain of f if

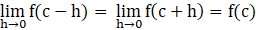
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.

A function is continuous at x = c if

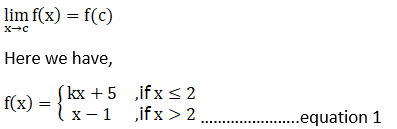
To find the value of constants always try to check continuity at the values of x for which f(x) is changing its expression.

As most of the time discontinuities are here only, if we make the function continuous here, it will automatically become continuous everywhere.

From equation 1, it is clear that f(x) is changing its expression at x = 2

Given, f (x) is continuous everywhere

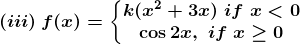
Solution:

A real function f is said to be continuous at x = c, where c is any point in the domain of f if

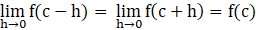
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.

This is very precise, using our fundamental idea of limit from class 11 we can summarise it as, A function is continuous at x = c if

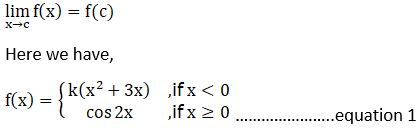
To find the value of constants always try to check continuity at the values of x for which f(x) is changing its expression.

As most of the time discontinuities are here only, if we make the function continuous here, it will automatically become continuous everywhere

From equation 1, it is clear that f(x) is changing its expression at x = 0

Given, f (x) is continuous everywhere

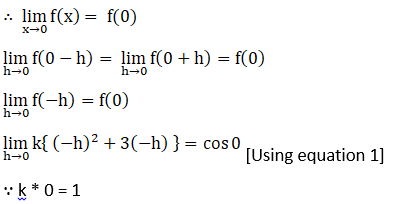
As above equality never holds true for any value of k

k = not defined

No such value of k is possible for which f(x) is continuous everywhere.

f (x) will always have a discontinuity at x = 0

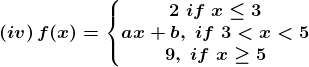
Solution:

A real function f is said to be continuous at x = c, where c is any point in the domain of f if

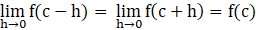
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.

This is very precise, using our fundamental idea of limit from class 11 we can summarise it as, A function is continuous at x = c if

To find the value of constants always try to check continuity at the values of x for which f(x) is changing its expression.

As most of the time discontinuities are here only, if we make the function continuous here, it will automatically become continuous everywhere

From equation 1, it is clear that f(x) is changing its expression at x = 3

Given, f(x) is continuous everywhere

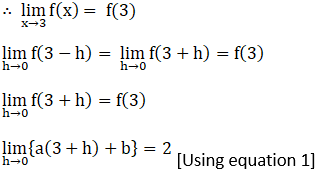
∴ 3a + b = 2 ……………….Equation 2

Also from equation 1, it is clear that f(x) is also changing its expression at x = 5

Given, f(x) is continuous everywhere

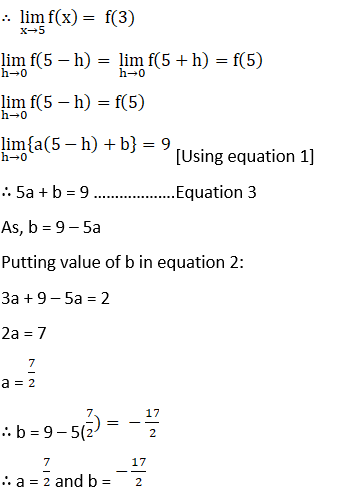
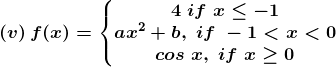
Solution:

A real function f is said to be continuous at x = c, where c is any point in the domain of f if

Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.

A function is continuous at x = c if

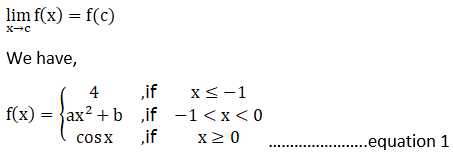
To find the value of constants always try to check continuity at the values of x for which f(x) is changing its expression.

As most of the time discontinuities are here only, if we make the function continuous here, it will automatically become continuous everywhere

From equation 1, it is clear that f(x) is changing its expression at x = –1

Given, f(x) is continuous everywhere

∴ a + b = 4 ……………….Equation 2

Also from equation 1, it is clear that f(x) is also changing its expression at x = 0

Given, f (x) is continuous everywhere

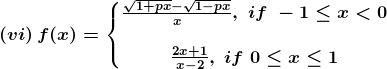
Solution:

A real function f is said to be continuous at x = c, where c is any point in the domain of f if

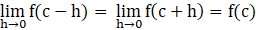
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.

A function is continuous at x = c if

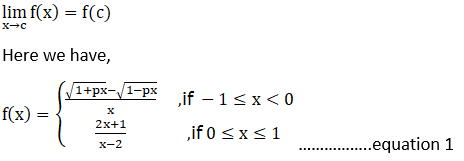
To find the value of constants always try to check continuity at the values of x for which f(x) is changing its expression.

As most of the time discontinuities are here only, if we make the function continuous here, it will automatically become continuous everywhere

From equation 1, it is clear that f(x) is changing its expression at x = 0

Given, f(x) is continuous everywhere

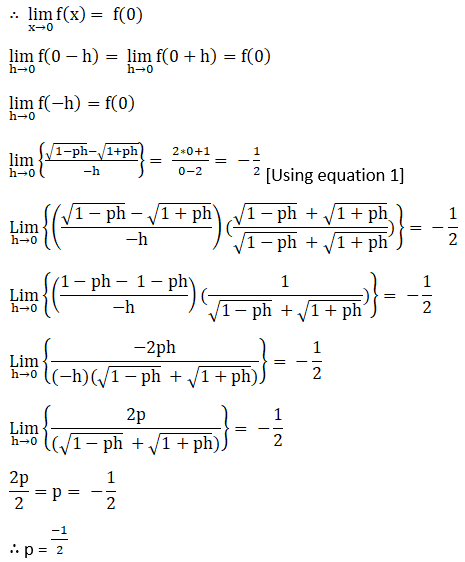
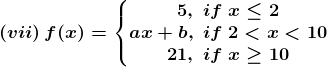
Solution:

A real function f is said to be continuous at x = c, where c is any point in the domain of f if

Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.

A function is continuous at x = c if

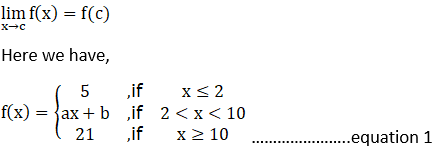
To find the value of constants always try to check continuity at the values of x for which f(x) is changing its expression.

As most of the time discontinuities are here only, if we make the function continuous here, it will automatically become continuous everywhere

From equation 1, it is clear that f(x) is changing its expression at x = 2

Given, f(x) is continuous everywhere

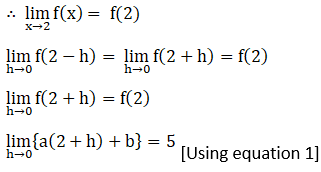
∴ 2a + b = 5 ……………….Equation 2

Also from equation 1, it is clear that f(x) is also changing its expression at x = 10

Given, f(x) is continuous everywhere

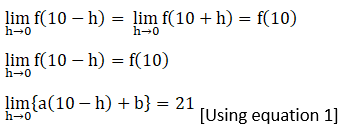
∴ 10a + b = 21 ……………….Equation 3

As, b = 21 – 10a

Putting value of b in equation 2, we get

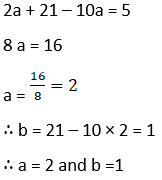
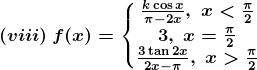
Solution:

A real function f is said to be continuous at x = c, where c is any point in the domain of f if

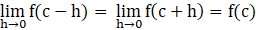
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.

A function is continuous at x = c if

To find the value of constants always try to check continuity at the values of x for which f(x) is changing its expression.

As most of the time discontinuities are here only, if we make the function continuous here, it will automatically become continuous everywhere

From equation 1, it is clear that f(x) is changing its expression at x = π/2

Given, f(x) is continuous everywhere

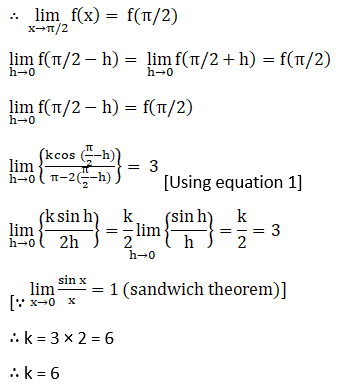
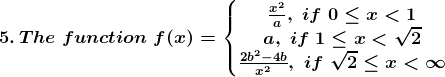
Is continuous on [0, ∞). Find the most suitable values of a and b.

Solution:

A real function f is said to be continuous at x = c, where c is any point in the domain of f if

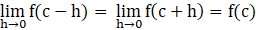
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.

A function is continuous at x = c if

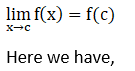
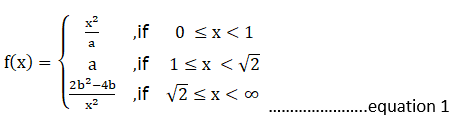
The function is defined for [0, ∞) and we need to find the value of a and b so that it is continuous everywhere in its domain (domain = set of numbers for which f is defined)

To find the value of constants always try to check continuity at the values of x for which f(x) is changing its expression.

As most of the time discontinuities are here only, if we make the function continuous here, it will automatically become continuous everywhere

From equation 1, it is clear that f(x) is changing its expression at x = 1

Given, f (x) is continuous everywhere

Also from equation 1, it is clear that f(x) is also changing its expression at x = √2

Given, f (x) is continuous everywhere

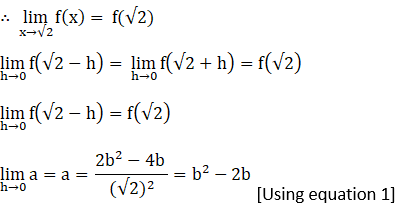
∴ b² – 2b = a ……………….Equation 3

From equation 2, a = –1

b² – 2b = –1

⇒ b² – 2b + 1 = 0

⇒ (b – 1)² = 0

∴ b = 1 when a = –1

Putting a = 1 in equation 3:

b² – 2b = 1

⇒ b² – 2b – 1 = 0

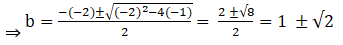
Thus,

For a = –1; b = 1

For a = 1; b = 1 ± √2

6. Find the values of a and b so that the function f (x) defined by

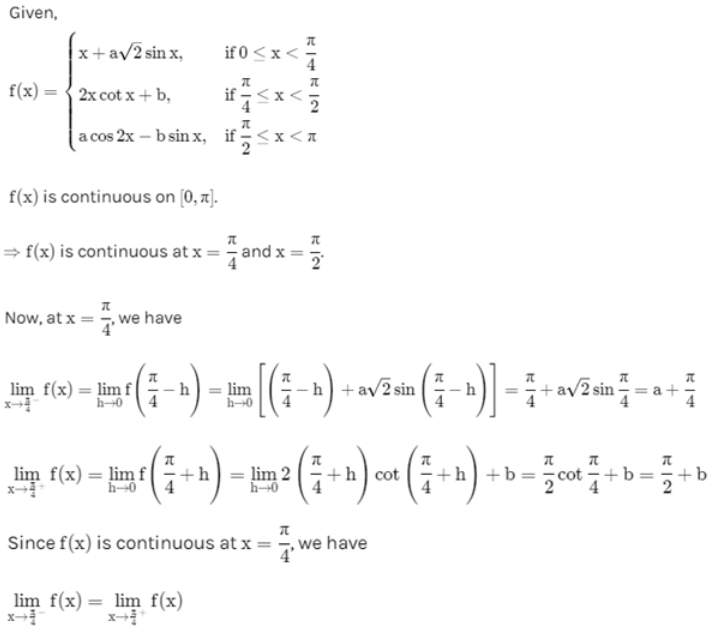
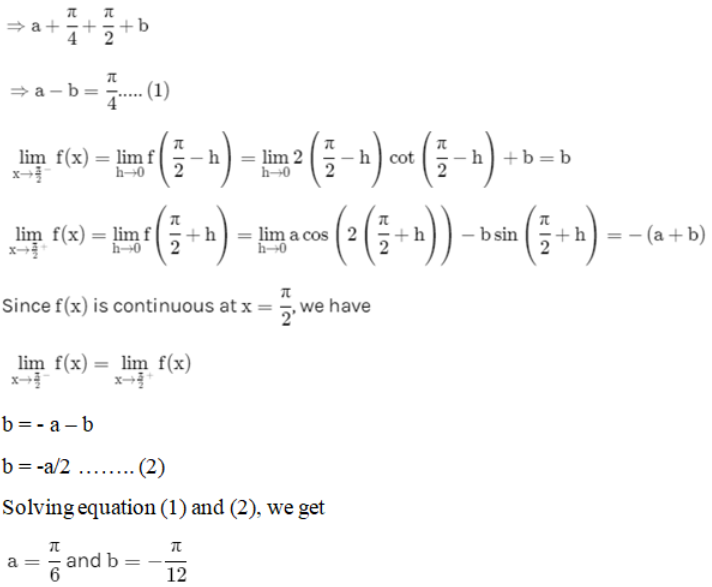
If f is continuous on [0, 8], find the values of a and b.

Solution:

A real function f is said to be continuous at x = c, where c is any point in the domain of f if

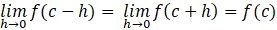
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.

A function is continuous at x = c if

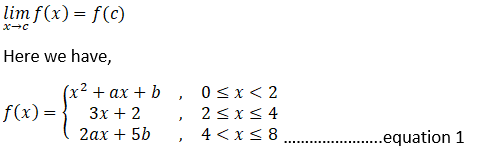
Function is defined for [0, 8] and we need to find the value of a and b so that it is continuous everywhere in its domain (domain = set of numbers for which f is defined)

To find the value of constants always try to check continuity at the values of x for which f(x) is changing its expression.

As most of the time discontinuities are here only, if we make the function continuous here, it will automatically become continuous everywhere

From equation 1, it is clear that f(x) is changing its expression at x = 2

Given, f (x) is continuous everywhere

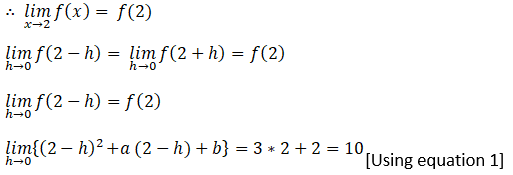
4+2a + b = 8

∴ 2a + b = 4

∴ b = 4 – 2a …………… equation 2

Also from equation 1, it is clear that f(x) is also changing its expression at x = 4

Given, f (x) is continuous everywhere

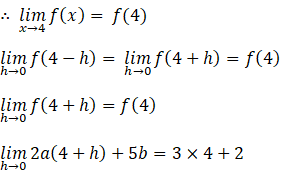
∴ 8a + 5b = 14 ……………….Equation 3

Putting value of a from equation 2 to equation 3

∴ 8a + 5(4–2a) = 14

⇒ 2a = 6

∴ a = 6/2

= 3

∴ b = 4 – 2×3 = –2

Thus, a = 3 and b = –2

8. If 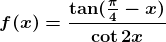for x ≠ π/4, find the value which can be assigned to f (x) at x = π/4 so that the function f (x) becomes continuous everywhere in [0, π/2].

Solution:

A real function f is said to be continuous at x = c, where c is any point in the domain of f if

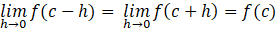
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.

A function is continuous at x = c if

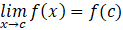
Function is defined for [0, π] and we need to find the value of f(x) so that it is continuous everywhere in its domain (domain = set of numbers for which f is defined)

As we have expression for x ≠ π/4, which is continuous everywhere in [0, π], so

If we make it continuous at x = π/4 it is continuous everywhere in its domain.

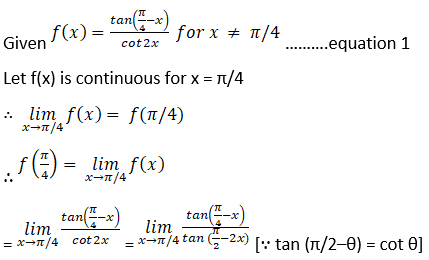
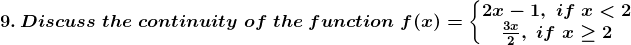
Solution:

A real function f is said to be continuous at x = c, where c is any point in the domain of f if

Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.

A function is continuous at x = c if

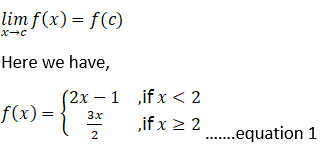
Function is changing its nature (or expression) at x = 2, so we need to check its continuity at x = 2 first.

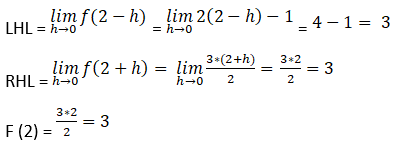
Clearly, LHL = RHL = f (2)

∴ Function is continuous at x = 2

Let c be any real number such that c > 2

∴ f (x) is continuous everywhere for x > 2.

Let m be any real number such that m < 2

∴ f (m) = 2m – 1 [using equation 1]

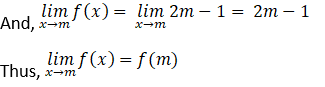
∴ f (x) is continuous everywhere for x < 2.

Hence, we can conclude by stating that f(x) is continuous for all Real numbers