Chapter 27 DIRECTION COSINES AND DIRECTION

Access Answers to RD Sharma Solution Class 12 Maths Chapter 27

EXERCISE 27.1

Q1.

Solution:

Let us consider l, m, and n to be the direction cosines of a line.

So,

l = cos 90^o = 0

m = cos 60^o = ½

n = cos 30^o = √3/2

Hence,

The direction cosines of the line are 0, ½, √3/2

Q2.

Solution:

Let us consider l, m, and n to be the direction cosines of a line.

Then,

a = 2, b = -1, c = -2 will be the direction ratios of the line.

So by using the formula,

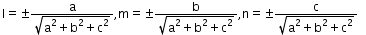
We get,

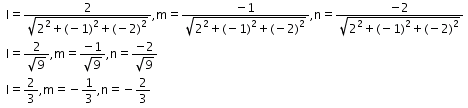
Hence,

The direction ratios of the line are 2/3, -1/3, -2/3

Q3.

Solution:

We know that the direction ratios of the line joining (-2, 4, -5) and (1, 2, 3) are

(1+2, 2-4, 3+5) = (3, -2, 8)

Then,

a = 3, b = -2, c = 8

So let us find the direction cosines:

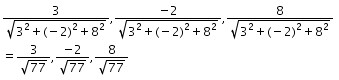
Hence,

The direction cosines are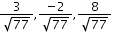

Q4.

Solution:

We know that,

A(2, 3, -4), B(1, -2, 3) and C(3, 8, -11)

So,

The direction ratios of AB = (1-2, -2-3, 3+4) = (-1, -5, 7)

The direction ratios of BC = (3-1, 8+2, -11-3) = (2, 10, -14)

Then,

The direction cosines of AB and AC

-1/2 = -5/10 = 7/-14 are proportional.

We also know that,

B is a common point between two lines,

Hence, the points A(2, 3, -4), B(1, -2, 3) and C(3, 8, -11) are collinear.

Q5.

Solution:

We know that,

A(3, 5, -4), B(-1, 1, 2) and C(-5, -5, -2)

So,

The direction ratios of side AB = (-1-3, 1-5, 2+4) = (-4, -4, 6)

So,

The direction cosines of AB is

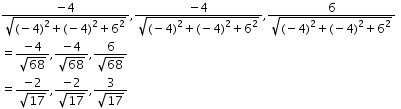
Now,

The direction ratios of side BC = (-5+1, -5-1, -2-2) = (-4, -6, -4)

So,

The direction cosines of BC is

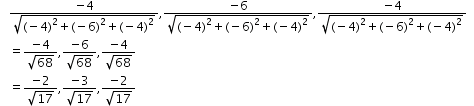
Now,

The direction ratios of side AC = (-5-3, -5-5, -2+4) = (-8, -10, 2)

So,

The direction cosines of AC is