Chapter 21 AREAS OF BOUNDED REGIONS

Access RD Sharma Solutions for Class 12 Maths Chapter 21 Exercise 1

EXERCISE 21.1

Question. 1

Solution:

From the question, it is given that two equations,

x = 2 … [equation (i)]

y² = 8x … [equation (ii)]

So, equation (i) represents a line parallel to the y-axis and equation (ii) represents a parabola with vertex at the origin and the x-axis as it axis, as shown in the rough sketch below,

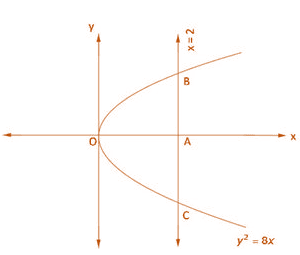
Now, we have to find the area of OCBO,

Then, the area can be found by taking a small slice in each region of width Δx,

And length = (y – 0) = y

The area of the sliced part will be as it is a rectangle = y Δx

So, this rectangle can move horizontal from x = 0 to x = 2

The required area of the region bounded between the lines = Region OCBO

= 2 (region OABO)

Now, applying limits we get,

= 4√2[((2/3)2√2) – ((2/3)0√0)]

= 4√2(4√2/3)

Therefore, the required area = 32/3 square units.

Question. 2

Solution:

From the question, it is given that equation,

y – 1 = x … [equation (i)]

So, equation (i) represents a line that meets at (0, 1) and (-1, 0), is as shown in the rough sketch below,

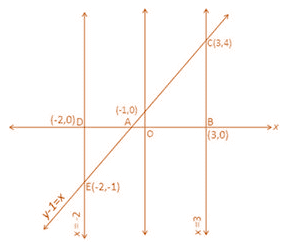
Now, we have to find the area of the region bounded by the line y – 1 = x,

So, Required area = Region ABCA + Region ADEA

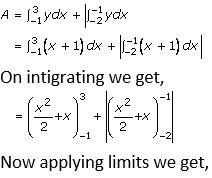
= [((9/2) + 3) – ((½) – 1)] + [((½) – 1) – (2 – 2)]

= [(15/2) + (½)] + [-½]

On simplification, we get,

= 8 + ½

A = 17/2 square units

Therefore, the area of the region bounded by the line y – 1 = x is 17/2 square units.

Question. 3

Solution:

From the question, it is given that two equations,

x = a … [equation (i)]

y² = 4ax … [equation (ii)]

So, equation (i) represents a line parallel to the y-axis and equation (ii) represents a parabola with vertex at the origin and the x-axis as it axis, as shown in the rough sketch below,

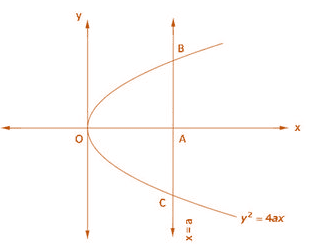
Now, we have to find the area of OCBO,

Then, the area can be found by taking a small slice in each region of width Δx,

And length = (y – 0) = y

The area of the sliced part will be as it is a rectangle = y Δx

So, this rectangle can move horizontally from x = 0 to x = a

The required area of the region bounded between the lines = Region OCBO

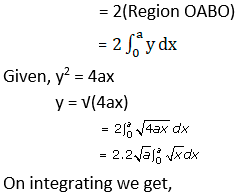
Question. 4

Solution:

From the question, it is given that equation,

y = 4x – x²

Adding 4 on both sides,

y + 4 = 4x – x² + 4

Transposing we get,

x² – 4x + 4 = – y + 4

We know that (a – b)² = a² – 2ab + b²

(x – 2)² = – (y – 4) … [equation (i)]

So, equation (i) represents a downward parabola with vertex (2, 4) and passing through (0, 0) and (0, 4), is as shown in the rough sketch below,

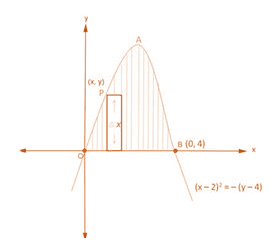
Then, the area can be found by taking a small slice in each region of width Δx,

And length = (y – 0) = y

The area of the sliced part will be as it is a rectangle = y Δx

So, this rectangle can move horizontally from x = 0 to x = a

The required area of the region bounded between the lines = Region OABO

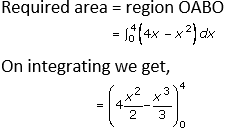
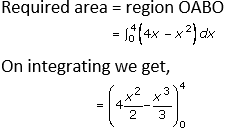
Now we have to apply limits,

= [((4 × 16)/2) – (64/3)] – [0 – 0]

On simplification, we get,

= 64/6

Dividing both the numerator and denominator by 2 we get,

= 32/3

Therefore, the required area is 32/3 square units.

Question. 5

Solution:

From the question, it is given that two equations,

y² = 4x … [equation (i)]

x = 3 … [equation (ii)]

So, equation (i) represents a parabola with vertex at the origin and the x-axis as its axis and equation (ii) represents a line parallel to the y-axis, as shown in the rough sketch below,

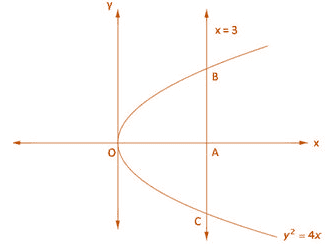
Now, we have to find the area of OCBO,

Then, the area can be found by taking a small slice in each region of width Δx,

And length = (y – 0) = y

The area of the sliced part will be as it is a rectangle = y Δx

So, this rectangle can move horizontal from x = 0 to x = 3

The required area of the region bounded between the lines = Region OCBO

= 2 (region OABO)

Access RD Sharma Solutions for Class 12 Maths Chapter 21 Exercise 2

EXERCISE 21.2

Question. 1

Solution:

From the question, it is given that,

Lines, x = 0, y = 1, y = 4

Parabola y = 4x² … [equation (i)]

So, equation (i) represents a parabola with vertex (0, 0) and axis as the y-axis. x = 0 is the y-axis, and y = 1, y = 4 are lines parallel to x – axis passing through (0, 1) and (0, 4), respectively, as shown in the rough sketch below,

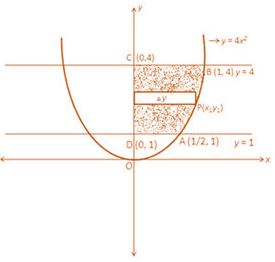
Now, we have to find the area of ABCDA,

Then, the area can be found by taking a small slice in each region of width Δy,

And length = x

The area of the sliced part will be as it is a rectangle = x Δy

So, this rectangle can move horizontally from y = 1 to x = 4

The required area of the region bounded between the lines = Region ABCDA

Now, applying limits, we get,

= ½ [((2/3) × 4 × √4) – ((2/3) × 1 × √1)]

= ½ [(16/3) – (2/3)]

= ½ [(16 – 2)/3]

= ½ [14/3]

= 7/3

Therefore, the required area is 7/3 square units.

Question. 2

Solution:

From the question, it is given that,

Region in first quadrant bounded by y = 1, y = 4

Parabola x² = 16y … [equation (i)]

So, equation (i) represents a parabola with vertex (0, 0) and axis as y-axis, as shown in the rough sketch below,

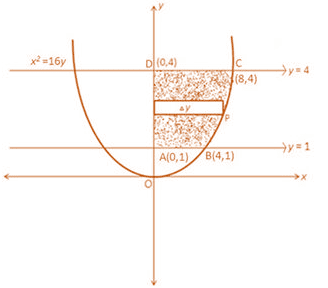
Now, we have to find the area of ABCDA,

Then, the area can be found by taking a small slice in each region of width Δy,

And length = x

The area of the sliced part will be as it is a rectangle = x Δy

So, this rectangle can move horizontally from y = 1 to x = 4

The required area of the region bounded between the lines = Region ABCDA

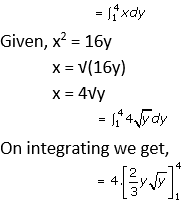
Now, applying limits, we get,

= 4 [((2/3) × 4 × √4) – ((2/3) × 1 × √1)]

= 4 [(16/3) – (2/3)]

= 4 [(16 – 2)/3]

= 4 [14/3]

= 56/3

Therefore, the required area is 56/3 square units.

Question. 3

Solution:

We have to find the area of the region bounded by x² = 4ay

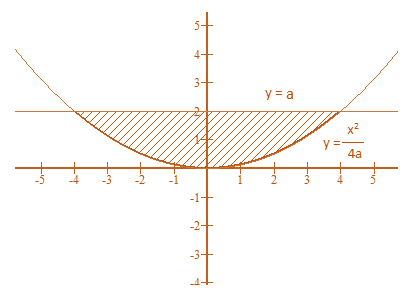
Now applying limits,

= 2 × [(a (2a – 0)) – ((2a)³ – 0³)/12a)]

= 2 × [(2a²) – (8a³/12a)]

= 2 × [(24a³ – 8a³)/12a]

= 2 × [16a³/12a]

= 2 × [4a²/3]

= 8a²/3

Therefore, the area of the region is 8a²/3 square units.

Question. 4

Solution:

We have to find the area of the region bounded by x² + 16y = 0

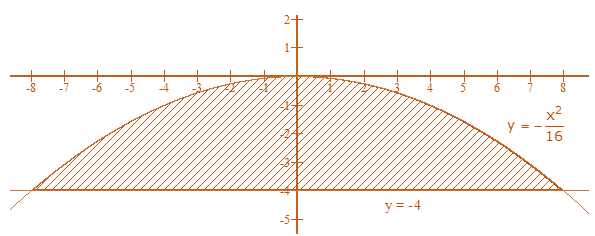
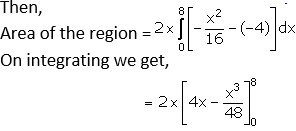
Now applying limits,

= 2 × [(4(8 – 0)) – ((8)³ – 0³)/48)]

= 2 × [(32) – (512/48)]

= 2 × [(32) – (32/3)]

= 2 × [(96 – 32)/3]

= 2 × [64/3]

= 128/3

Therefore, the area of the region is 128/3 square units.

Question. 5

Solution:

We have to find the area of the region bounded by curve ay² = x³, and lines y = a and y = 2a.

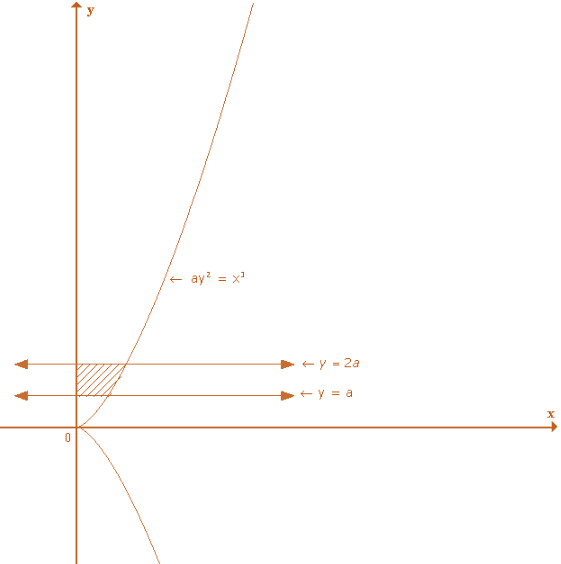
Access RD Sharma Solutions for Class 12 Maths Chapter 21 Exercise 3

EXERCISE 21.3

Question. 1

Solution:

From the question, it is given that the area of the region common to the parabolas y² = 6x and x² = 6y,

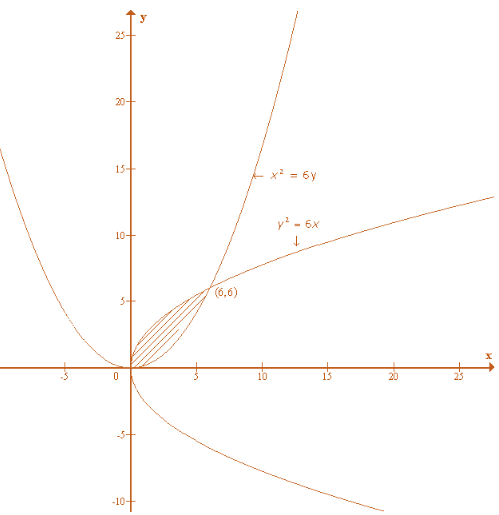
Given, y² = 6x

y = √(6x)

and x² = 6y, then y = x²/6

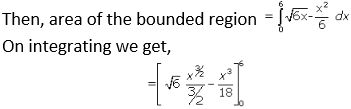
Now applying limits, we get,

= [√6 ((6)^3/2/(3/2) – (6³/18) – 0]

By simplification, we get,

= 12 square units

Question. 2

Solution:

From the question, it is given that the area of the region common to the parabolas 4y² = 9x and 3x² = 16y,

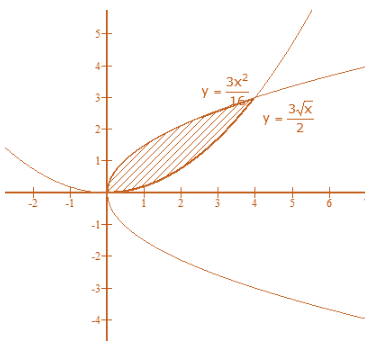
Given, 4y² = 9x

y² = 9x/4

y = √(9x/4)

y = (3/2) √x

and 3x² = 16y

y = 3x²/16

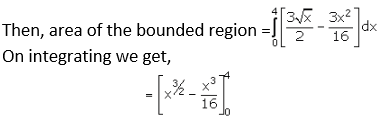
Now applying limits, we get,

= [(4)^3/2 – (4³/16)]

By simplification, we get,

= [(8) – (64/16)]

= [8 – 4]

= 4 square units

Question. 3

Solution:

From the question, it is given that the area of the region bounded by,

y = √x

y² = x … [equation (i)]

y = x … [equation (ii)]

So, equation (i) represents a parabola with vertex at (0, 0) and x-axis as its axis and equation (ii) represents a line passing through the origin and intersecting parabola at (0, 0) and (1, 1), is as shown in the rough sketch below,

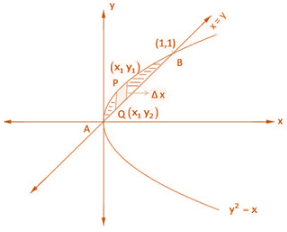
Now, we have to find the area of AOBPA,

Then, the area can be found by taking a small slice in each region of width Δx,

And length = (y₁ – y₂)

The area of the sliced part will be as it is a rectangle = (y₁ – y₂) Δx

So, this rectangle can move horizontal from x = 0 to x = 1

The required area of the region bounded between the lines = Region AOBPA

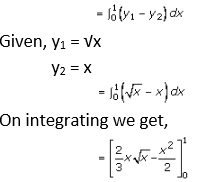
Now, applying limits, we get,

= [((2/3) × 1 × √1) – ((1)²/2)] – [0]

= [(2/3) – ½]

= [(4 – 3)/6]

= 1/6

Therefore, the required area = 1/6 square units.

Question. 4

Solution:

From the question, it is given that the area bounded by the curve y = 4 – x²

And line y = 0, y = 3

So, y = 4 – x²

x² = – (y – 4) … [equation (i)]

y = 0 … [equation (ii)]

y = 3 … [equation (iii)]

So, equation (i) represents a parabola with vertex at (0, 4) and passes through (0, 2), (0, -2) on the x-axis and equation (ii) is the x-axis and cutting the parabola at (2, 0) and (-2, 0). Equation (iii) represents a line parallel to the x-axis passing through (0, 3), as shown in the rough sketch below,

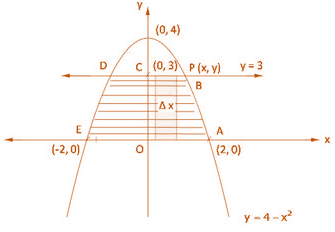
Now, we have to find the area of AOBPA,

Then, the area can be found by taking a small slice in each region of width Δx,

And length = (y – 0) = y

The area of the sliced part will be as it is a rectangle = (y₁ – y₂) Δx

So, this rectangle can move horizontal from x = 0 to x = 2

The required area of the region bounded between the lines = Region ABDEA

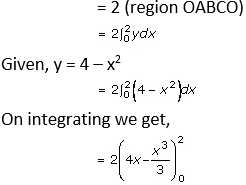
Now, applying the limits, we get,

= 2 [(8 – (8/3)) – 0]

= 2 [(24 – 8)/3]

= 2 [16/3]

= 32/3

Therefore, the required area 32/3 square units.

Question. 5

Solution:

From the question, it is given that,

(x²/a²) + (y²/b²) = 1 … [equation (i)]

(x/a) + (y/b) = 1 … [equation (ii)]

So, equation (i) represents an ellipse with centre at origin and passing through (±a, 0), (0, ±b), and equation (ii) represents a line passing through (a, 0) and (0, b), as shown in the rough sketch below,

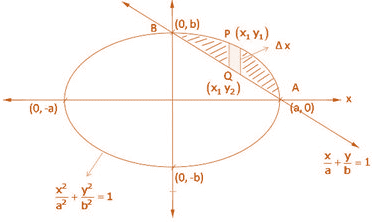
Then, from the figure, the region shaded is the required region and substituting (0, 0) in x²/a² + y²/b² ≤ 1 gives a true statement and substituting (0, 0) in 1 ≤ x/a + y/b gives a false statement.

Then, the area can be found by taking a small slice in each region of width Δx,

And length = (y – 0) = y

The area of the sliced part will be as it is a rectangle = (y₁ – y₂) Δx

So, this rectangle can move horizontal from x = 0 to x = a,

Access RD Sharma Solutions for Class 12 Maths Chapter 21 Exercise 4

EXERCISE 21.4

Question. 1

Solution:

From the question it is given that, parabola x = 4y – y² and the line x = 2y – 3,

As shown in the figure,

x₁ = 4y – y²

x₂ = 2y – 3

So,

2y – 3 = 4y – y²

y² + 2y – 4y – 3 = 0

y² – 2y – 3 = 0

y² – 3y + y – 3 = 0

y(y – 3) + 1(y – 3) = 0

(y – 3) (y + 1) = 0

y = -1, 3

Now, we have to find the area of the bounded region,

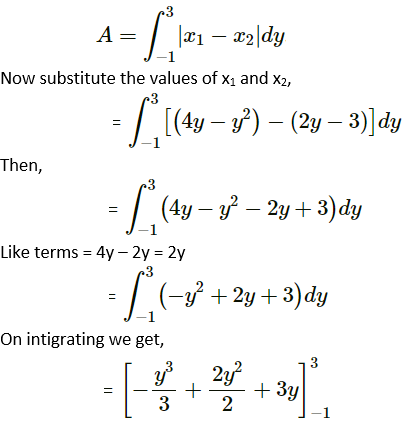
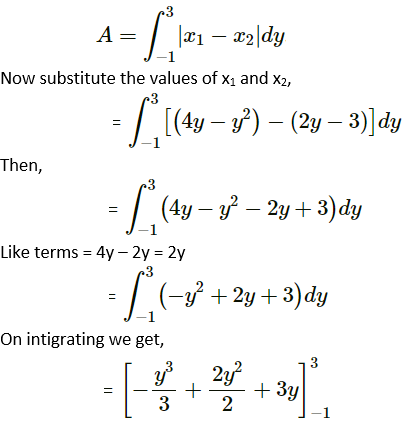
Applying limits, we get,

= [- (3³/3) + 2(3²)/2 + 3(3)] – [- ((-1)³/3) + 2(-1²)/2 + 3(-1)]

= [- 3² + 3² + 9] – [(1/3) + 1 – 3]

= [9] – [(1/3) – 1 + 3]

= 9 – (1/3) + 2

= 11 – (1/3)

= (33 – 1)/3

= 32/3 square units

Therefore, the required area is 32/3 square units.

Question. 2

Solution:

From the question it is given that, parabola x = 8 + 2y – y² and the line y = – 1, y = 3

As shown in the figure,

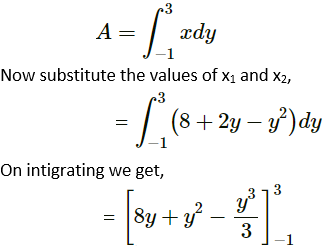
Applying limits, we get,

= [8(3) + (3²) – (3)³/3] – [8(-1) + (-1²) – (-1)³/3]

= [24 + 9 – 9] – [-8 + 1 + (1/3)]

= [24] – [-7 + 1/3]

= 24 + 7 – (1/3)

= 31 – (1/3)

= (93 – 1)/3

= 92/3 square units

Therefore, the required area is 92/3 square units.

Question. 3

Solution:

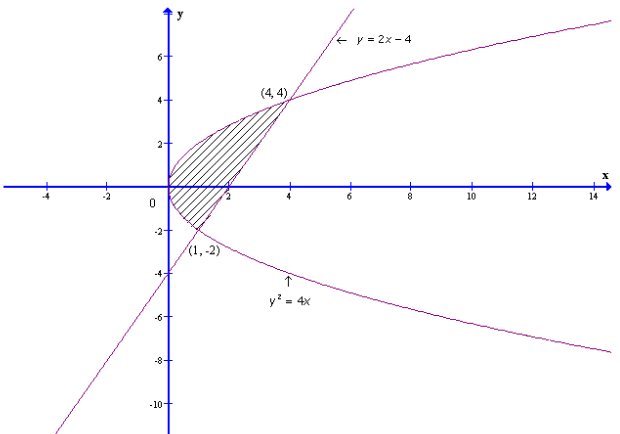
From the question it is given that, parabola y² = 4x and the line y = 2x – 4,

As shown in the figure,

So,

Now, we have to find the points of intersection,

2x – 4 = √(4x)

Squaring on both sides,

(2x – 4)² = (√(4x))²

4x² + 16 – 16x = 4x

4x² + 16 – 16x – 4x = 0

4x² + 16 – 20x = 0

Dividing both sides by 4, we get,

x² – 5x + 4 = 0

x² – 4x – x + 4 = 0

x(x – 4) – 1(x – 4) = 0

(x – 4) (x – 1) = 0

x = 4, 1

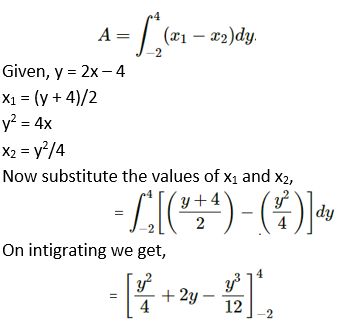
Applying limits, we get,

= [(4²/4) + 2(4) – (4³/12)] – [((-2²)/4) + 2(-2) – ((-2)³/12)]

= [4 + 8 – (64/12)] – [1 – 4 + (8/12)]

= [12 – (16/3)] – [-3 + (2/3)]

= 12 – (16/3) + 3 – (2/3)

= 15 – 18/3

= 15 – 6

= 9 square units

Therefore, the required area is 9 square units.

Question. 4

Solution:

From the question it is given that, parabola y² = 2x and the line x – y = 4,

As shown in the figure,

y² = 2x … [equation (i)]

x = y + 4 … [equation (ii)]

Now, we have to find the points of intersection,

So,

y² = 2(y + 4)

y²= 2y + 8

Transposing we get,

y² – 2y – 8 = 0

y² – 4y + 2y – 8 = 0

y (y – 4) + 2(y – 4) = 0

(y – 4) (y + 2) = 0

y = 4, -2

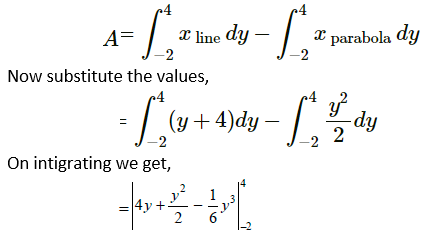
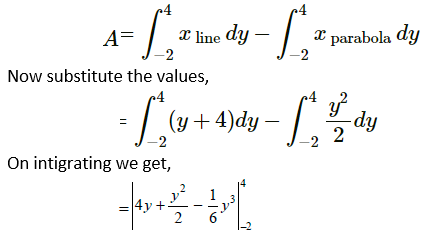
Applying limits, we get,

= 4(4 – (-2)) + ½ (4² – (-2)²) – (1/6) (4³ + 2³)

= 4(4 + 2) + ½ (16 – 4) – (1/6) (64 + 8)

= 4(6) + ½ (12) – 1/6 (72)

= 24 + 6 – 12

= 30 – 12

= 18 square units

Therefore, the required area is 18 square units.