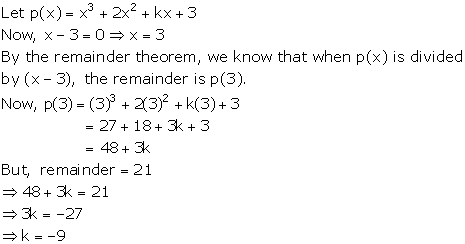ROUTERA

Chapter 2 Polynomials

Class 10th R. S. Aggarwal Maths Solution
CBSE Class 10 Maths
R. S. Aggarwal Solution

Polynomials Exercise Ex. 2A

Solution 1

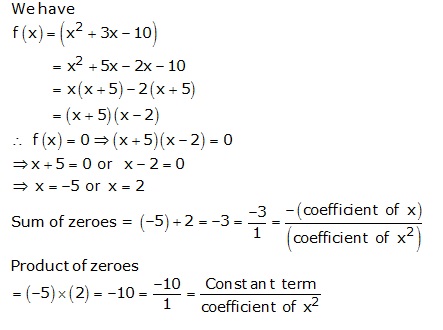
Solution 2

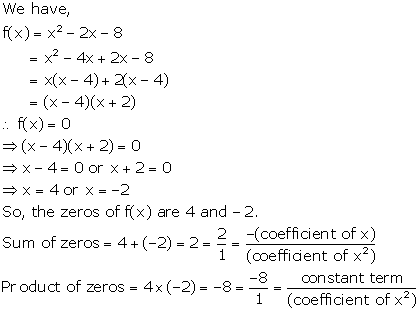
Solution 3

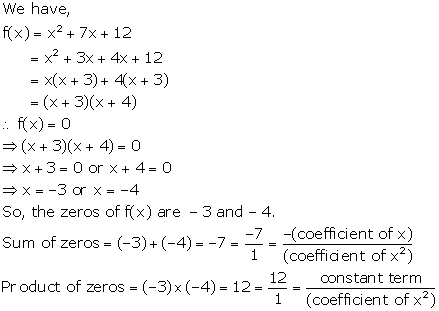
Solution 4

2x2 - x - 6 = 0

⇒ 2x2 - 4x + 3x - 6 = 0

⇒ 2x(x - 2) + 3(x - 2) = 0

⇒ (x - 2)(2x + 3) = 0

⇒ (x - 2) = 0 or (2x + 3) = 0

So, the zeroes of the given quadratic polynomial are 2 and

Sum of the zeros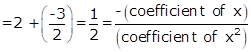

Product of the zeros

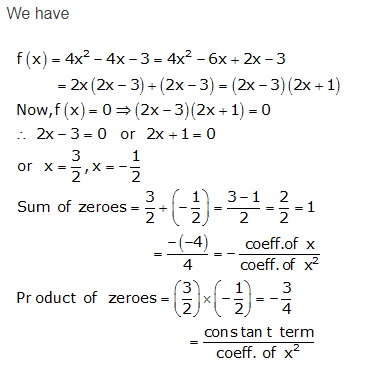
Solution 5

We have


Solution 6

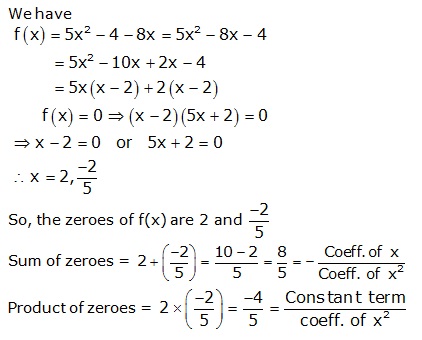
Solution 7

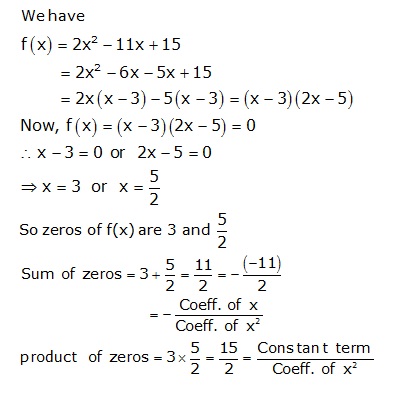
Solution 8

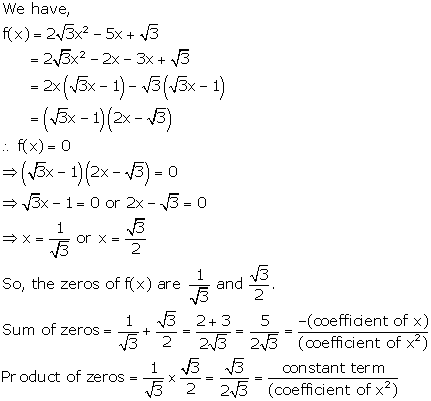
Solution 9

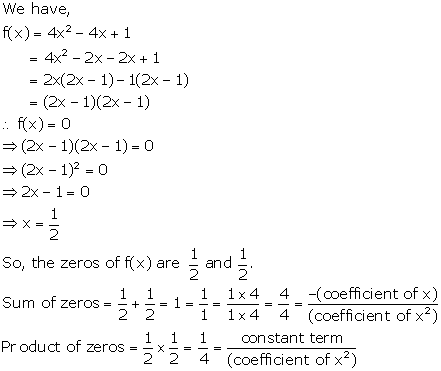
Solution 10

Solution 11

5x2 + 10x = 0

⇒ 5x(x + 2) = 0

⇒ 5x = 0 or (x + 2) = 0

⇒ x = 0 or x = -2

So, the zeroes of the given quadratic polynomial are 0 and -2.

Sum of the zeros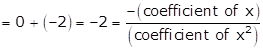

Product of the zeros

Solution 12

Let

 

 

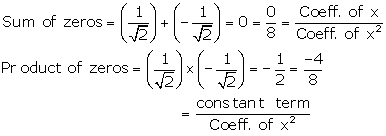
Solution 13

p(x) = 2x2 + 5x + k

Here, a = 2, b = 5 and c = k

α + β = and αβ =

α2 + β2 + αβ = …. Given

Solution 14

 

Solution 15

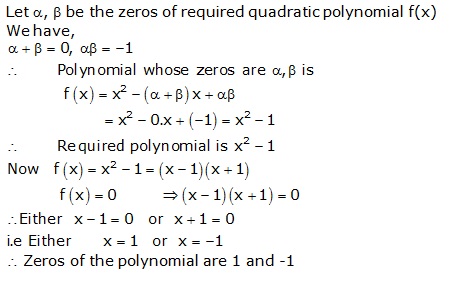
Solution 16

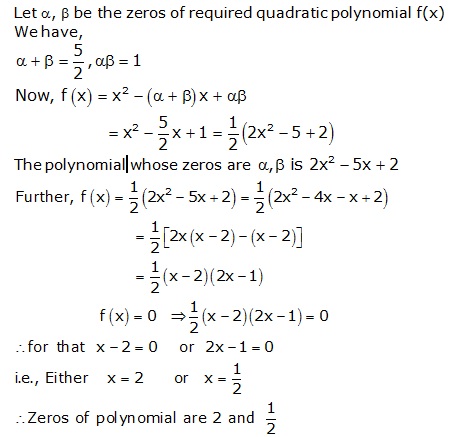
Solution 17

Let α = 2 and β = -6

Sum of zeros = α + β = 2 + (-6) = -4

Product of zeros = αβ = 2(-6) = -12

So, the required quadratic polynomial is

x2 - (α + β)x + αβ = x2 + 4x - 12

Here, a = 1, b = 4 and c = -12

Sum of the zeros

Product of the zeros

Solution 18

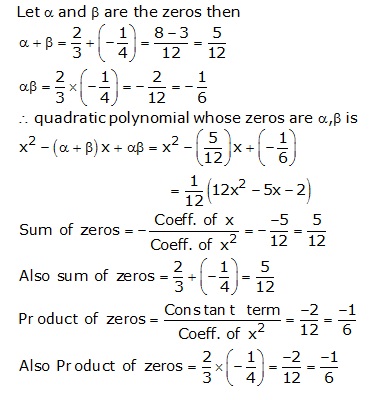
Solution 19

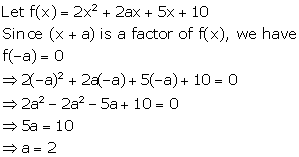
Solution 20

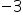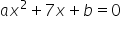Since 2 over 3 and negative 3 are zeros of a x squared plus 7 x plus b equals 0, we have

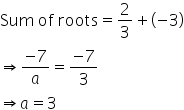
Sum space of space roots equals 2 over 3 plus open parentheses negative 3 close parentheses rightwards double arrow fraction numerator negative 7 over denominator a end fraction equals fraction numerator negative 7 over denominator 3 end fraction rightwards double arrow a equals 3

 

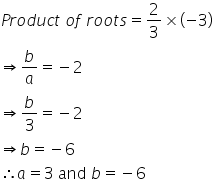
P r o d u c t space o f space r o o t s equals 2 over 3 cross times open parentheses negative 3 close parentheses rightwards double arrow b over a equals negative 2 rightwards double arrow b over 3 equals negative 2 rightwards double arrow b equals negative 6 therefore a equals 3 space and space b equals negative 6

Solution 21

Let  and  be the zeros of polynomial .

Then,

Now,

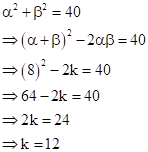
Polynomials Exercise Ex. 2B

Solution 1

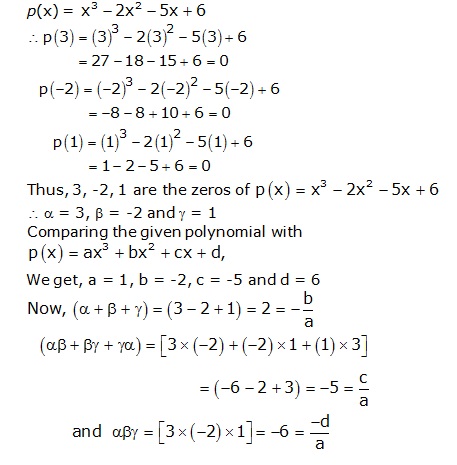
Solution 2

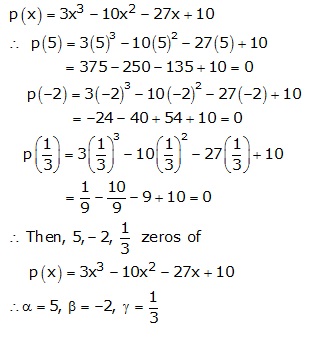
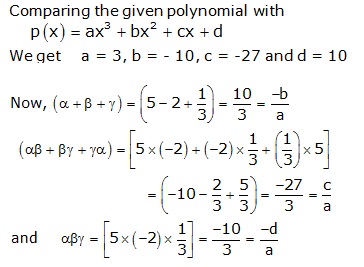
Solution 3

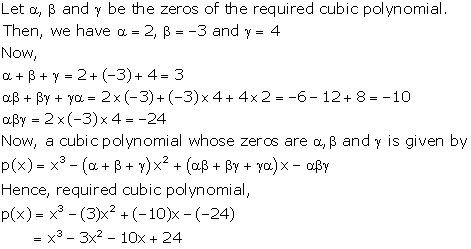
Solution 4

Solution 5

Solution 6

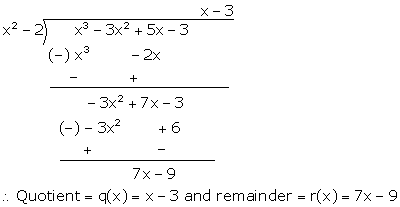
Solution 7

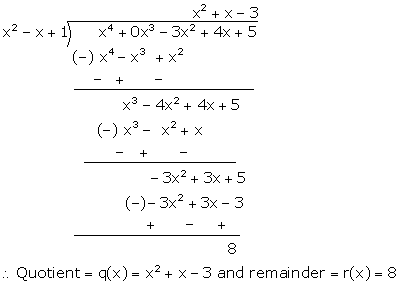
Solution 8

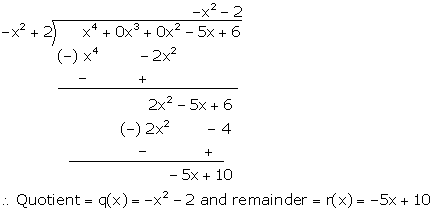
Solution 9

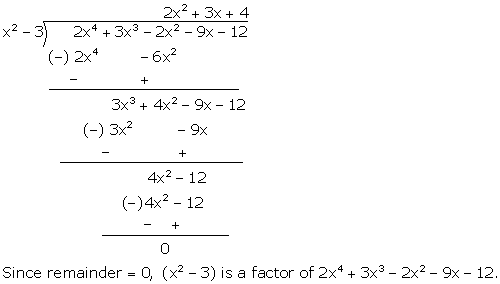
Solution 10

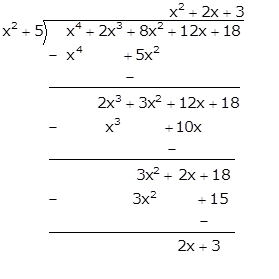
Here, remainder = 2x + 3 = px + q

⇒ p = 2 and q = 3

Solution 11

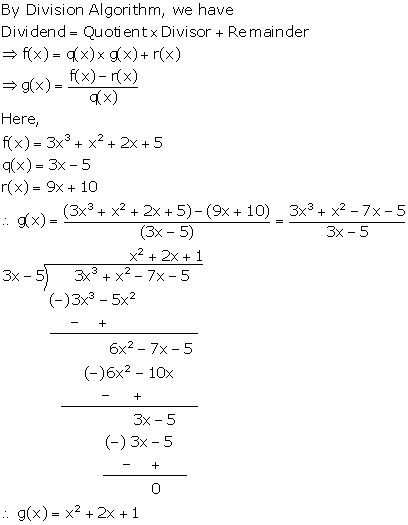
Solution 12

Solution 13


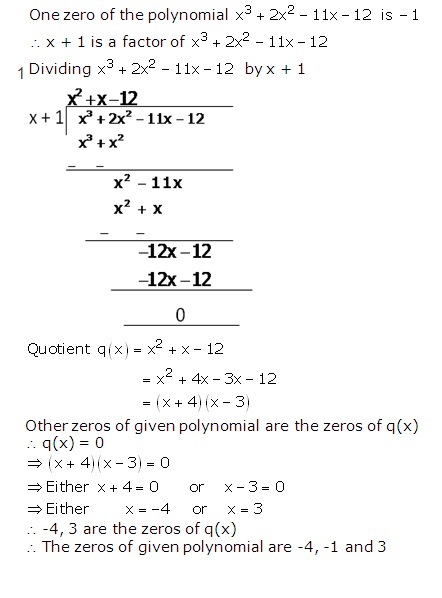
Solution 14



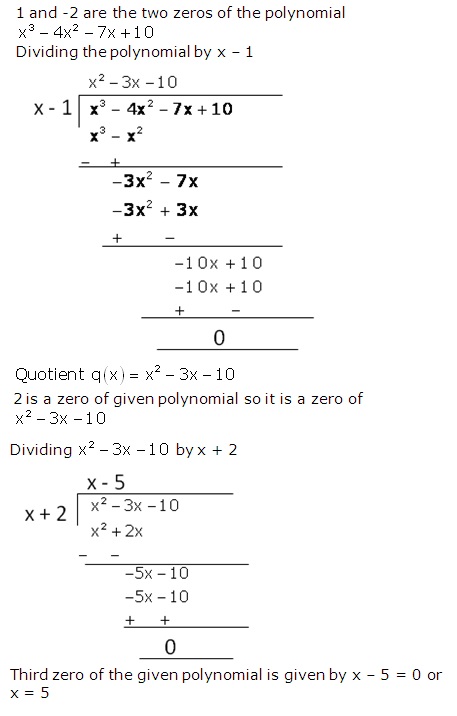
Solution 15

 

Solution 16

 and  are the zeros of the polynomial .

Then,

will divide the given polynomial completely.

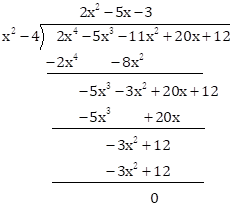
Now,

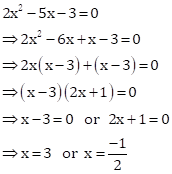
Therefore, the zeros of the given polynomial are .

Solution 17


Solution 18


Solution 19

Here, f(x) = x4 + x3 - 14x2 - 2x + 24

Since, √2 and -√2 are two zeros of f(x)

⇒ (x - √2) and (x + √2) is a factor of f(x).

⇒ (x - √2) and (x + √2) = x - 2 is a factor of f(x).

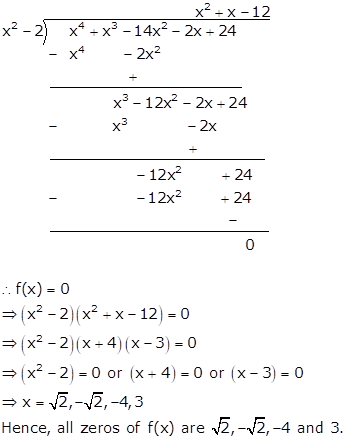
Solution 20

Here, f(x) = 2x4 - 13x3 + 19x2 + 7x - 3

Let α = (2 + √3) and β = (2 - √3). Then,

α + β = (2 + √3).+ (2 - √3) = 4

αβ = (2 + √3).(2 - √3) =4 - 3 = 1

So, the quadratic polynomial whose roots are α and β is given by

x2 - (α + β)x + αβ = x2 - 4x + 1

⇒ x2 - 4x + 1 is a factor of f(x).

Solution 21

f(x) = 3x3 + 16x3 + 15x - 18

is one zero of f(x).

is a factor of f(x).

⇒ (3x - 2) is also a factor of f(x).

Now,

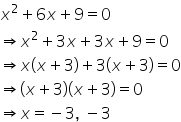
x squared plus 6 x plus 9 equals 0 rightwards double arrow x squared plus 3 x plus 3 x plus 9 equals 0 rightwards double arrow x left parenthesis x plus 3 right parenthesis plus 3 left parenthesis x plus 3 right parenthesis equals 0 rightwards double arrow left parenthesis x plus 3 right parenthesis left parenthesis x plus 3 right parenthesis equals 0 rightwards double arrow x equals negative 3 comma space minus 3

Hence, the zeros of the given polynomial are 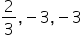2 over 3 comma negative 3 comma negative 3.

Solution 22

Here, f(x) = 2x4 - 3x3 - 3x2 + 6x - 2

1 and are two zeros of f(x).

⇒ is a factor of f(x).

⇒ is a factor of f(x).

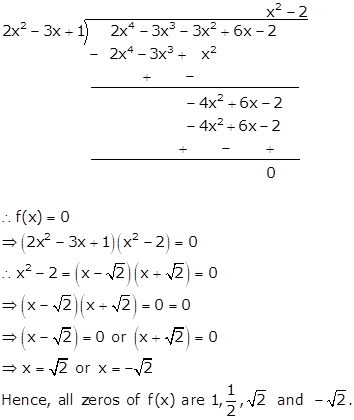
Solution 23



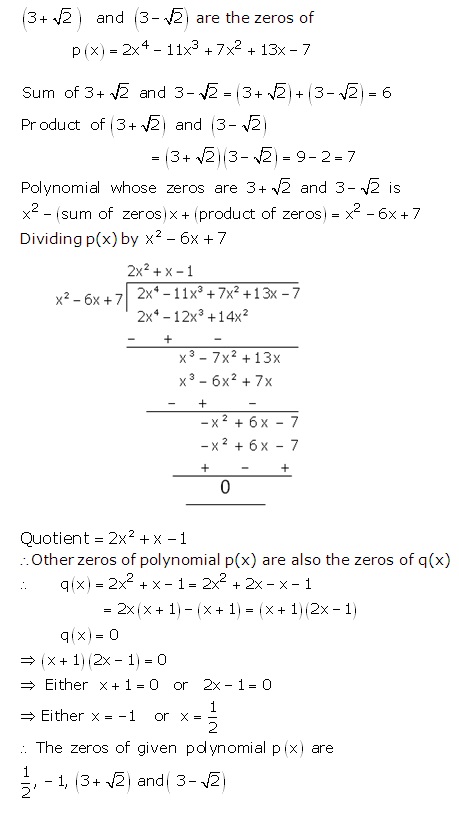
Polynomials Exercise Ex. 2C

Solution 1

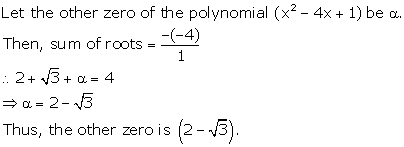
Solution 2

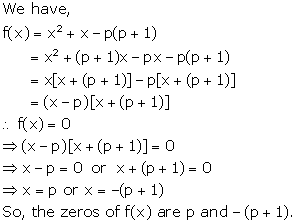
Solution 3

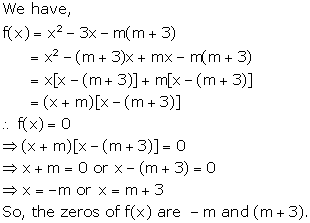
Solution 4

Solution 5

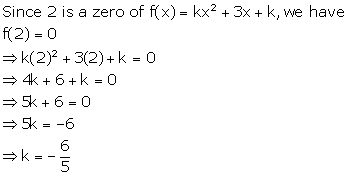
Solution 6

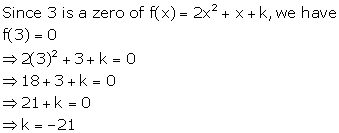
Solution 7

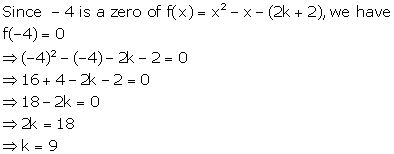
Solution 8

Solution 9

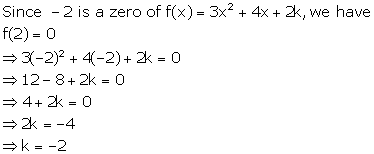
Solution 10

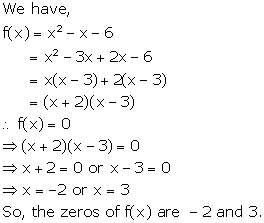
Solution 11

Solution 12

Solution 13

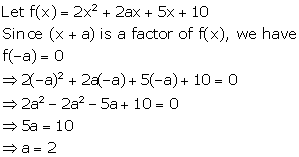
Solution 14

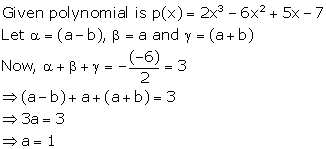
Solution 15

Solution 16

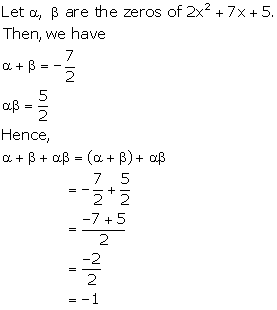
Solution 17

If f(x) and g(x) are any two polynomials with g(x) ≠ 0, then we can always find polynomials q(x) and r(x) such that f(x) = q(x)g(x) + r(x),

where r(x) = 0 or degree r(x) < degree g(x).

Solution 18

Solution 19

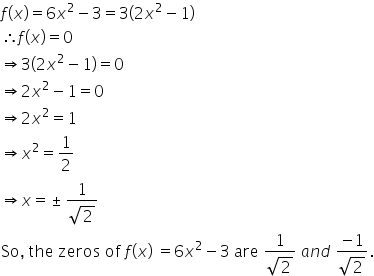
f left parenthesis x right parenthesis equals 6 x squared minus 3 equals 3 left parenthesis 2 x squared minus 1 right parenthesis therefore f left parenthesis x right parenthesis equals 0 rightwards double arrow 3 left parenthesis 2 x squared minus 1 right parenthesis equals 0 rightwards double arrow 2 x squared minus 1 equals 0 rightwards double arrow 2 x squared equals 1 rightwards double arrow x squared equals 1 half rightwards double arrow x equals plus-or-minus fraction numerator 1 over denominator square root of 2 end fraction So comma space the space zeros space of space f left parenthesis x right parenthesis space equals 6 x squared minus 3 space are space fraction numerator 1 over denominator square root of 2 end fraction space a n d space fraction numerator negative 1 over denominator square root of 2 end fraction.

Solution 20

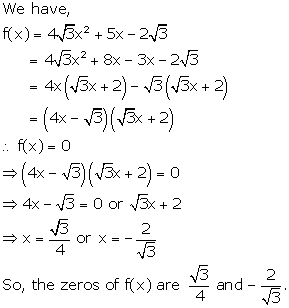
Solution 21

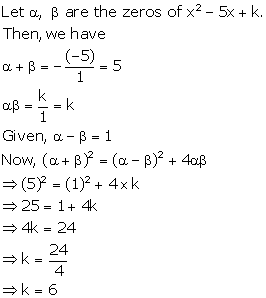
Solution 22

Solution 23

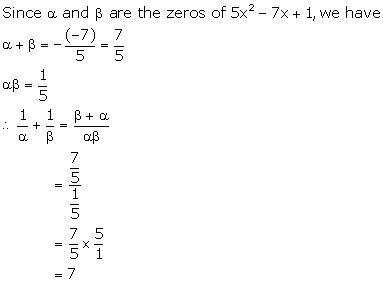
Solution 24

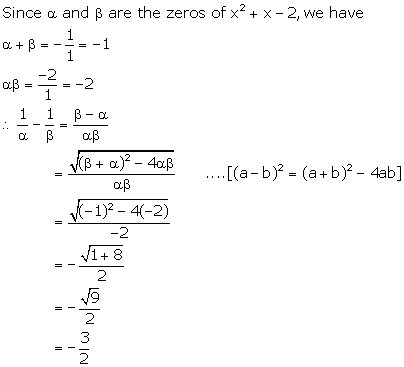
Solution 25

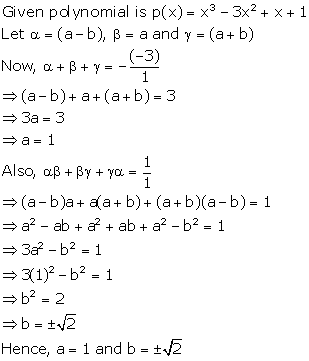
Polynomials Exercise MCQ

Solution 1

Correct answer: (d)

An expression of the form p(x) = a0 + a1x + a2x2 + ….. + anxn, where an ≠ 0, is called a polynomial in x of degree n.

Here, a0, a1, a2, ……, an are real numbers and each power of x is a non-negative integer.

Solution 2

Correct answer: (d)

An expression of the form p(x) = a0 + a1x + a2x2 + ….. + anxn, where an ≠ 0, is called a polynomial in x of degree n.

Here, a0, a1, a2, ……, an are real numbers and each power of x is a non-negative integer.

Solution 3

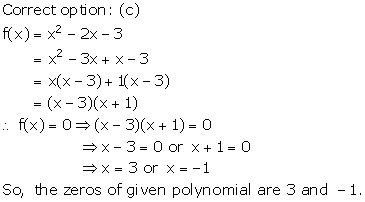
Solution 4

Solution 5

Solution 6

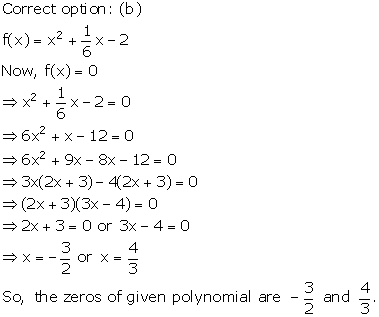
Solution 7

Solution 8

Solution 9

Solution 10

Solution 11

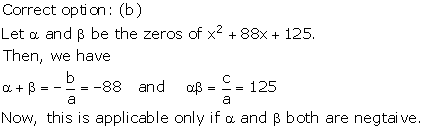
Solution 12

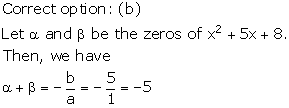
Solution 13

Correct option: (c)


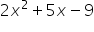alpha space and space beta be the zeros of 2 x squared plus 5 x minus 9.

Then,

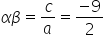
alpha beta equals c over a equals fraction numerator negative 9 over denominator 2 end fraction

Solution 14

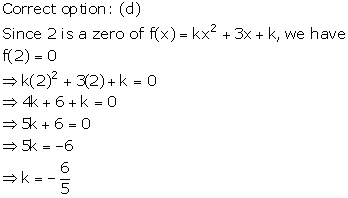
Solution 15

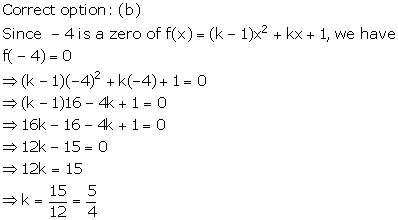
Solution 16

Solution 17

Solution 18

Solution 19

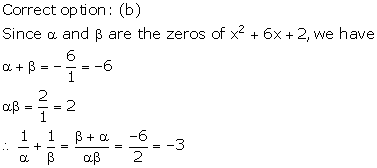
Solution 20

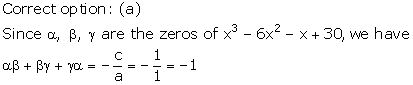
Solution 21

Solution 22

Solution 23

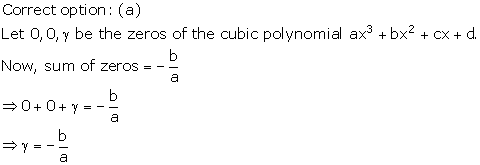
Solution 24

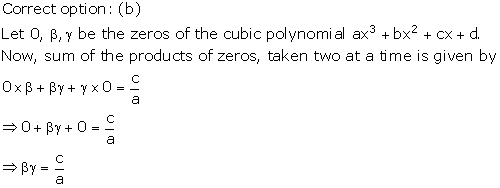
Solution 25

Solution 26

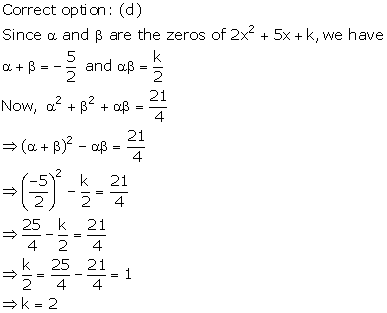
Solution 27

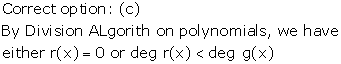
Solution 28

Polynomials Exercise Test Yourself

Solution 1

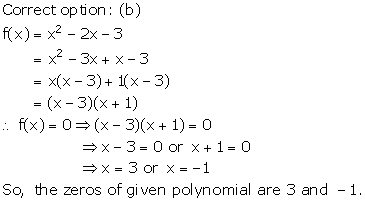
Solution 2

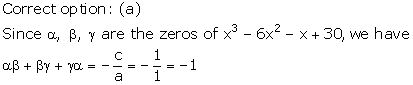
Solution 3

Solution 4

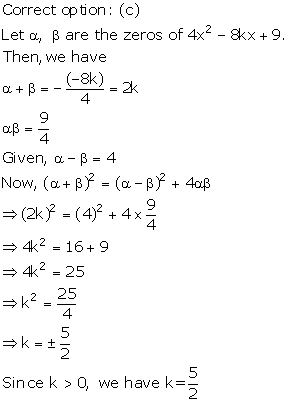
Solution 5

Solution 6

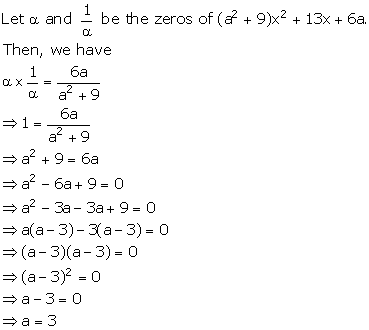
Solution 7

Solution 8

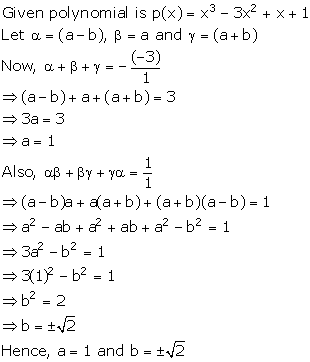
Solution 9

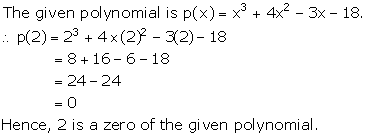
Solution 10

Solution 11

Solution 12

Solution 13

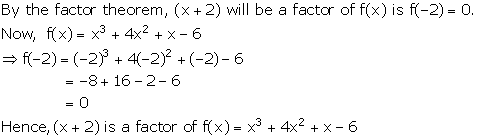
Solution 14

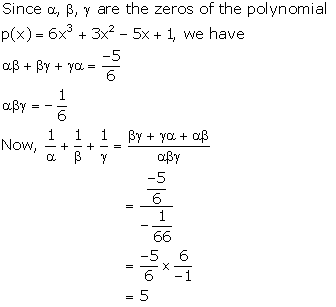
Solution 15

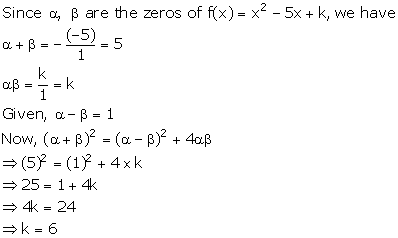
Solution 16

Solution 17

Solution 18

Solution 19

 

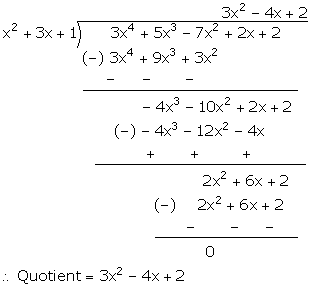
Solution 20