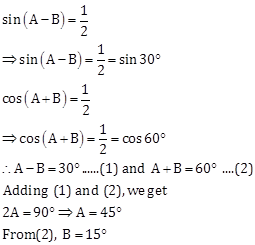Chapter 11 T-Ratios of Some Particular Angles

Class 10^th R. S. Aggarwal Maths Solution

CBSE Class 10 Maths

R. S. Aggarwal Solution

T-Ratios of Some Particular Angles Exercise Ex. 11

Solution 1

On substituting the value of various T-ratios, we get

sin60^o cos30^o + cos60^o sin30^o

Solution 2

sin60° cos30° - cos60° sin30°

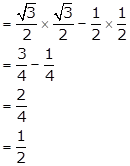
Solution 3

cos60° cos30° - sin60° sin30°

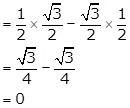
Solution 4

cos45° cos30° + sin45° sin30°

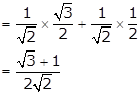
Solution 5

tan30° cosec60° - tan60° sec30°

Solution 6

On substituting the value of various Tratios, we get

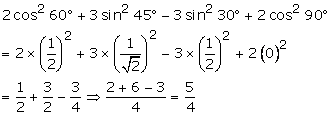
Solution 7

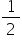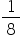sin²30° cos²45° + 4 tan²30° + $1 half$ sin²90° + $1 over 8$ cot²60°

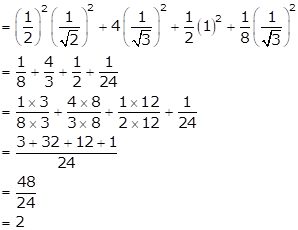
Solution 8

On substituting the value of various Tratios, we get

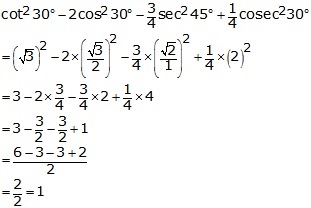
Solution 9

(cos0° + cos45° + sin30°)(sin 90° - cos45° + cos60°)

Solution 10

(i)

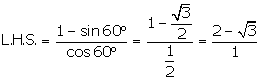
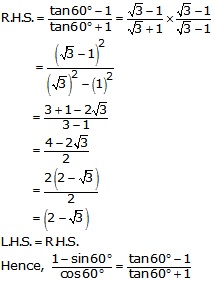
(ii)

L.H.S = R.H.S.

Solution 11

(i)

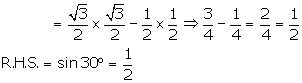
R.H.S. = L.H.S.

Hence, sin60° cos30° - cos60° sin30° = sin30°

(ii)L.H.S. = cos60° cos30° + sin60° sin30°

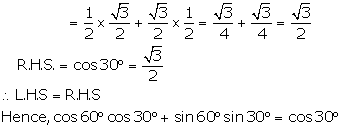
(iii)

R.H.S. = L.H.S.

Hence,2sin30° cos30° = sin60°

(iv)

R.H.S. = sin90° = 1

R.H.S. = L.H.S.

Hence, 2 sin 45° cos45° = sin90°

Solution 12

A = 45° 2 A = 90°

(i)Sin 2A = sin90° = 1

(ii) cos2A = cos90° = 0

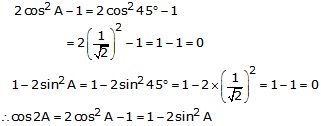
Solution 13

A = 302A = 60

(i)

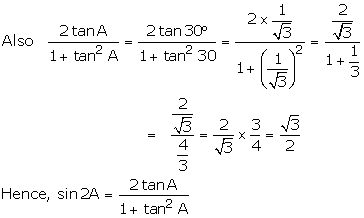
(ii)

(iii)

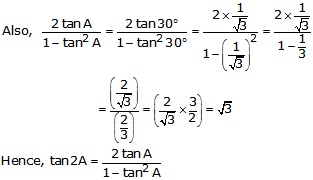
Solution 14(i)

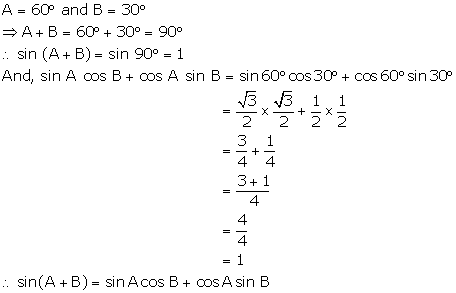
Solution 14(ii)

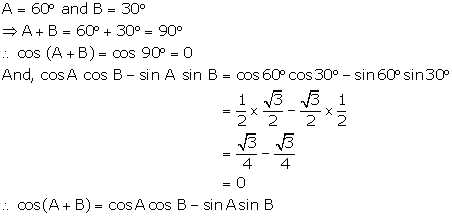
Solution 15(i)

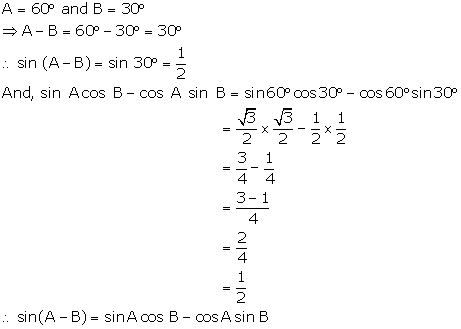
Solution 15(ii)

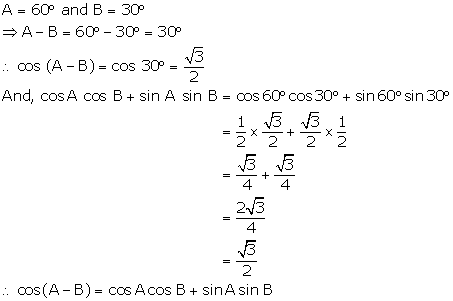
Solution 15(iii)

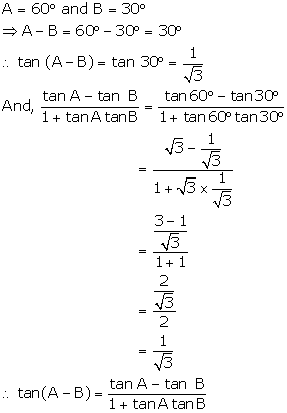
Solution 16

Hence, (A + B) = 45^o

Solution 17

Putting A = 30^o 2 A = 60^o

Solution 18

Putting A = 30^o 2 A = 60^o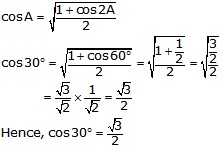

Solution 19

Putting A = 30^o 2 A = 60^o

Solution 20

Let A = 45° and B =30°

(i) sin75°

= sin(45°+ 30°)

= sin 45° cos 30° + cos 45° sin 30°

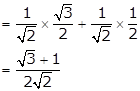
(ii) cos15°

= cos(45°- 30°)

= cos 45° cos 30° + sin 45° sin 30°

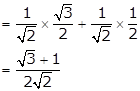
Solution 21

tan(x + 30°) = 1

We know that tan45° = 1

⇒ tan (15° + 30°) = 1

∴ x = 15°

Solution 22

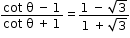
$begin mathsize 12px style fraction numerator cot space straight theta space minus space 1 over denominator cot space straight theta space plus space 1 end fraction equals fraction numerator 1 space minus space square root of 3 over denominator 1 space plus thin space square root of 3 end fraction end style$

⇒ (1 + √3)(cot θ - 1) = (1 - √3)(cot θ + 1)

⇒ cot θ - 1 + √3 cot θ - √3 = cot θ + 1 - √3 cot θ - √3

⇒ 2√3 cot θ = 2

⇒ cot θ = 1√3 = cot 60°

⇒ θ = 60°

Solution 23

sin(A + B) = 1 = sin90°

⇒ A + B = 90°……(1)

tan(A - B) = 1/√3 = tan 30°

⇒ A - B = 30°…….(2)

Adding (1) and (2), we get

A = 60° and B = 30°

Solution 24

sin(A + B) = √3/2 = sin60°

⇒ A + B = 60°…….(1)

Cos(A - B) = √3/2 = cos30°

⇒ A - B = 30°……(2)

Adding (1) and (2), we get

A = 45° and B = 15°

Solution 25

Solving (1) and (2), we get

2A = 90^oA = 45^o

Putting A = 45^o in (1), we get

45^o - B = 30^o B = 45^o - 30^o = 15^o

A = 45^o, B = 15^o

Solution 26

cosec(A + B) = 1 - cosec90°

⇒ A + B = 90°…….(1)

Cosec(A - B) = 2 = cosec30°

⇒ A - B = 30°…..(2)

Adding (1) and (2), we get

A = 60° and B = 30°

(i) sinA cosB + cosA sinB

= sin60° cos30° + cos60°sin30°

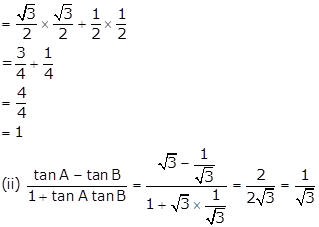
Solution 27

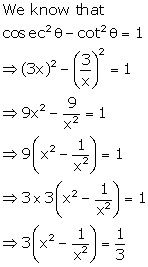
Solution 28

From right angled ABC,

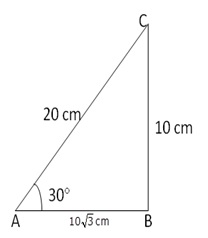
Solution 29

From right angled ABC,

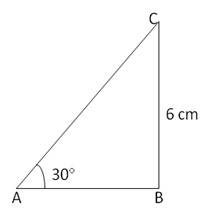
Solution 30

From right angled ABC,

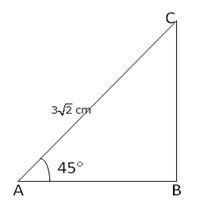
(i)

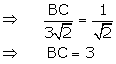
(ii) By Pythagoras theorem

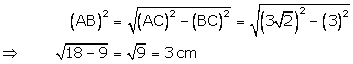
Hence, (i) BC = 3cm and (ii) AB = 3cm

Solution 31

(i) x tan 45° cot60° = sin 30° cosec60°

⇒ x = 1

(ii) 2cosec²30° + x sin² 60° - ¾ tan² 30° = 10

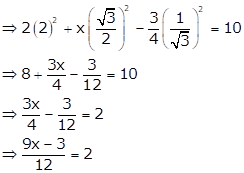
⇒ 9x = 27

⇒ x = 3

T-Ratios of Some Particular Angles Exercise MCQ

Solution 1

Correct Option: (c)

Solution 2

Correct Option: (d)

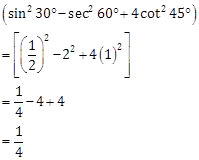
Solution 3

Correct Option: (c)

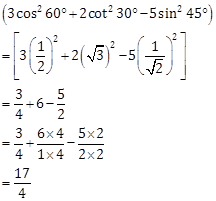
Solution 4

Correct Option: (b)

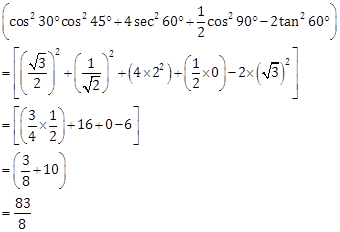
Solution 5

Correct option: (a)

Solution 6

Correct Option: (c)

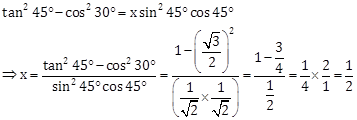
Solution 7

Correct Option: (a)

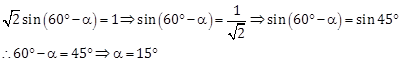
Solution 8

Correct Option: (a)

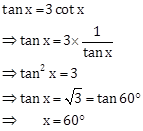
Solution 9

Correct Option: (b)

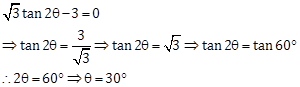
Solution 10

Correct Option: (a)

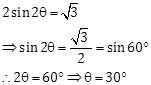
Solution 11

Correct Option: (c)

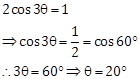
Solution 12

Correct Option: (a)

Solution 13

Correct Option: (d)

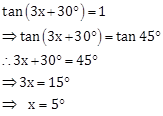
Solution 14

Correct Option: (b)

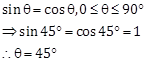
Solution 15

Correct Option: (c)