ROUTERA

Chapter 7 Triangles

Class 10th R. D. Sharma Maths Solution
CBSE Class 10 Maths
R. D. Sharma Solution

Triangles Exercise Ex. 7.1

Solution 1

(i) All circles are similar.
(ii) All squares are similar.
(iii) All equilateral triangles are similar.

(iv) Two triangles are similar, if their corresponding angles are equal.

(v) Two triangles are similar, if their corresponding sides are proportional.
(vi) Two polygons of the same number of sides are similar, if (a) their corresponding angles are equal and (b) their corresponding sides are proportional.

Solution 2

(i) False

(ii) True

(iii) False

(iv) False

(v) True

(vi) True

Triangles Exercise Ex. 7.2

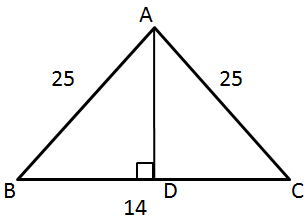
Solution 1(i)



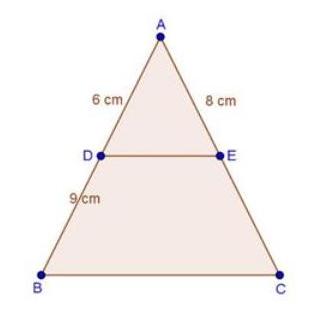
Solution 1(ii)



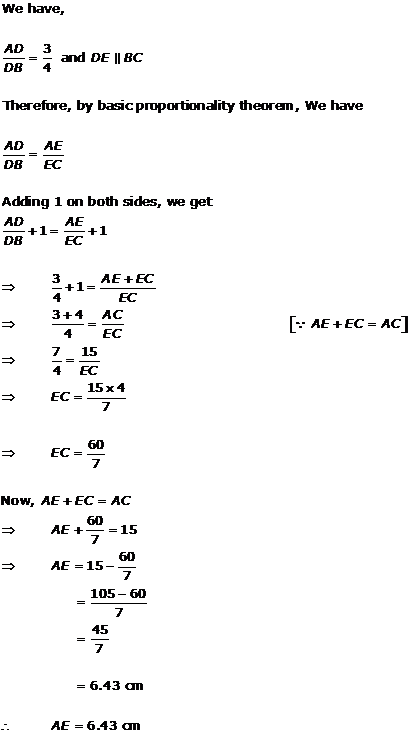
Solution 1(iii)



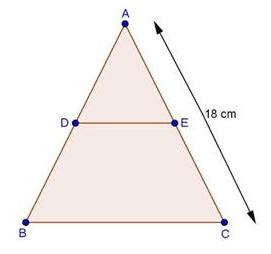
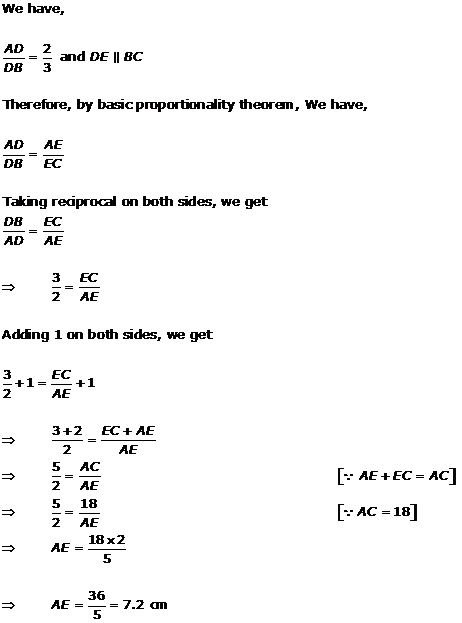
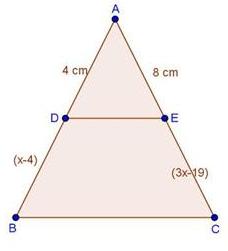
Solution 1(iv)



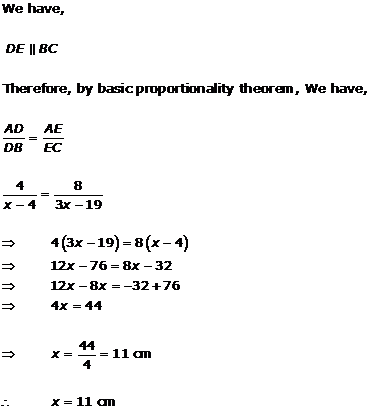
Solution 1(v)



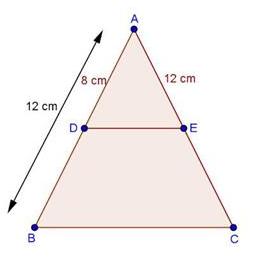
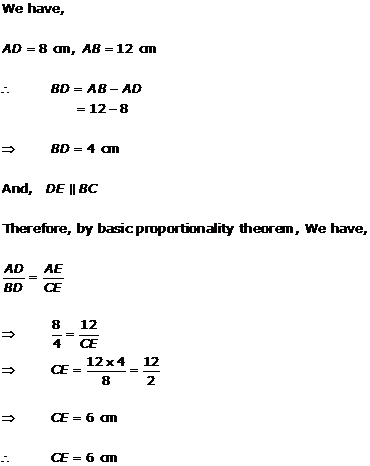
Solution 1(vi)



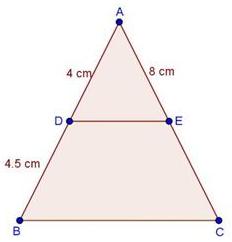
Solution 1(vii)



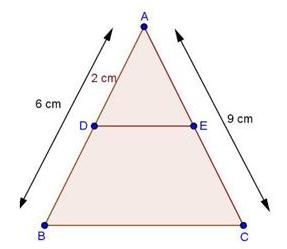
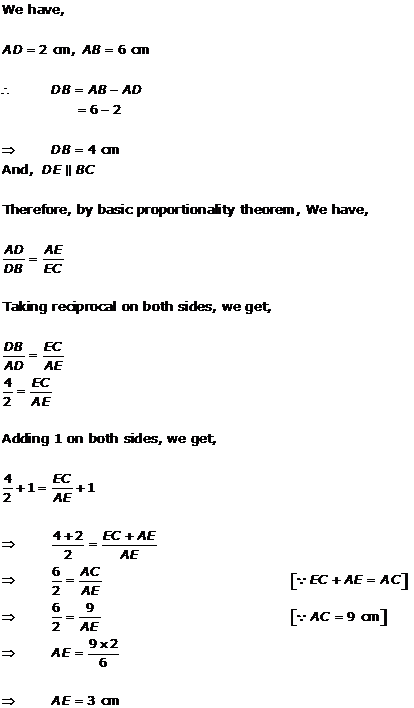
Solution 1(viii)



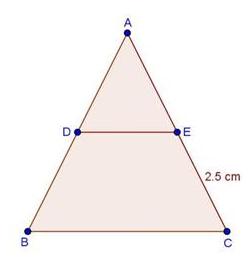
Solution 1(ix)



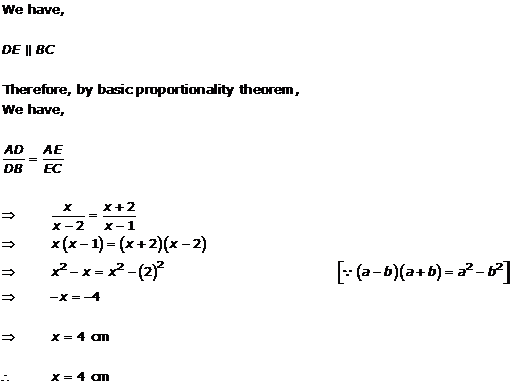
Solution 1(x)



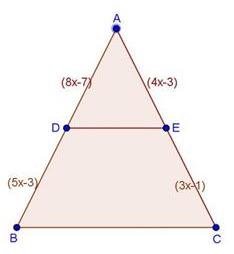
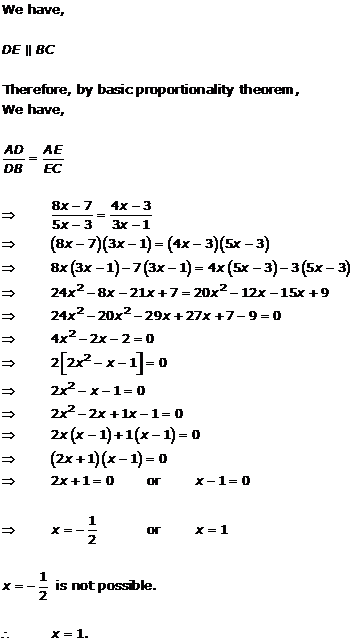
Solution 1(xi)



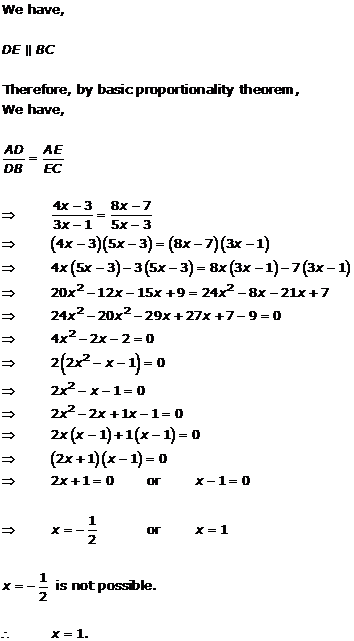
Solution 1(xii)



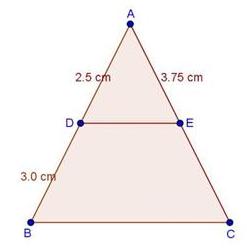
Solution 2(i)



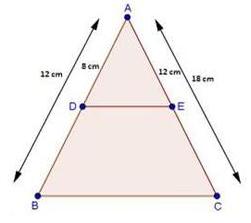
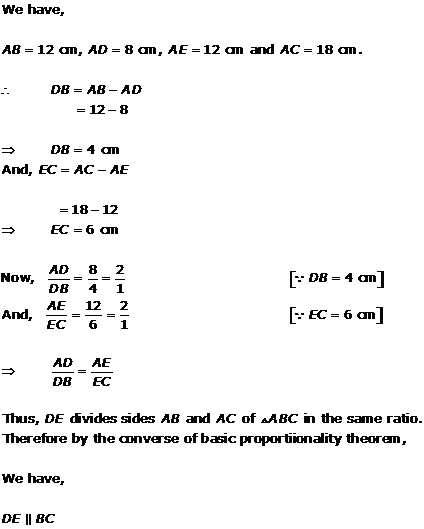
Solution 2(ii)



Solution 2(iii)



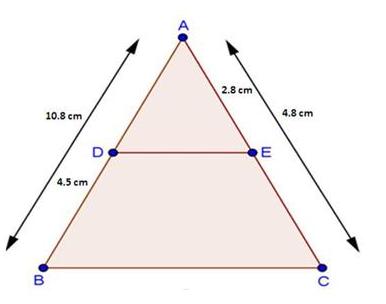
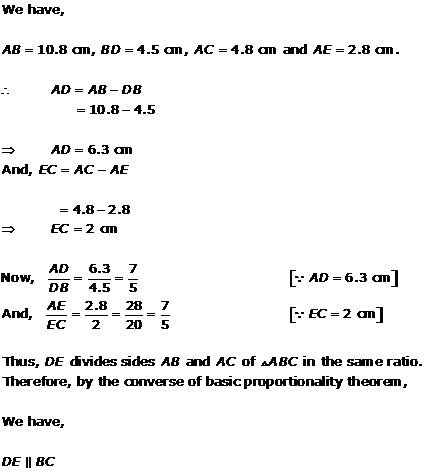
Solution 2(iv)



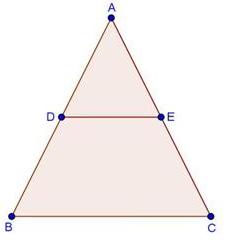
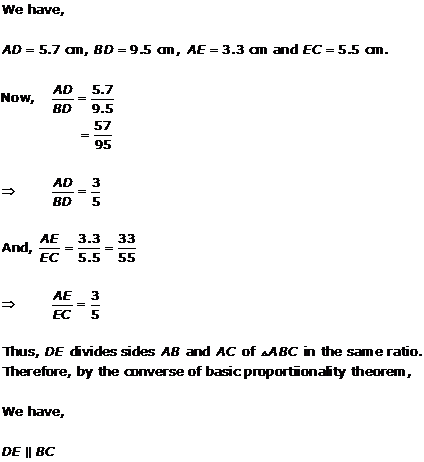
Solution 3




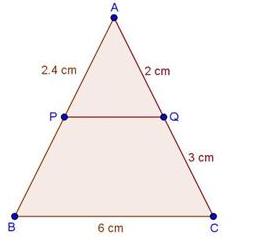
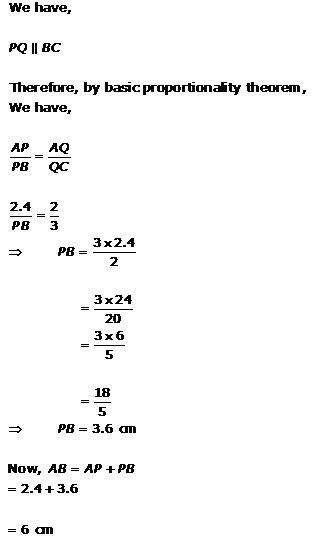
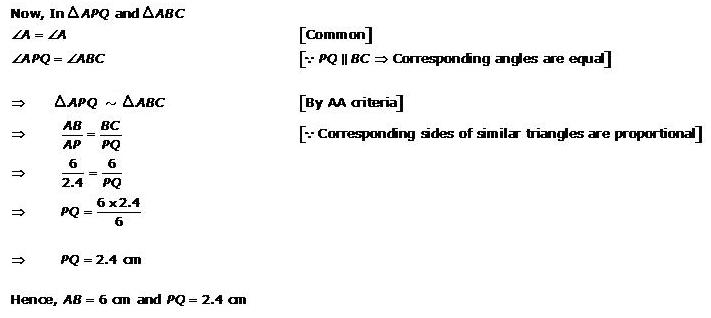
Solution 4




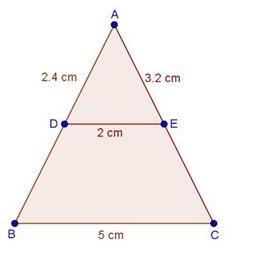
Solution 5

Solution 6




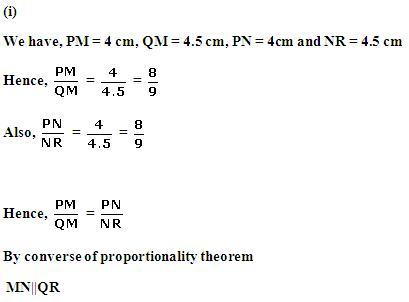
Solution 7



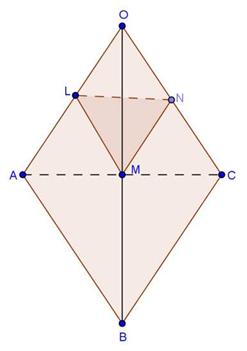
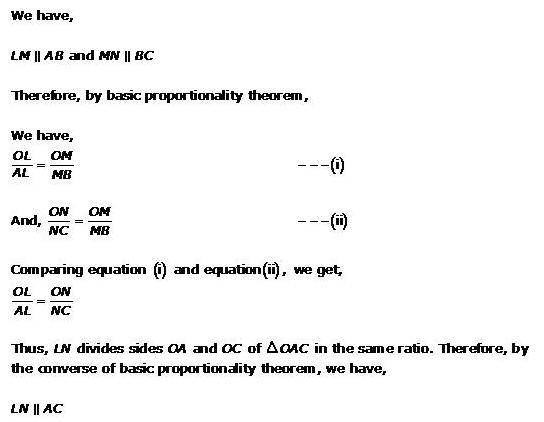
Solution 8



Triangles Exercise Ex. 7.3

Solution 1(i)



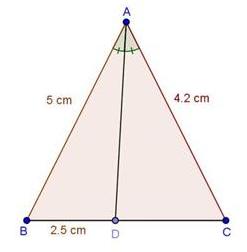
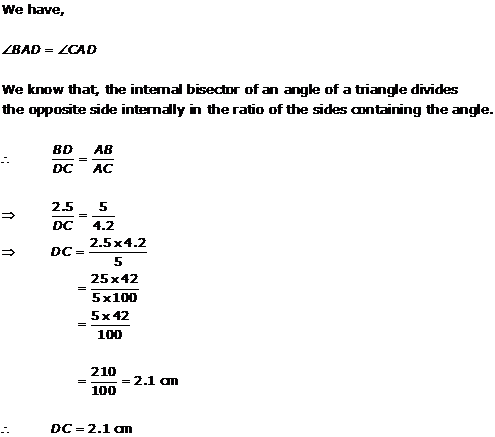
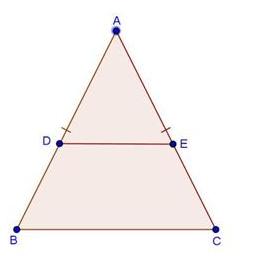
Solution 1(ii)



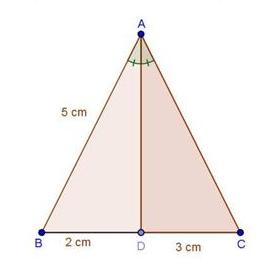
Solution 1(iii)



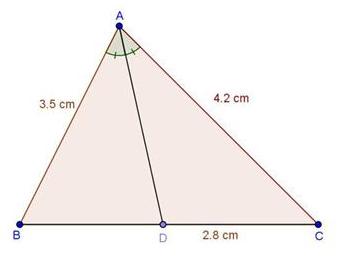
Solution 1(iv)



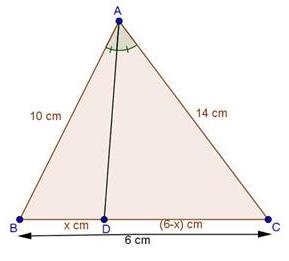
Solution 1(v)



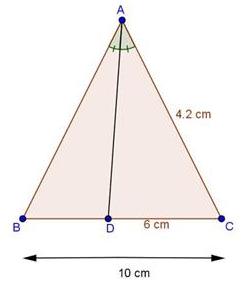
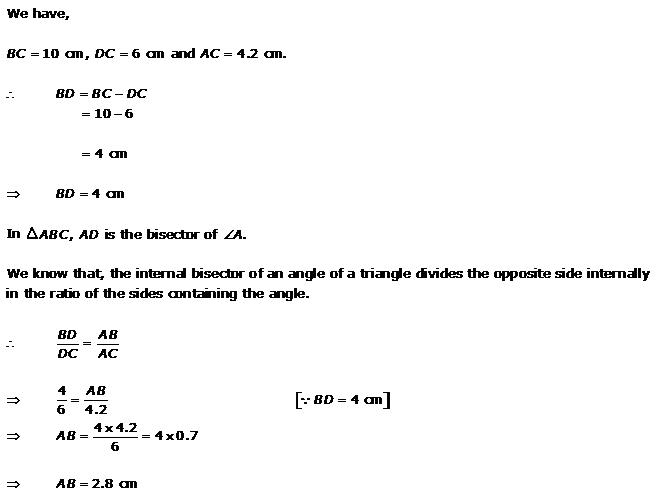
Solution 1(vi)



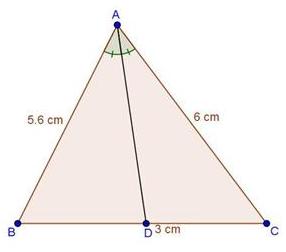
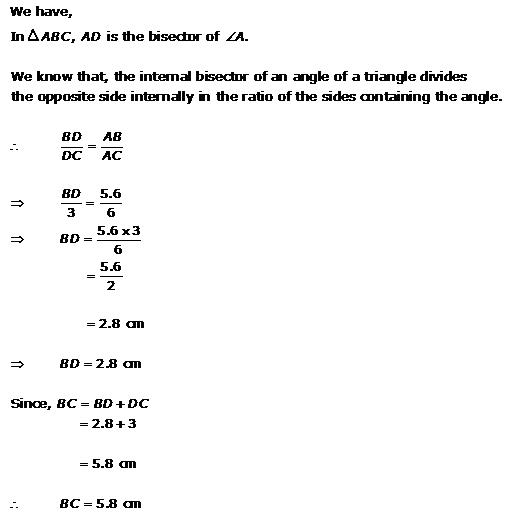
Solution 1(vii)



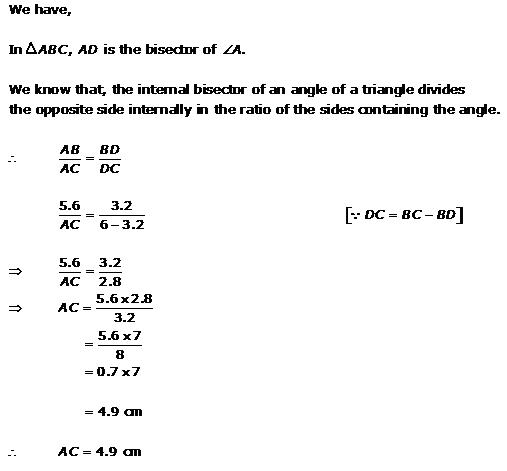
Solution 1(viii)



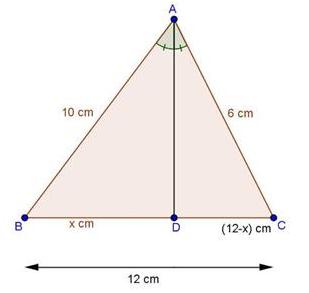
Solution 2

Solution 3

Solution 4(i)

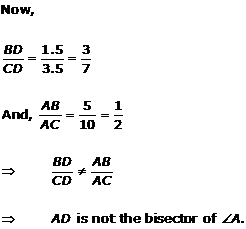
Solution 4(ii)

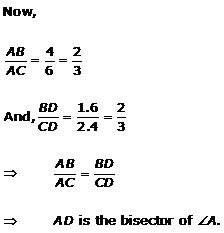
Solution 4(iii)

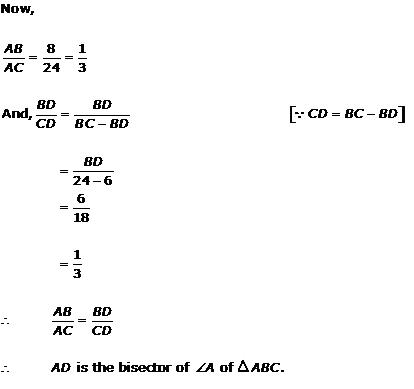
Solution 4(iv)

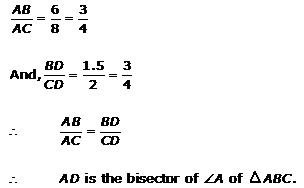
Solution 4(v)

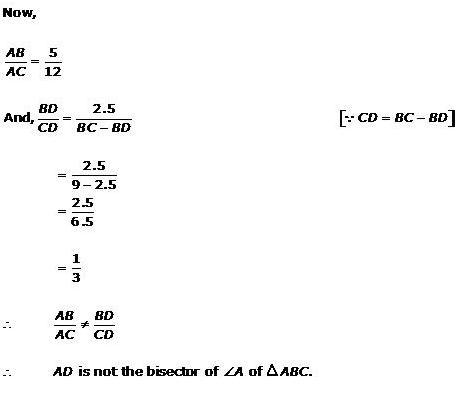
Solution 5

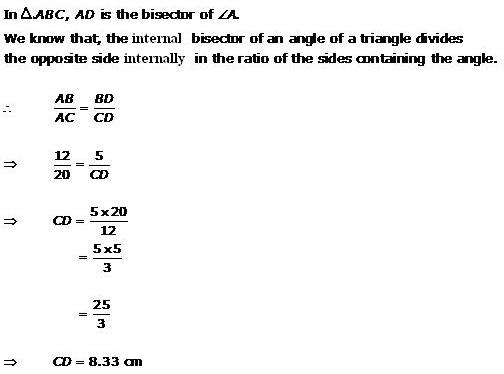
Solution 6

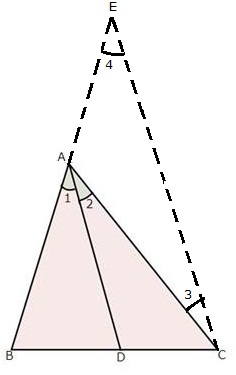
Solution 7




Triangles Exercise Ex. 7.4

Solution 1(i)

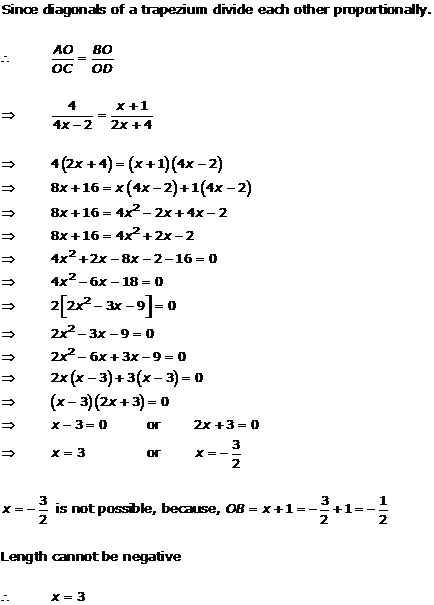
Solution 1(ii)

Solution 1(iii)

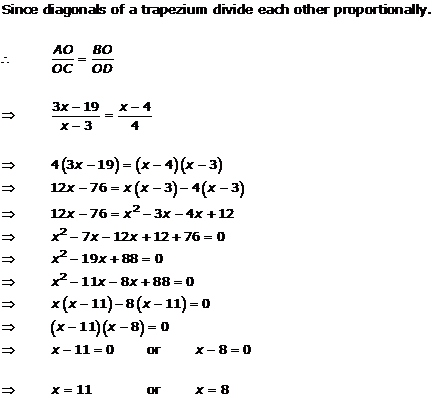
Triangles Exercise Ex. 7.5

Solution 1

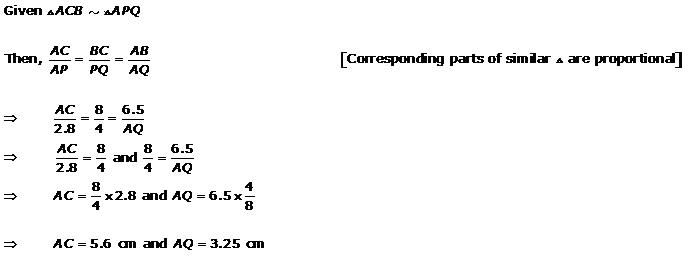
Solution 2

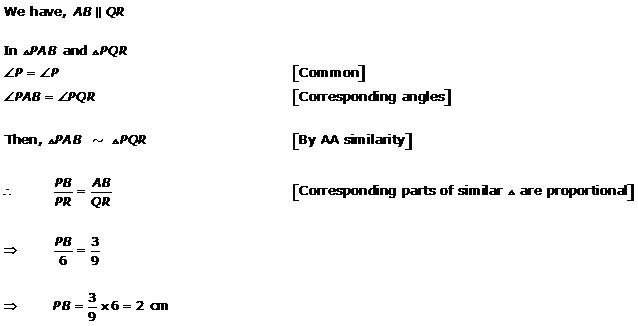
Solution 3

Solution 4

We have: 



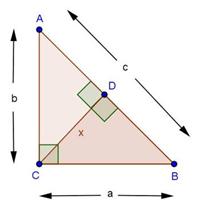
Solution 5

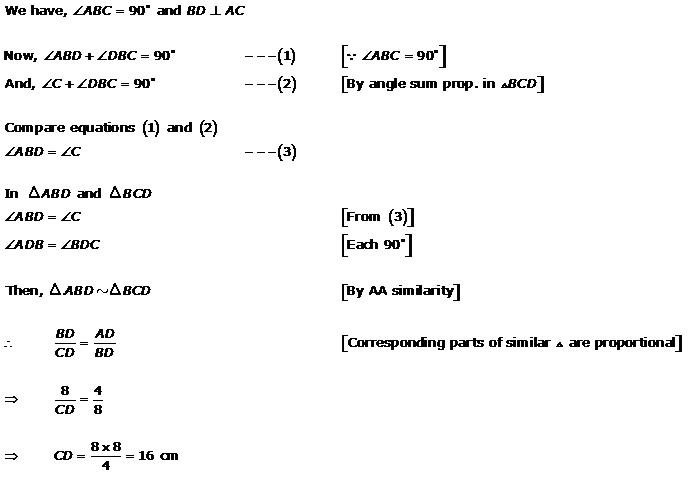
Solution 6

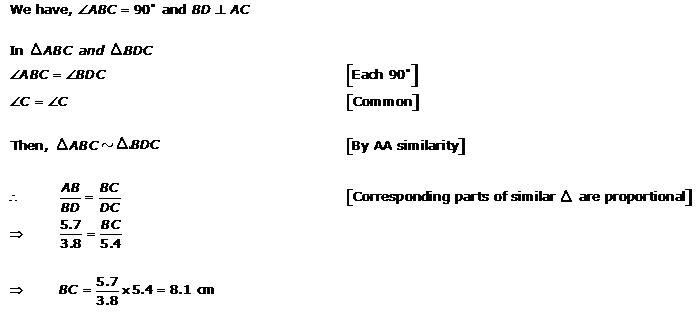
Solution 7

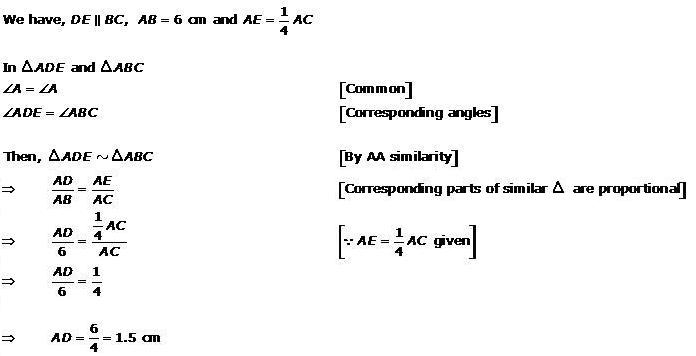
Solution 8

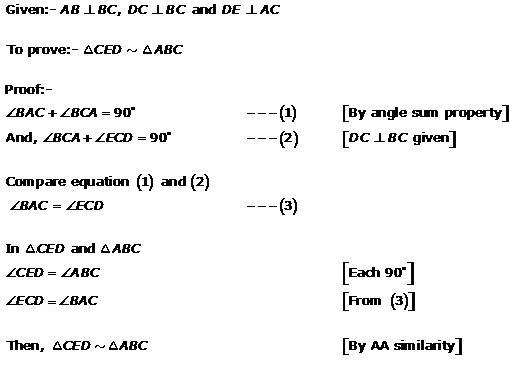
Solution 9



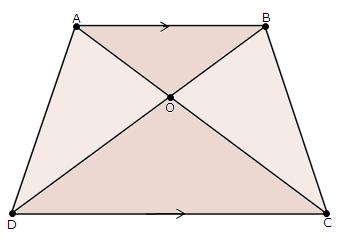
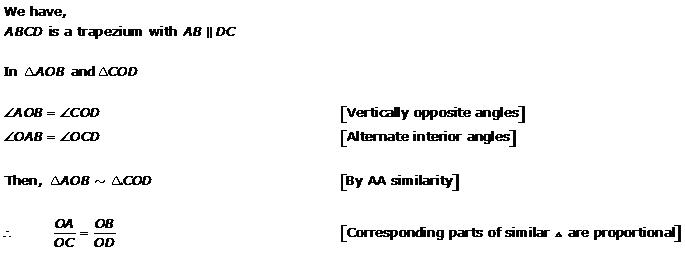
Solution 10




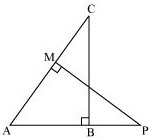
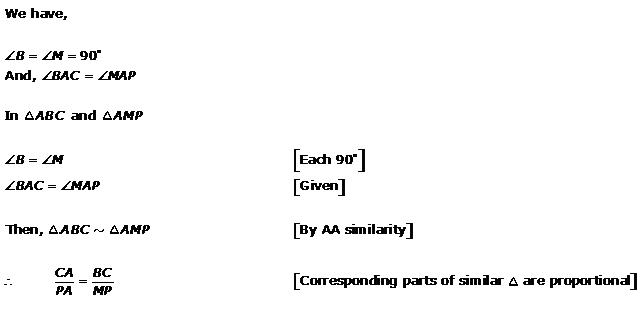
Solution 11




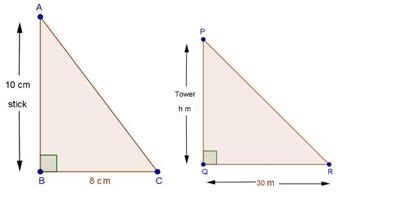
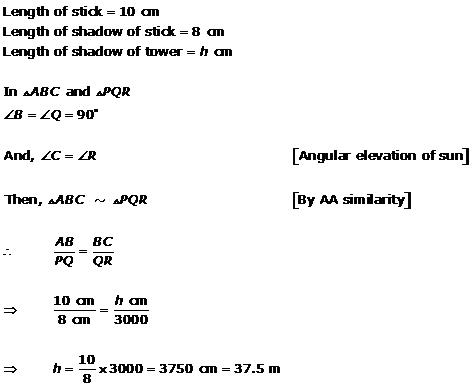
Solution 12

Solution 13

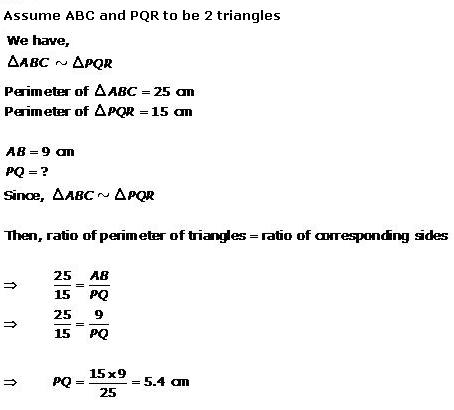
Solution 14



Solution 15



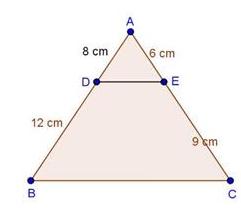
Solution 16



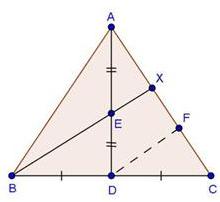
Solution 17



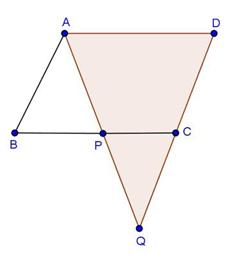
Solution 18



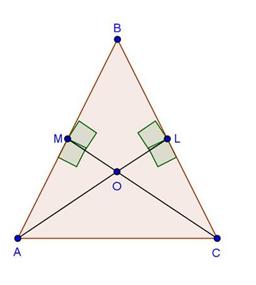
Solution 19




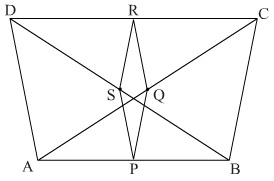
Solution 20



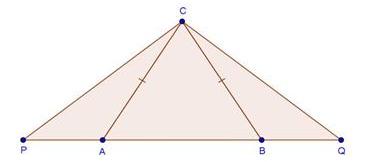
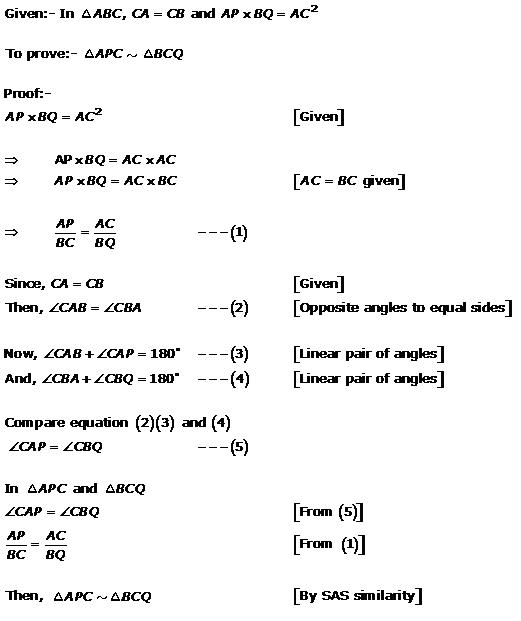
Solution 21



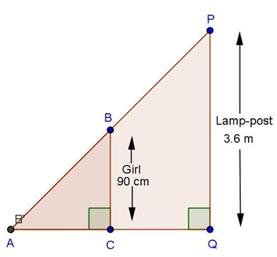
Solution 22

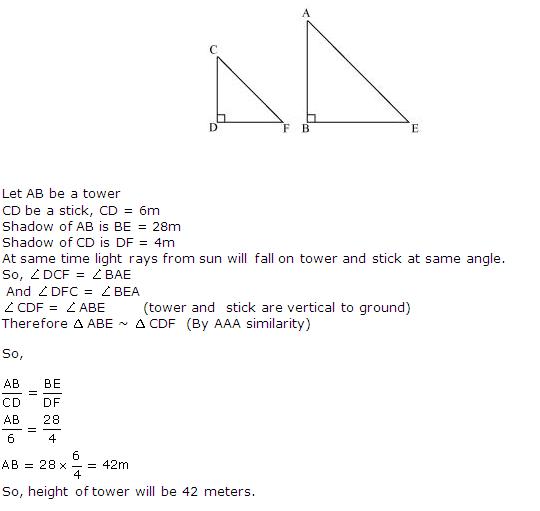
Solution 23

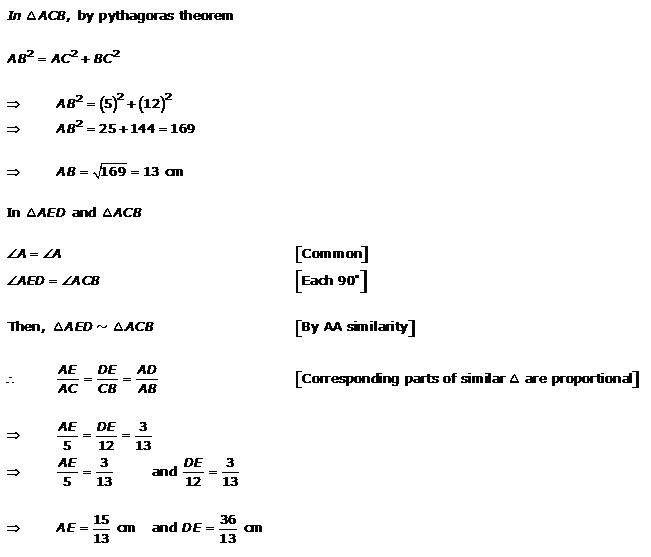
Solution 24

Solution 25



Triangles Exercise Ex. 7.6

Solution 1(i)

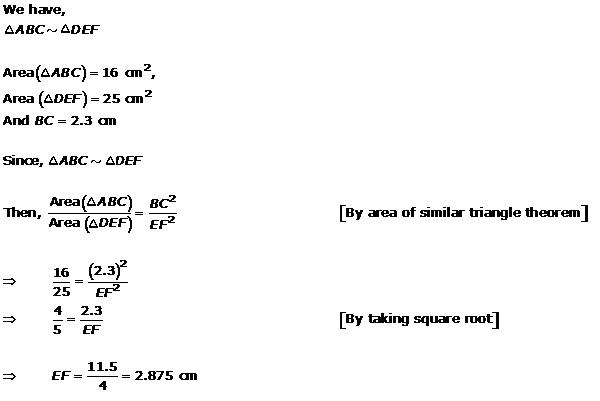
Solution 1(ii)

Solution 1(iii)

Solution 1(iv)

Solution 1(v)

Solution 2

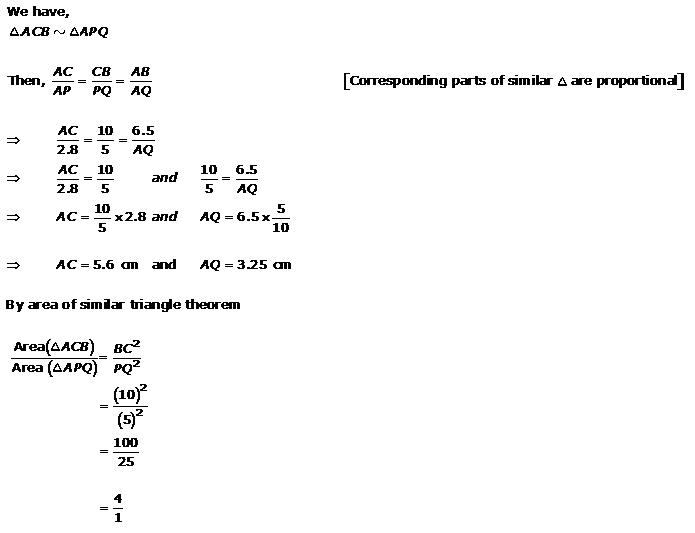
Solution 3



Solution 4



Solution 5



Solution 6



Solution 7



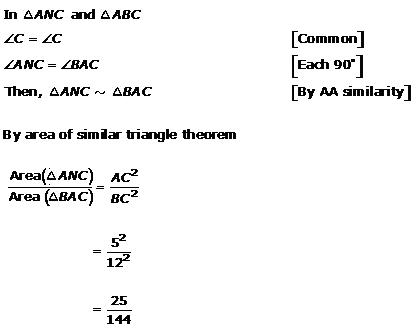
Solution 8(i)

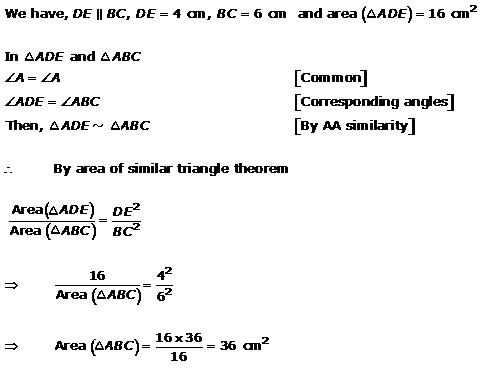
Solution 8(ii)

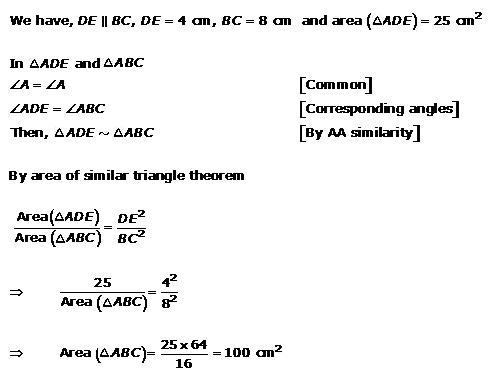
Solution 8(iii)

Solution 9



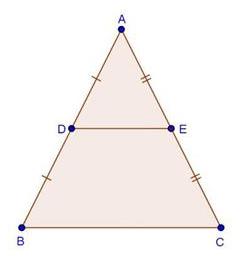
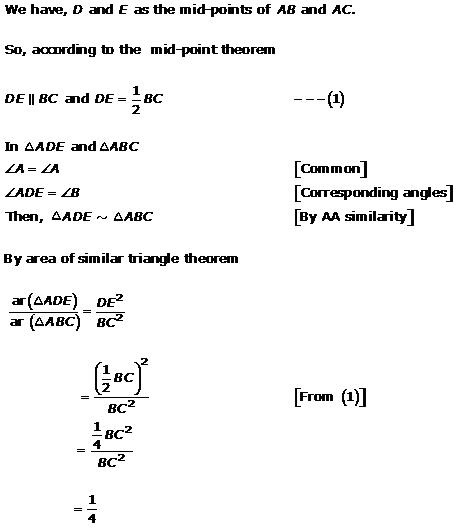
Solution 10



Solution 11


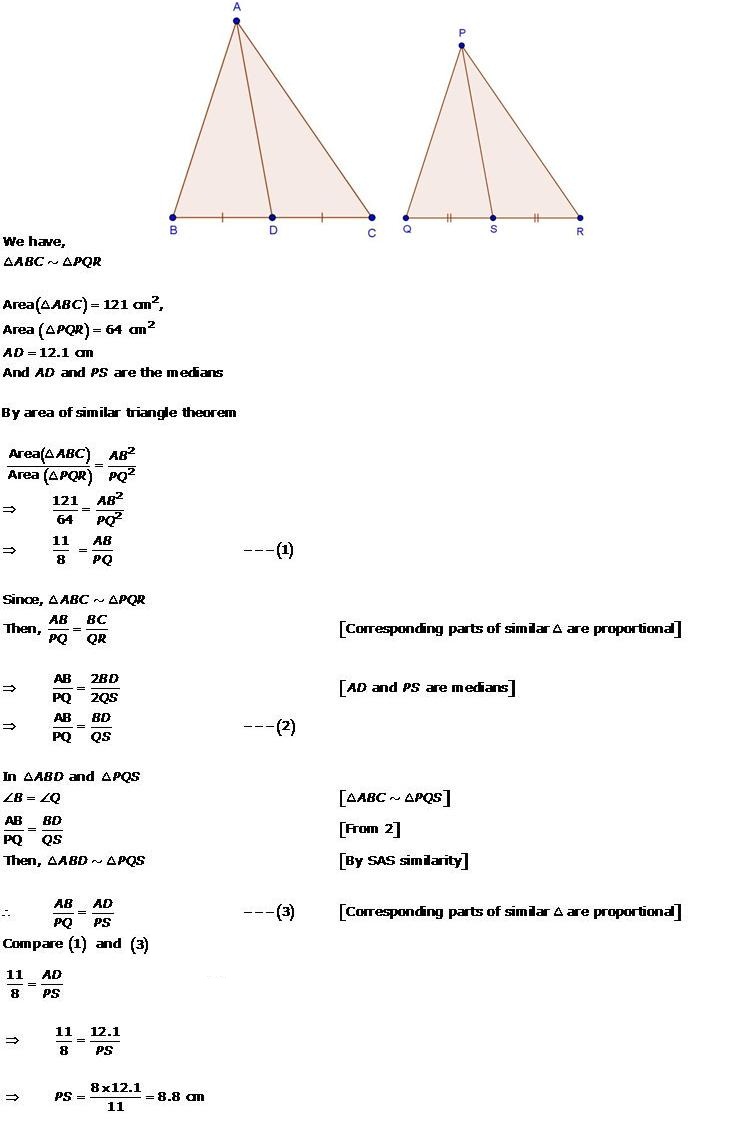
Solution 12

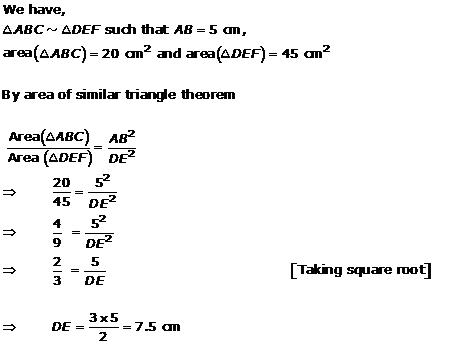
Solution 13

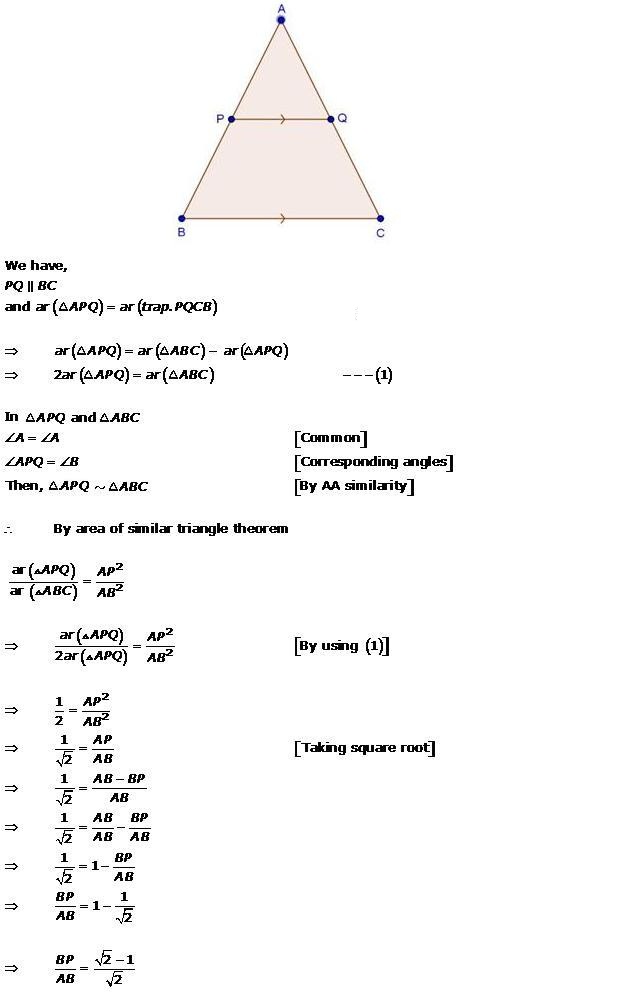
Solution 14

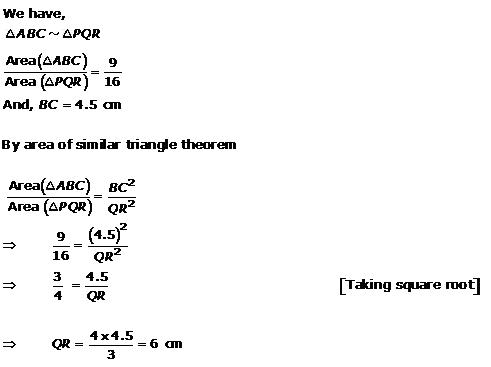
Solution 15



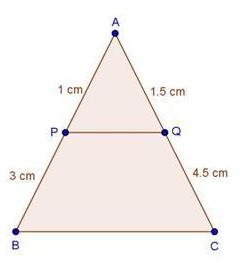
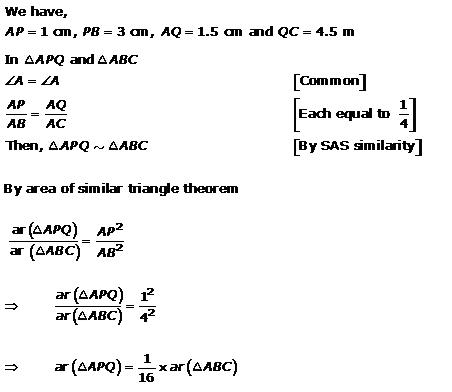
Solution 16



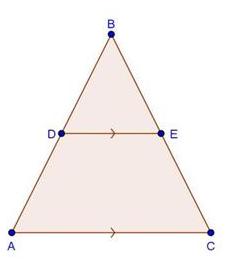
Solution 17



Solution 19


Since ABC and DBC are one same base,
Therefore ratio between their areas will be as ratio of their heights.
Let us draw two perpendiculars AP and DM on line BC.


In APO and DMO,
APO = DMO    (Each is90o)
AOP = DOM          (vertically opposite angles)
OAP = ODM         (remaining angle)
Therefore APO ~  DMO    (By AAA rule)

Solution 20



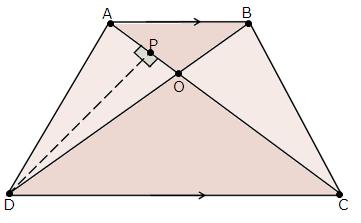
Solution 21



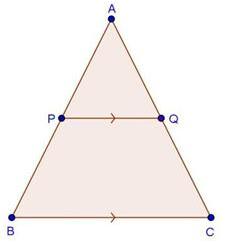
Solution 22



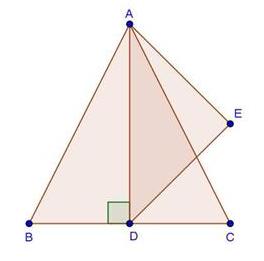
Solution 18

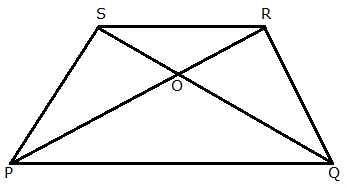
In trapezium PQRS, PQ || RS and PQ = 3RS.

 … (i)

In ∆POQ and ∆ROS,

∠SOR = ∠QOP … [Vertically opposite angles]

∠SRP = ∠RPQ … [Alternate angles]

∴ ∆POQ ∼ ∆ROS … [By AA similarity criteria]

Using the property of area of areas of similar triangles, we have

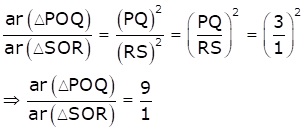
Hence, the ratio of the areas of triangles POQ and ROS is 9:1. 

Triangles Exercise Ex. 7.7

Solution 1

Solution 2(i)

Solution 2(ii)

Solution 2(iii)

Solution 2(iv)

Solution 3



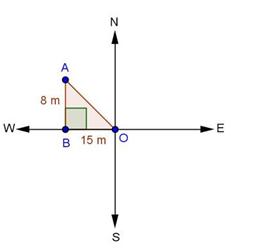
Solution 4



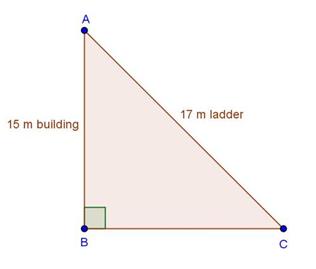
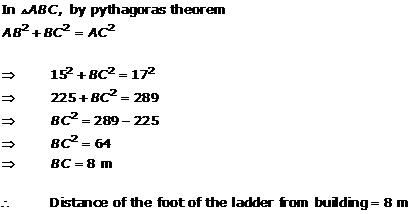
Solution 5


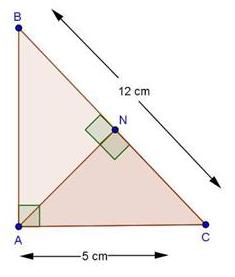
Let CD and AB be the poles of height 11 and 6 m.
Therefore CP = 11 - 6 = 5 m
From the figure we may observe that AP = 12m
In triangle APC, by applying Pythagoras theorem

Therefore distance between their tops = 13 m.

Solution 6



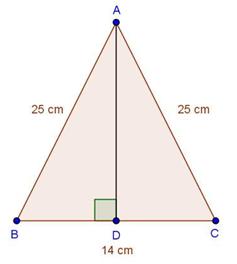
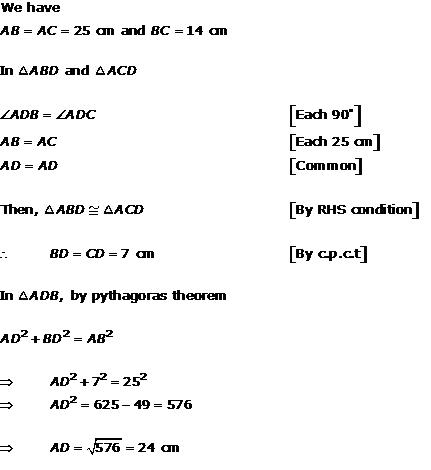
Solution 7



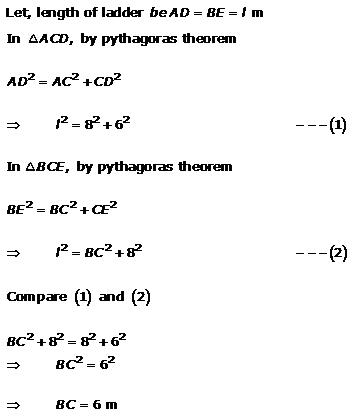
Solution 8



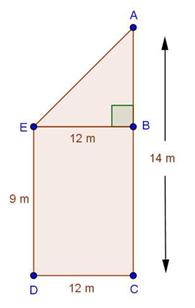
Solution 9

Solution 10



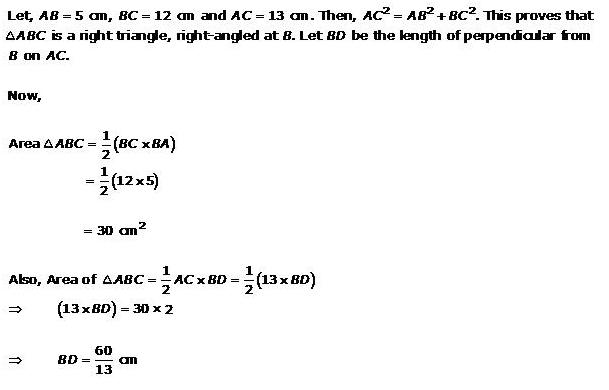
Solution 11



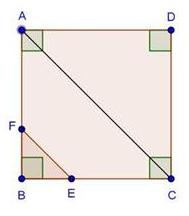
Solution 12



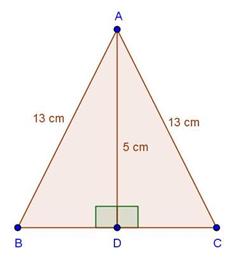
Solution 13



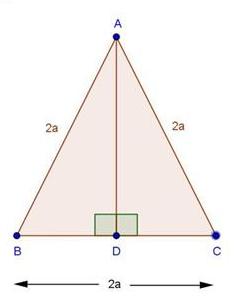
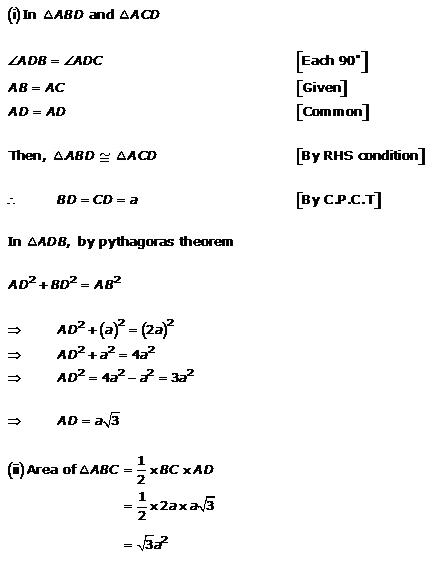
Solution 14



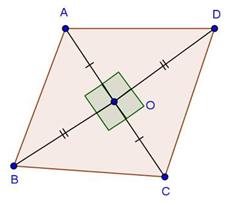
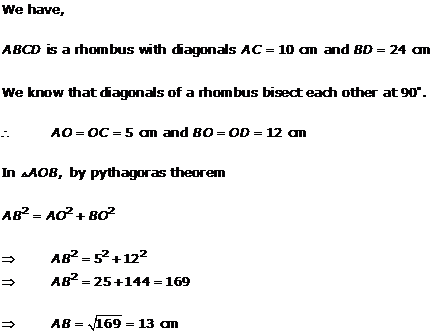
Solution 15



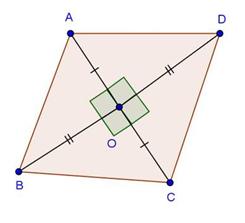
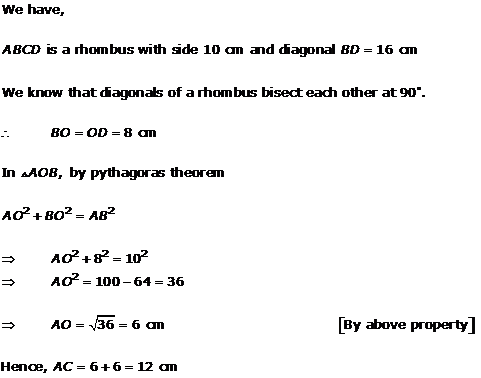
Solution 16



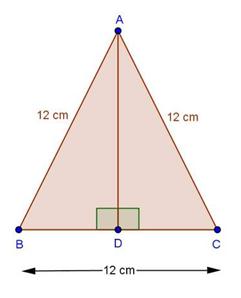
Solution 17

(i)

Solution 18



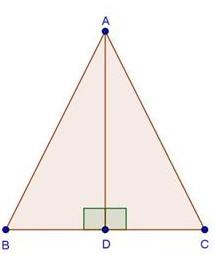
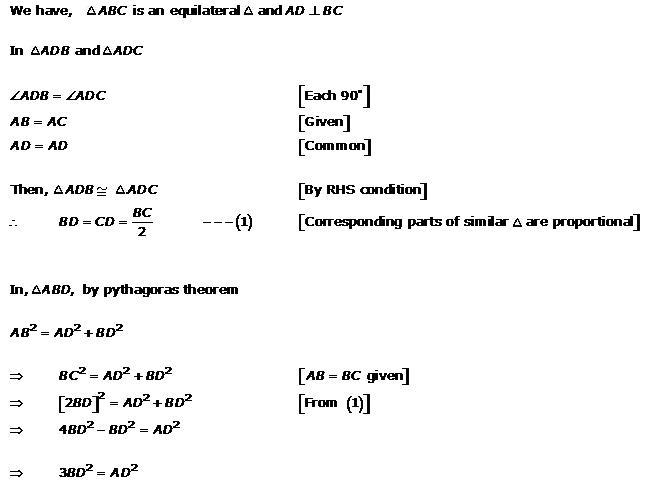
Solution 19



Solution 20



Solution 21

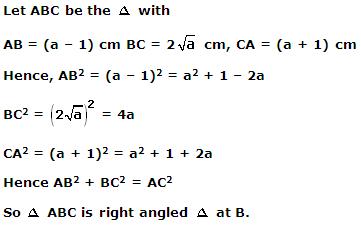
Solution 22



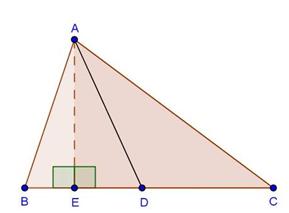
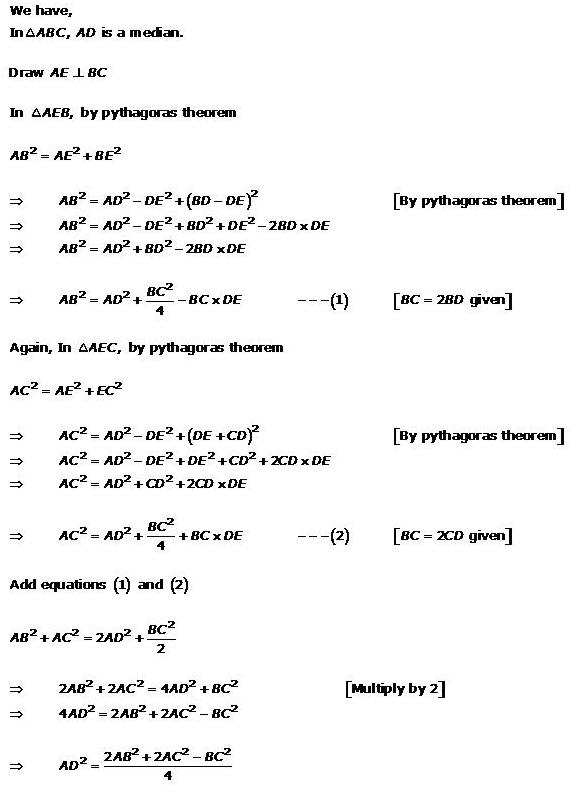
Solution 23



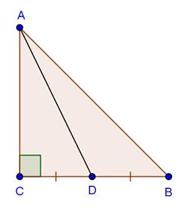
Solution 24

Solution 25



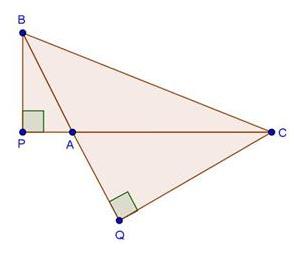
(i)

Solution 26



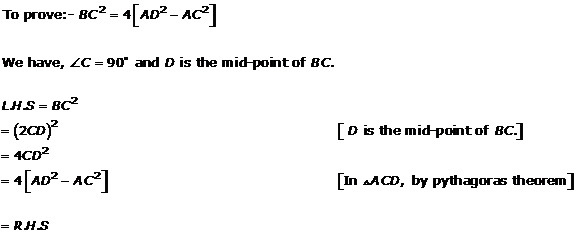
Solution 27



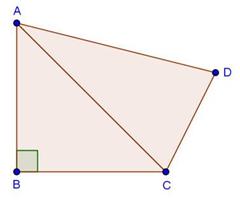
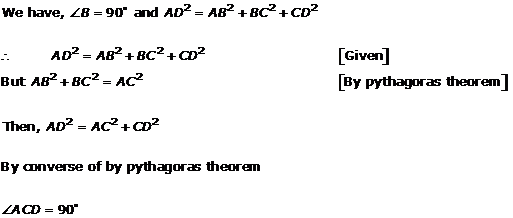
Solution 28



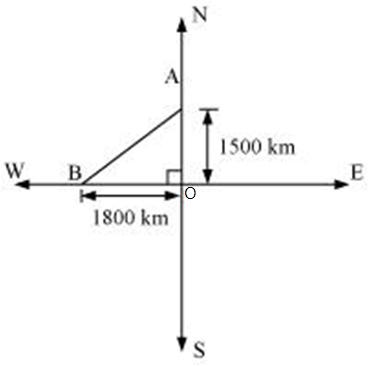
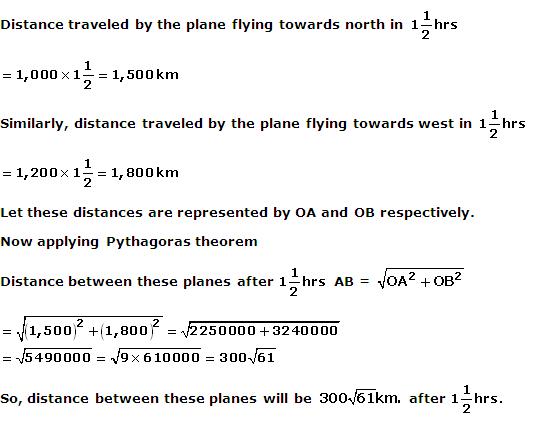
Triangles Exercise Rev. 7

Solution 1(i)

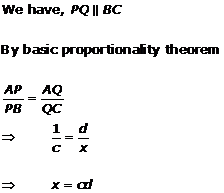
Solution 1(ii)

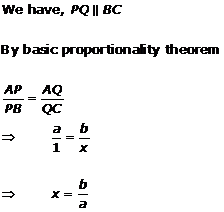
Solution 1(iii)

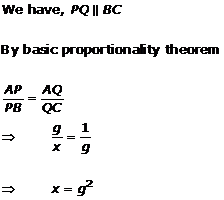
Solution 1(iv)

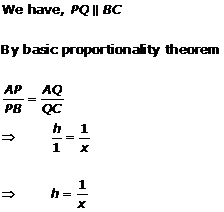
Solution 2

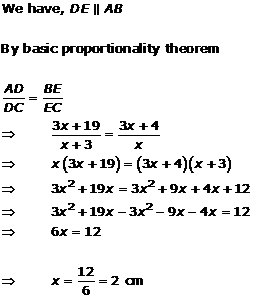
Solution 3



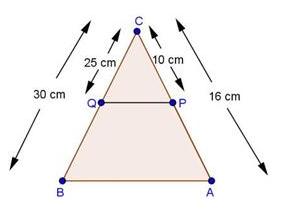
Solution 4

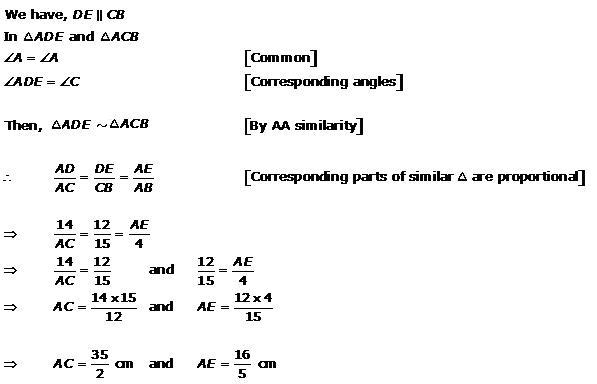
Solution 5

Solution 6



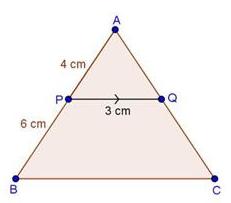
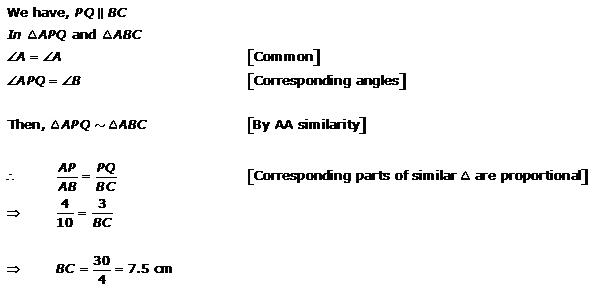
Solution 7(i)

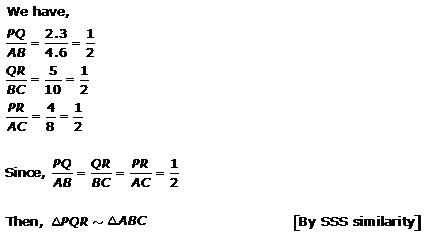
Solution 7(ii)

Solution 7(iii)

Solution 7(iv)

Incomplete question (two triangles are not given in the figure).

Solution 7(v)

Incomplete question (two triangles are not given in the figure).

Solution 7(vi)

Solution 8



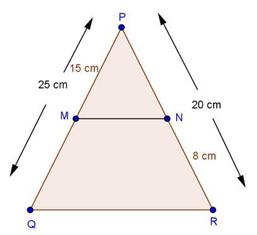
Solution 9



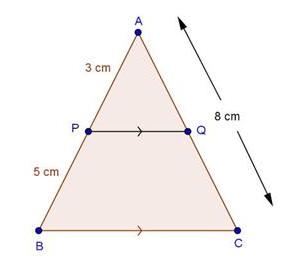
Solution 10

Solution 11



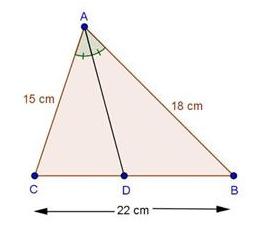
Solution 12

Solution 13

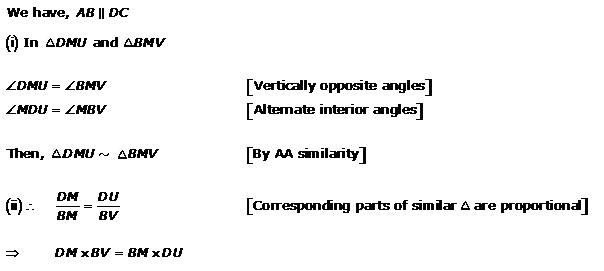
Solution 14



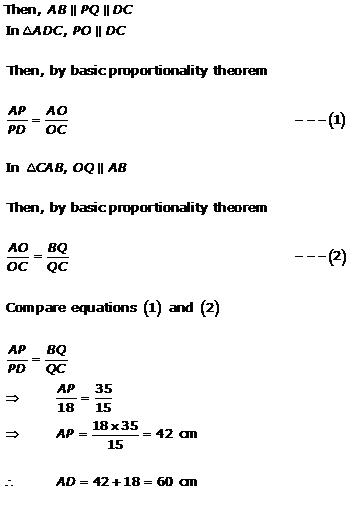
Solution 15



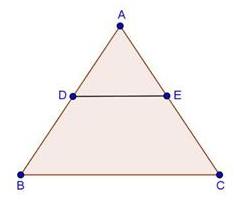
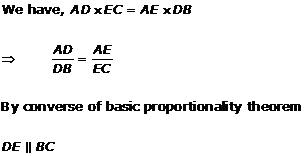
Solution 16



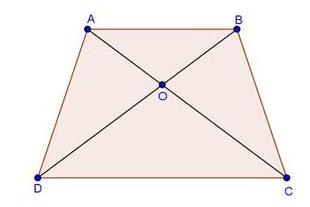
Solution 17



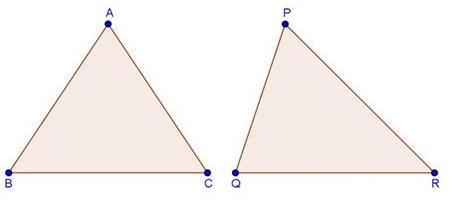
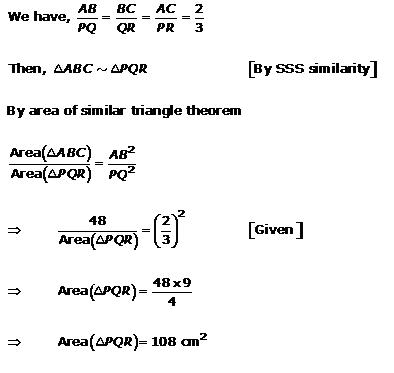
Solution 18

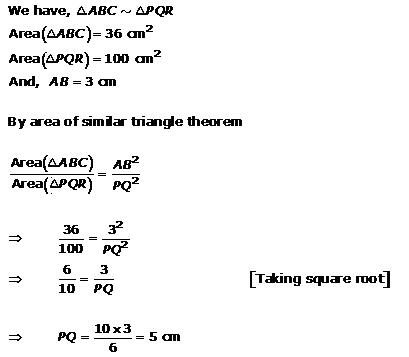
Solution 19

Solution 20

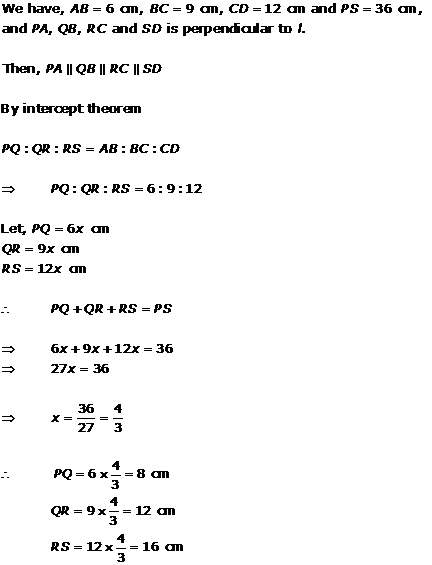
Solution 21




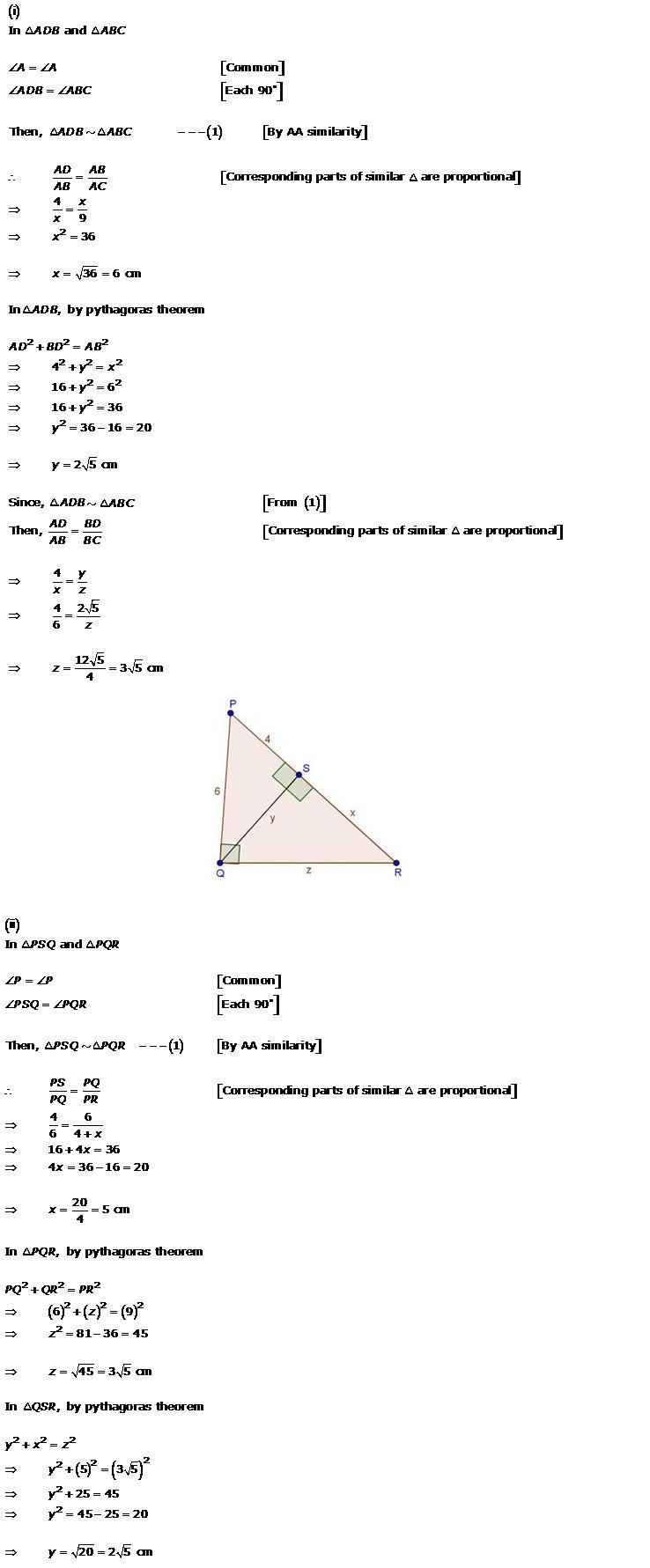
Solution 22



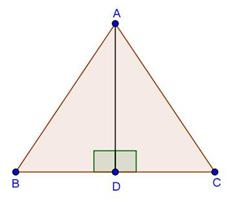
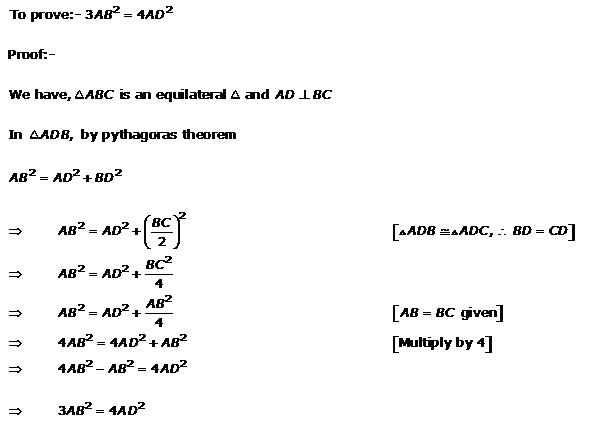
Solution 23



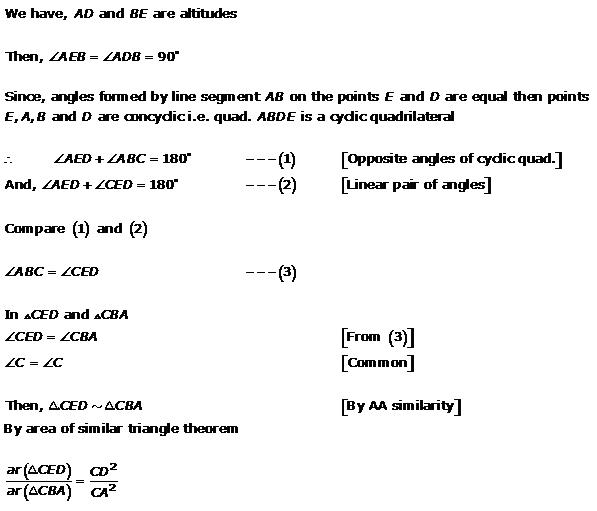
Solution 24



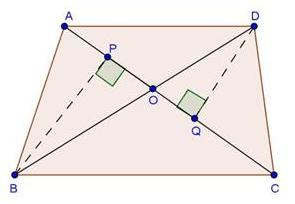
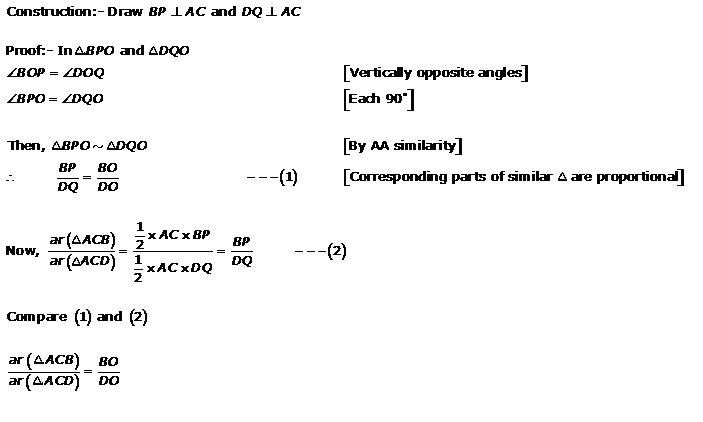
Solution 25



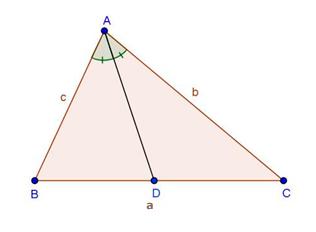
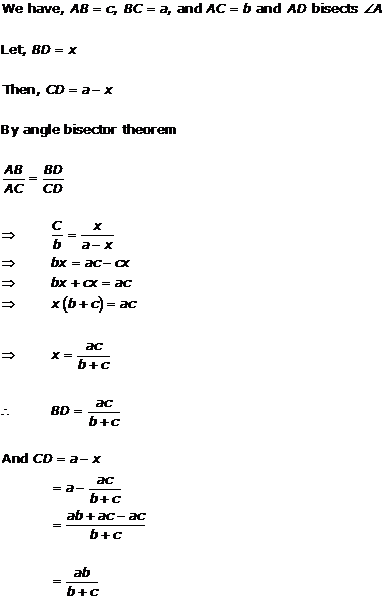
Solution 26

Solution 27



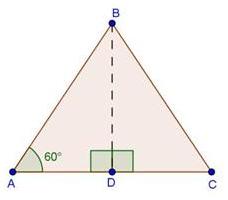
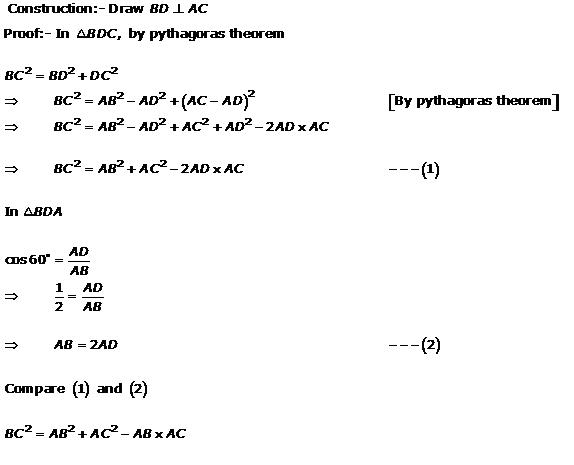
Solution 28



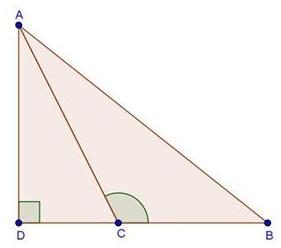
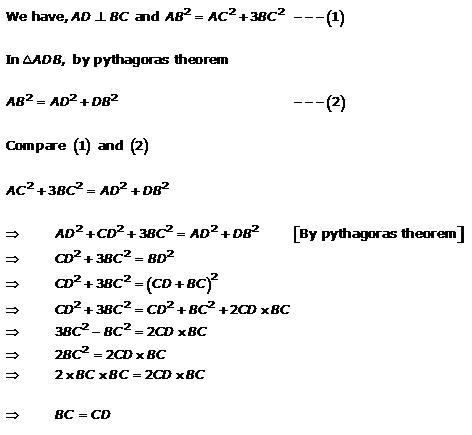
Solution 29



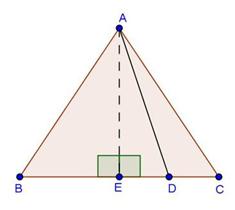
Solution 30



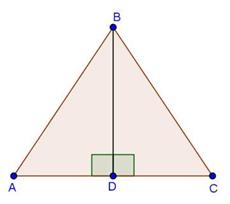
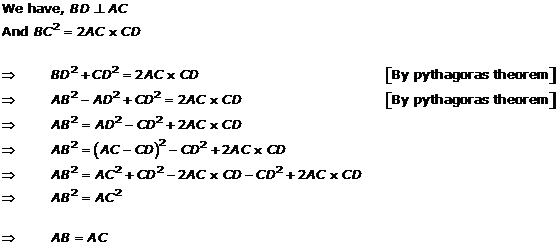
Solution 31



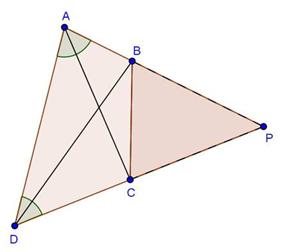
Solution 32



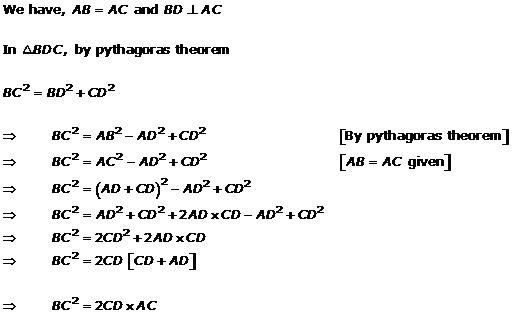
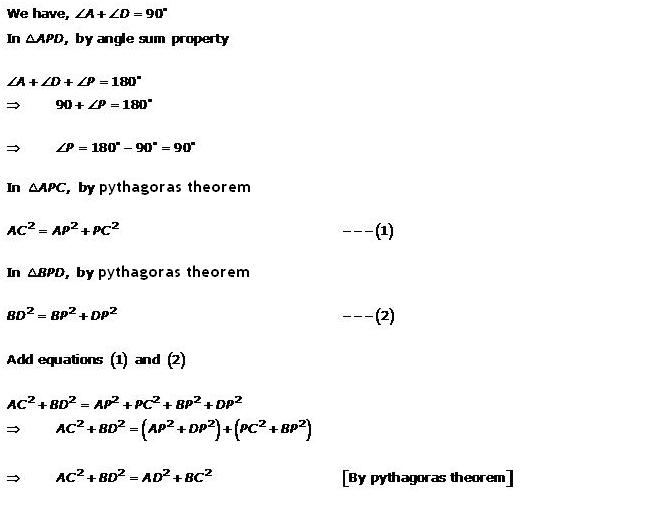
Solution 33



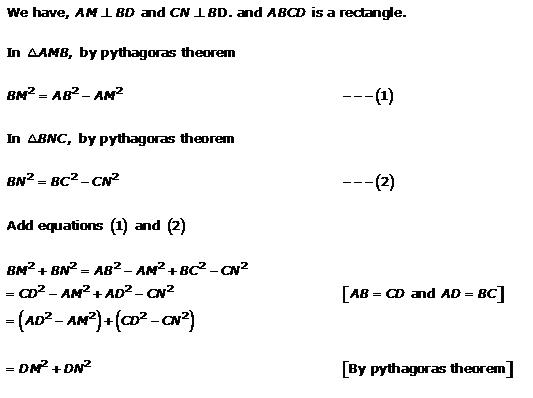
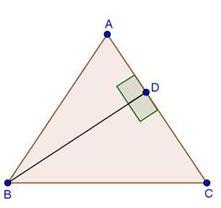
Solution 34



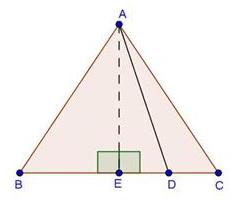
Solution 35



Solution 36



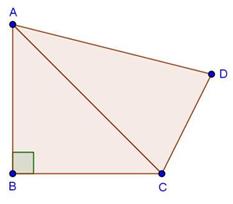
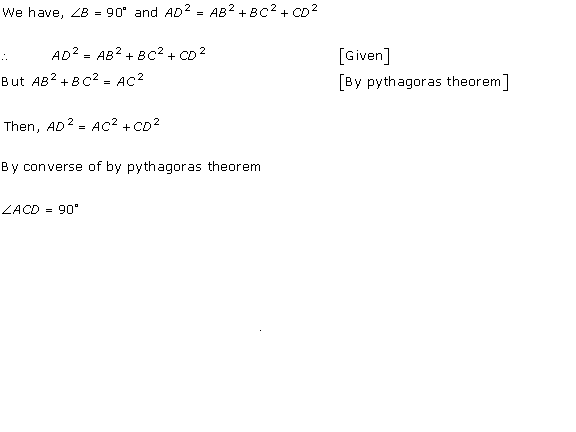
Solution 37



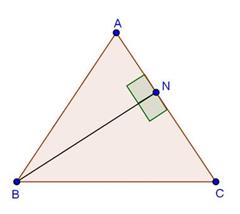
Solution 38

The given information can be represented by the figure given below.




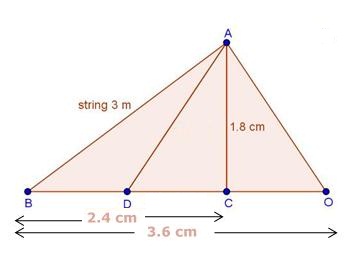
Triangles Exercise 7.131

Solution 1

We know if sides of two similar triangles are in ratio a:b then area of these triangles are in ratio a2b2

According to question, ratio of sides= 4:9

 Hence ratio of areas = 42:92

                             = 16:81

So, the correct option is (d).

Solution 2

begin mathsize 11px style table attributes columnalign left end attributes row cell If straight space areas straight space of straight space two straight space similar straight space triangles straight space are space in space the straight space ratio straight space straight a colon straight b straight space end cell row cell then straight space their straight space corresponding straight space sides straight space are straight space in space the straight space ratio square root of straight a colon square root of straight b end cell row cell Hence comma given space ratio space of space areas space is space 9 colon 16 end cell row cell so space ratio space of space corresponding space sides space is space square root of 3 colon square root of 16 end cell row cell space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space equals space 3 colon 4 end cell end table end style

So, the correct option is (a).

Solution 3

begin mathsize 11px style table attributes columnalign left end attributes row cell ratio text  of areas of triangle =  end text 144 over 81 end cell row cell ratio text  of their corresponding sides =  end text fraction numerator square root of 144 over denominator square root of 81 end fraction end cell row cell text                                                  =  end text 12 over 9 end cell row cell rightwards double arrow text   end text fraction numerator side text  of  end text straight capital delta text ABC end text over denominator side text  of  end text ΔDEF end fraction equals 12 over 9 end cell row cell rightwards double arrow text   end text fraction numerator 36 over denominator sides text  of  end text ΔDEF end fraction equals 12 over 9 end cell row cell rightwards double arrow text  side of  end text ΔDEF equals fraction numerator 36 straight x 9 over denominator 12 end fraction end cell row cell text                         = 27 cm end text end cell end table So comma space the space correct space option space is space left parenthesis straight c right parenthesis. end style

Triangles Exercise 7.132

Solution 4

begin mathsize 11px style table attributes columnalign left end attributes row cell Equilateral space triangles space are space also space similar space triangles. end cell row cell As space straight D space is space mid space point space of space BC space end cell row cell Hence space BC space equals space 2 space BD end cell row cell space space space space space rightwards double arrow straight space BC over BD equals 2 colon 1 end cell row cell ratio space of space corresponding space sides space are space 2 colon 1 end cell row cell ratio space of space areas space of space triangles space is space space 2 to the power of straight 2 colon 1 squared end cell row cell space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space equals space 4 colon 1 end cell end table So comma space the space correct space option space is space left parenthesis straight c right parenthesis. end style

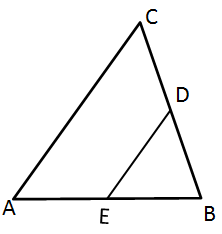
Solution 5

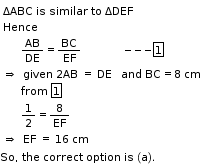
begin mathsize 11px style table attributes columnalign left end attributes row cell ΔABC space is space similar space to space ΔDEF end cell row Hence row cell space space space space space space AB over DE equals BC over EF space space space space space space space space space space space space space space minus negative negative box enclose 1 end cell row cell rightwards double arrow space space given space 2 AB space equals space DE space space space and space BC equals 8 space cm end cell row cell space space space space space space from space box enclose 1 end cell row cell space space space space space space 1 half equals 8 over EF end cell row cell rightwards double arrow space space EF space equals space 16 space cm end cell end table So comma space the space correct space option space is space left parenthesis straight a right parenthesis. end style

Solution 6

All these pairs of corresponding sides are in the same proportion so by SSS similarity criteria triangle ∆ABC are similar.

Given ratio of sides = 2.5

So, ratio of areas    = 22:52

                            = 4:25

So, the correct option is (b).

Solution 7

begin mathsize 11px style table attributes columnalign left end attributes row cell Given straight space AB over BX equals 4 end cell row cell XY parallel to BC space Hence end cell row cell angle AXY space equals space angle ABC space space space and end cell row cell angle AYX space equals space angle ACB end cell row cell By space AAA space similarity space criteria comma space triangles space are space similar end cell row cell Given space space AB over BX  =  4    ---- box enclose 1 end cell row cell       rightwards double arrow straight space AB over BX minus 1 equals 4 minus 1 end cell row cell       rightwards double arrow straight space fraction numerator AB minus BX over denominator BX end fraction equals 3 end cell row cell       rightwards double arrow straight space AX over BX equals 3 end cell row cell    space rightwards double arrow straight space BX over AX equals 1 third    ----- box enclose 2 end cell row cell multiply straight space box enclose 1 space space by space box enclose 2 end cell row cell          rightwards double arrow straight space AB over BX straight space cross times straight space BX over AX straight space cross times   4 cross times 1 third end cell row cell          rightwards double arrow    AB over AX  =  4 over 3 end cell row cell straight space rightwards double arrow Also straight space AB over AX equals AC over AY space space left parenthesis Since space XY parallel to BC right parenthesis end cell row cell        rightwards double arrow straight space 4 over 3 equals fraction numerator AY plus YC over denominator AY end fraction end cell row cell        rightwards double arrow    4 over 3 equals fraction numerator AY plus 2 over denominator AY end fraction end cell row cell        rightwards double arrow space 4 AY space equals space 3 AY plus 6 end cell row cell        rightwards double arrow straight space box enclose AY equals 6 space cm end enclose end cell end table So comma space the space correct space option space is space left parenthesis straight c right parenthesis. end style

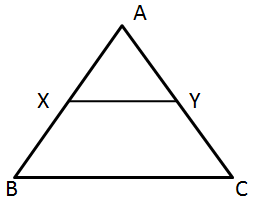
Solution 8

begin mathsize 11px style angle ACB equals angle DCE space left parenthesis common space angle right parenthesis angle ABC equals angle DEC space left parenthesis both space are space right space angles right parenthesis Since space DE parallel to AC comma space angle BAC equals angle EDC space left parenthesis corresponding space angles right parenthesis table attributes columnalign left end attributes row cell ΔABC straight space is space similar space to space ΔDEC end cell row cell by straight space AAA space similarity space criterion end cell row cell so space AB over DE straight space equals straight space BC over EC equals straight space AC over DC end cell row cell rightwards double arrow straight space 11 over 6 equals straight space fraction numerator BE space plus space EC over denominator EC end fraction end cell row cell rightwards double arrow straight space 11 over 6 equals straight space BE over EC plus 1 end cell row cell rightwards double arrow straight space 5 over 6 equals straight space BE over EC end cell row cell rightwards double arrow space EC space equals space fraction numerator BE straight space cross times space 6 over denominator 5 end fraction equals straight space fraction numerator 12 cross times 6 over denominator 5 end fraction end cell row cell rightwards double arrow space EC space equals space 14.4 space straight m end cell row cell In space ΔDEC space comma space DC to the power of straight 2 equals space DE to the power of straight 2 plus EC to the power of straight 2 end cell row cell space space space space space space space space space space space space space space space space space space space space rightwards double arrow space DC to the power of straight 2 equals 6 squared plus 14.4 squared rightwards double arrow DC equals 15.6 space straight m end cell row cell In space ΔABC space comma space AC to the power of straight 2 equals space AB to the power of straight 2 plus BC to the power of straight 2 end cell row cell space space space space space space space space space space space space space space space space space space space space rightwards double arrow space AC to the power of straight 2 equals 11 squared plus 26.4 squared rightwards double arrow AC equals 28.6 space straight m end cell row cell space space space space space space space space space space space space space space space space space AD space equals space AC space minus space DC equals 13 space straight m end cell end table So comma space the space correct space option space is space left parenthesis straight c right parenthesis. end style

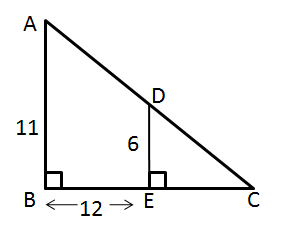
Solution 9

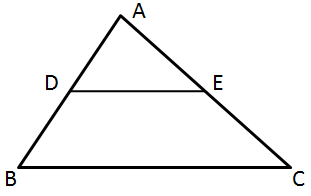
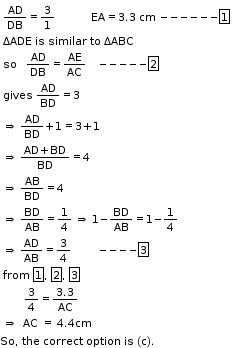
begin mathsize 11px style table attributes columnalign left end attributes row cell AD over DB equals 3 over 1 space space space space space space space space space space space space EA equals 3.3 space cm space minus negative negative negative negative negative box enclose 1 end cell row cell ΔADE space is space similar space to space ΔABC end cell row cell so space space space AD over DB equals AE over AC space space space space minus negative negative negative negative box enclose 2 end cell row cell gives straight space AD over BD equals 3 end cell row cell rightwards double arrow straight space AD over BD plus 1 equals 3 plus 1 end cell row cell rightwards double arrow straight space fraction numerator AD plus BD over denominator BD end fraction equals 4 end cell row cell rightwards double arrow straight space AB over BD equals 4 end cell row cell rightwards double arrow straight space BD over AB equals 1 fourth space rightwards double arrow straight space 1 minus BD over AB equals 1 minus 1 fourth end cell row cell rightwards double arrow straight space AD over AB equals 3 over 4 space space space space space space space space space minus negative negative negative box enclose 3 end cell row cell from straight space box enclose 1 comma straight space box enclose 2 comma straight space box enclose 3 end cell row cell space space space space space space space 3 over 4 equals fraction numerator 3.3 over denominator AC end fraction end cell row cell rightwards double arrow space space AC space equals space 4.4 cm end cell end table So comma space the space correct space option space is space left parenthesis straight c right parenthesis. end style

Solution 10

begin mathsize 11px style table attributes columnalign left end attributes row cell AB over ED equals AC over EF end cell row cell ΔABC space and space ΔEDF space are space similar space by space SAS space criterion end cell row cell so space angle straight B equals angle straight D end cell row cell given straight space angle straight D equals 180 degree minus 40 degree minus 65 degree end cell row cell space space space space space space equals space 75 degree end cell row cell so straight space box enclose angle straight B equals 75 degree end enclose end cell end table So comma space the space correct space option space is space left parenthesis straight c right parenthesis. end style

Solution 11

begin mathsize 11px style table attributes columnalign left end attributes row cell As straight space ΔABC straight space and straight space ΔDEF space are straight space similar colon end cell row cell So space space space angle straight A straight equals angle straight D    &    angle straight C equals angle straight F end cell row cell rightwards double arrow straight space angle straight D space equals space 47 degree end cell row cell so comma straight space angle straight F space equals space 180 degree minus 83 degree minus 47 degree end cell row cell           =  50 degree end cell row cell so     box enclose angle straight C space equals space 50 degree end enclose end cell end table So comma space the space correct space option space is space left parenthesis straight a right parenthesis. end style

Solution 12

begin mathsize 11px style table attributes columnalign left end attributes row cell FE parallel to BC end cell row cell and space straight F comma space straight E space are space mid space points end cell row cell so space EF space equals space 1 half BC end cell row cell similarly space DE space equals space 1 half AB end cell row cell space space space space space space space & space space space FD space equals space 1 half AC end cell row cell So straight space ΔABC space and space ΔDEF space are space similar space and space their space sides space are space in end cell row cell ratio space 2 colon 1 end cell row cell Hence space ratio space of space areas space 2 to the power of straight 2 colon 1 squared end cell row cell space space space space space space space space space space space space space space space space space space space space space space space space space rightwards double arrow space 4 colon 1 end cell row cell So space ratio space of space areas space of space ΔDEF space and space ΔABC space is space 1 colon 4 end cell end table So comma space the space correct space option space is space left parenthesis straight a right parenthesis. end style

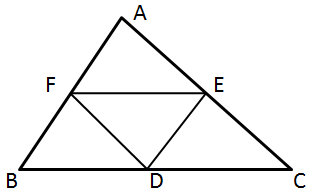
Solution 13

begin mathsize 11px style table attributes columnalign left end attributes row cell BD squared plus AD squared equals AB squared       --- box enclose 1 end cell row cell DC squared plus AD squared equals AC squared       --- box enclose 2 end cell row cell ΔABC space is space equilateral comma space AB space equals space BC space equals space AC space equals space straight a end cell row cell space Let space space space space space space BD space equals space straight x space then space DC space equals space BC space minus space BD end cell row cell                                     space space space space space space =  straight a minus straight x end cell row cell from straight space box enclose 1    & straight space box enclose 2 end cell row cell     straight x squared plus AD squared equals straight a squared    space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space and space space space space space space space space space space space space space space space left parenthesis straight a minus straight x right parenthesis squared plus AD squared equals straight a squared end cell row cell rightwards double arrow left parenthesis straight a minus square root of straight a squared minus AD squared end root right parenthesis squared plus AD squared equals straight a squared                space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space rightwards double arrow left parenthesis straight a minus straight x right parenthesis squared equals straight a squared minus AD squared end cell row cell rightwards double arrow straight a squared plus straight a squared minus AD squared minus 2 straight a square root of straight a squared minus AD squared end root plus AD squared equals straight a squared space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space rightwards double arrow straight a minus straight x equals square root of straight a squared minus AD squared end root end cell row cell rightwards double arrow straight a squared equals 2 straight a square root of straight a squared minus AD squared end root                              space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space rightwards double arrow space straight x space equals space straight a minus square root of straight a squared minus AD squared end root end cell row cell rightwards double arrow straight a to the power of 4 equals 4 straight a squared left parenthesis straight a squared minus AD squared right parenthesis end cell row cell rightwards double arrow straight a squared equals 4 left parenthesis straight a squared minus AD squared right parenthesis end cell row cell rightwards double arrow straight a to the power of 4 equals 4 straight a squared minus 4 AD squared end cell row cell rightwards double arrow 4 AD squared equals 3 straight a squared end cell row cell rightwards double arrow 4 AD squared equals 3 AB squared end cell end table So comma space the space correct space option space is space left parenthesis straight c right parenthesis. end style

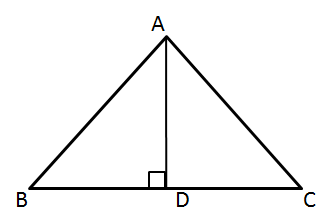
Solution 14

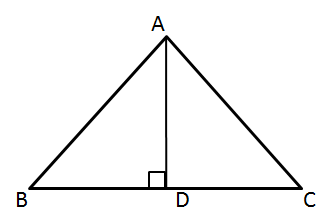
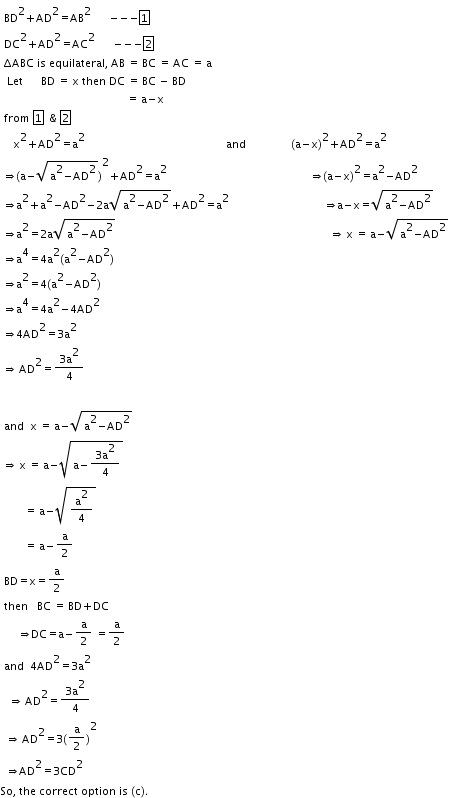
Error converting from MathML to accessible text.

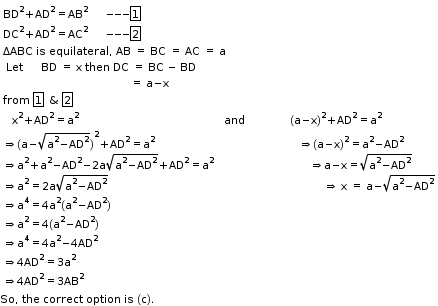
Solution 15

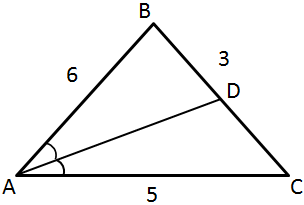
begin mathsize 11px style By space angle space bisector space theorem table attributes columnalign left end attributes row cell text     end text AC over AB equals CD over DB end cell row cell rightwards double arrow text   end text 5 over 6 equals CD over 3 end cell row cell rightwards double arrow CD equals 5 over 2 equals 2.5 cm end cell end table So comma space the space correct space option space is space left parenthesis straight b right parenthesis. end style

Solution 16

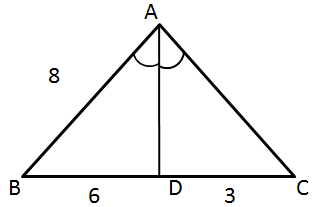
begin mathsize 11px style table attributes columnalign left end attributes row cell By space angle space bisector space theorem end cell row cell space space space AC over AB equals CD over BD end cell row cell rightwards double arrow AC over 8 equals 3 over 6 end cell row cell rightwards double arrow AC over 8 equals 1 half end cell row cell rightwards double arrow AC equals 4 space cm end cell end table So comma space the space correct space option space is space left parenthesis straight a right parenthesis. end style

Solution 17

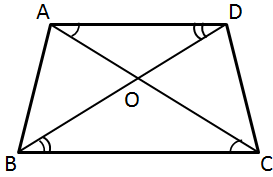
Error converting from MathML to accessible text.

Triangles Exercise 7.133

Solution 18

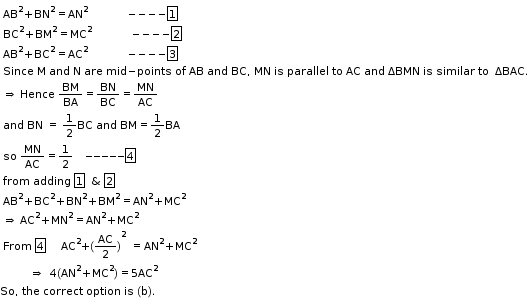
begin mathsize 11px style table attributes columnalign left end attributes row cell AB squared plus BN squared equals AN squared space space space space space space space space space space space space space minus negative negative negative box enclose 1 end cell row cell BC squared plus BM squared equals MC squared space space space space space space space space space space space space space minus negative negative negative box enclose 2 end cell row cell AB squared plus BC squared equals AC squared space space space space space space space space space space space space space minus negative negative negative box enclose 3 end cell row cell Since space straight M space and space straight N space are space mid minus points space of space AB space and space BC comma space MN space is space parallel space to space AC space and space ΔBMN space is space similar space to space space ΔBAC. end cell row cell rightwards double arrow space Hence space BM over BA equals BN over BC equals MN over AC end cell row cell and space BN space equals space 1 half BC space and space BM equals 1 half BA end cell row cell so straight space MN over AC equals 1 half     ----- box enclose 4 end cell row cell from space adding space box enclose 1   &  box enclose 2 end cell row cell AB squared plus BC squared plus BN squared plus BM squared equals AN squared plus MC squared end cell row cell rightwards double arrow space AC squared plus MN squared equals AN squared plus MC squared end cell row cell From straight space box enclose 4 space space space space space AC squared plus left parenthesis AC over 2 right parenthesis to the power of straight 2   =  AN squared plus MC squared end cell row cell           rightwards double arrow    4 left parenthesis AN squared plus MC squared right parenthesis equals 5 AC squared end cell end table So comma space the space correct space option space is space left parenthesis straight b right parenthesis. end style

Solution 19

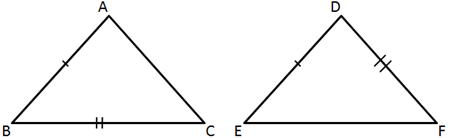
 For triangles to be similar by SAS

∠B = ∠D

So, the correct option is (c).

Solution 20

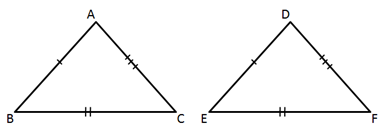
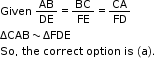
begin mathsize 11px style Given text   end text AB over DE equals BC over FE equals CA over FD ΔCAB tilde ΔFDE So comma space the space correct space option space is space left parenthesis straight a right parenthesis. end style

Solution 21

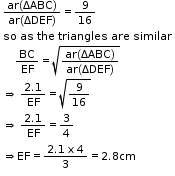
begin mathsize 11px style table attributes columnalign left end attributes row cell fraction numerator ar left parenthesis ΔABC right parenthesis over denominator ar left parenthesis ΔDEF right parenthesis end fraction equals 9 over 16 end cell row cell so space as space the space triangles space are space similar end cell row cell      BC over EF equals square root of fraction numerator ar left parenthesis ΔABC right parenthesis over denominator ar left parenthesis ΔDEF right parenthesis end fraction end root end cell row cell rightwards double arrow straight space fraction numerator 2.1 over denominator EF end fraction equals square root of 9 over 16 end root end cell row cell rightwards double arrow straight space fraction numerator 2.1 over denominator EF end fraction equals 3 over 4 end cell row cell rightwards double arrow EF equals fraction numerator 2.1 space straight x space 4 over denominator 3 end fraction equals 2.8 cm end cell end table end style

So, the correct option is (a).

Solution 22

begin mathsize 11px style table attributes columnalign left end attributes row cell AB equals AC end cell row cell and space space AB space equals space 4 square root of 2 space cm end cell row cell also space space space AB to the power of straight 2 plus AC to the power of straight 2 equals BC to the power of straight 2 end cell row cell rightwards double arrow space space space 2 AB to the power of straight 2 equals BC to the power of straight 2 end cell row cell rightwards double arrow space space BC space equals space square root of 2 straight space AB end cell row cell space space space space space space space space space space space equals space square root of 2 space straight x space 4 square root of 2 end cell row cell space space space space space space space space space space space equals space 8 space cm end cell end table So comma space the space correct space option space is space left parenthesis straight b right parenthesis. end style

Solution 23

begin mathsize 11px style table attributes columnalign left end attributes row cell He space is space AC space straight m space for end cell row cell and space space AC to the power of straight 2 equals AB squared plus BC squared end cell row cell space space space space space space space space space space space space space space equals space 24 to the power of straight 2 plus 7 squared end cell row cell space space space space space space space space space space space space space space equals space 576 space plus space 49 end cell row cell space space space space space space space space space space space space space space equals space 625 end cell row cell space space space space space space space space AC space space equals space square root of 625 end cell row cell space space space space space space space space AC space space equals space 25 straight m end cell end table So comma space the space correct space option space is space left parenthesis straight c right parenthesis. end style

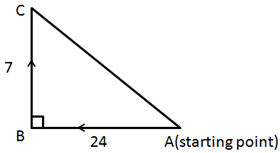
Solution 24

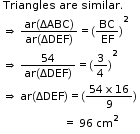
begin mathsize 11px style table attributes columnalign left end attributes row cell Triangles space are space similar. end cell row cell rightwards double arrow space fraction numerator ar left parenthesis ΔABC right parenthesis over denominator ar left parenthesis ΔDEF right parenthesis end fraction equals left parenthesis BC over EF right parenthesis squared end cell row cell rightwards double arrow straight space fraction numerator 54 over denominator ar left parenthesis ΔDEF right parenthesis end fraction equals left parenthesis 3 over 4 right parenthesis squared end cell row cell rightwards double arrow straight space ar left parenthesis ΔDEF right parenthesis equals left parenthesis fraction numerator 54 straight space straight x straight space 16 over denominator 9 end fraction right parenthesis end cell row cell space space space space space space space space space space space space space space space space space space space space equals space 96 space cm to the power of straight 2 end cell end table end style

So, the correct option is (b).

Solution 25

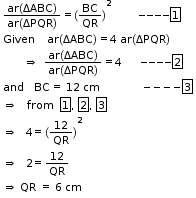
begin mathsize 11px style table attributes columnalign left end attributes row cell fraction numerator ar left parenthesis ΔABC right parenthesis over denominator ar left parenthesis ΔPQR right parenthesis end fraction equals left parenthesis BC over QR right parenthesis squared          ---- box enclose 1 end cell row cell Given      ar left parenthesis ΔABC right parenthesis equals 4 straight space ar left parenthesis ΔPQR right parenthesis end cell row cell         rightwards double arrow    fraction numerator ar left parenthesis ΔABC right parenthesis over denominator ar left parenthesis ΔPQR right parenthesis end fraction equals 4       ---- box enclose 2 end cell row cell and    BC equals space 12 space cm space space space space space space space space space space space space space space minus negative negative negative box enclose 3 end cell row cell rightwards double arrow    from space space box enclose 1 comma straight space box enclose 2 ,  box enclose 3 end cell row cell rightwards double arrow     4 =  left parenthesis 12 over QR right parenthesis squared end cell row cell rightwards double arrow     2 =  12 over QR end cell row cell rightwards double arrow space QR space equals space 6 space cm end cell end table end style

So, the correct option is (c).

Solution 26

begin mathsize 11px style table attributes columnalign left end attributes row cell space space space fraction numerator area space of space straight capital delta subscript straight 1 over denominator area space of space straight capital delta subscript 2 end fraction equals left parenthesis fraction numerator median space of space straight capital delta subscript straight 1 straight space over denominator median space of space straight capital delta subscript 2 end fraction right parenthesis squared end cell row cell rightwards double arrow 121 over 64 equals left parenthesis fraction numerator 12.1 over denominator straight M end fraction right parenthesis squared end cell row cell rightwards double arrow space 12.1 divided by straight M space equals 11 over 8 end cell row cell rightwards double arrow straight M equals fraction numerator 12.1 cross times straight space 8 over denominator 11 end fraction end cell row cell space space space space space space equals space 8.8 space cm end cell end table So comma space the space correct space option space is space left parenthesis straight b right parenthesis. end style

Solution 27

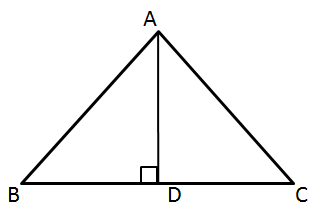
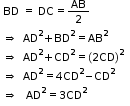
begin mathsize 11px style table attributes columnalign left end attributes row cell BD straight space equals straight space DC equals AB over 2 end cell row cell rightwards double arrow space space AD to the power of straight 2 plus BD squared equals AB squared end cell row cell rightwards double arrow space space AD to the power of straight 2 plus CD squared equals left parenthesis 2 CD right parenthesis squared straight space end cell row cell rightwards double arrow space space AD to the power of straight 2 equals 4 CD squared minus CD squared straight space end cell row cell rightwards double arrow space space space AD to the power of straight 2 equals 3 CD squared end cell end table end style

So, the correct option is (c).

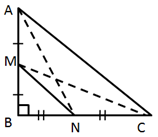
Solution 28

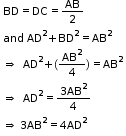
begin mathsize 11px style table attributes columnalign left end attributes row cell BD equals DC equals AB over 2 end cell row cell and text  AD end text to the power of text 2 end text end exponent plus BD squared equals AB squared end cell row cell rightwards double arrow text   AD end text to the power of text 2 end text end exponent plus left parenthesis AB squared over 4 right parenthesis equals text AB end text to the power of text 2 end text end exponent end cell row cell rightwards double arrow text   AD end text to the power of text 2 end text end exponent equals fraction numerator 3 AB squared over denominator 4 end fraction end cell row cell rightwards double arrow text   end text 3 AB squared equals 4 AD squared end cell end table end style

So, the correct option is (b).

Solution 29

begin mathsize 11px style table attributes columnalign left end attributes row cell AC equals BC space space space and space AB to the power of straight 2 equals 2 AC squared end cell row cell space space space space space space space space space space space space space space space space space space space space space AB to the power of straight 2 equals AC squared plus AC squared end cell row cell space space space space space space space space space space space space space space space space space space space space space AB to the power of straight 2 equals AC squared plus BC squared end cell row cell It space means space angle straight C space is space 90 to the power of straight 0 end cell row cell So comma space the space correct space option space is space left parenthesis straight c right parenthesis. end cell end table end style

Solution 30

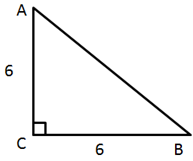
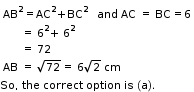
begin mathsize 11px style table attributes columnalign left end attributes row cell AB squared equals AC squared plus BC squared space space space and space AC space equals space BC equals 6 end cell row cell space space space space space space equals space 6 to the power of straight 2 plus space 6 to the power of straight 2 end cell row cell space space space space space space equals space 72 end cell row cell AB space equals space square root of 72 equals space 6 square root of 2 space cm end cell end table So comma space the space correct space option space is space left parenthesis straight a right parenthesis. end style

Triangles Exercise 7.134

Solution 31

begin mathsize 11px style angle straight A equals angle straight E space and space angle straight B equals angle straight F comma space so space 3 rd space angle space of space t h e space t riangle space must space be space equal. space Hence space by space AAA space similarity space criteria space increment ABC space ∿ space increment EFD Hence AB over EF equals BC over FD equals AC over ED On space seeing space options comma space only space left parenthesis straight b right parenthesis space is space incorrect. space So space false space option space is space left parenthesis straight b right parenthesis. end style

So, the correct option is (b).

Solution 32

begin mathsize 11px style table attributes columnalign left end attributes row cell In straight space ΔABC comma space space angle straight A space plus space angle straight B plus angle straight c equals 180 degree end cell row cell thin space thin space thin space thin space thin space thin space thin space rightwards double arrow space space angle straight A space plus space 30 plus 20 equals 180 end cell row cell space space space space space space space rightwards double arrow straight space angle straight A space equals space 130 degree end cell row cell also straight space AB over EF equals AC over DE space space and space angle straight A space equals space angle straight E end cell row cell Hence space by space SAS space similarity space criterion comma space ΔBAC tilde ΔFED end cell row cell and space space angle straight B space equals space angle straight F space space space and space space angle straight C space equals space angle straight D end cell row cell rightwards double arrow space space angle straight F space equals 30 degree space space and space angle straight D space equals space 20 degree end cell end table So comma space the space correct space option space is space left parenthesis straight b right parenthesis. end style

Solution 33

begin mathsize 11px style table attributes columnalign left end attributes row cell AB straight space equals straight space AD plus DB end cell row cell space space space space equals straight x plus 3 straight space plus straight space 3 straight x plus 19 end cell row cell AB straight space equals straight space 4 straight x straight space plus straight space 22 end cell row cell AC straight space equals straight space AE straight space plus straight space EC equals straight space straight x straight space plus straight space 3 straight x straight space plus 4 end cell row cell AC straight space equals straight space 4 straight x straight space plus 4 end cell row cell table attributes columnalign left end attributes row cell space DE parallel to AE end cell row cell So comma straight space angle ADE space equals space angle straight B space space and space angle AED space equals space angle straight C space end cell row cell Hence space by space AA comma space space ΔABC tilde ΔADE end cell row cell so comma space AD over AB equals AE over AC end cell row cell rightwards double arrow space space fraction numerator straight x plus 3 over denominator 4 straight x plus 22 end fraction equals fraction numerator straight x over denominator 4 straight x plus 4 end fraction end cell row cell rightwards double arrow space 4 straight x to the power of straight 2 plus 16 straight x plus 12 equals 4 straight x squared plus 22 straight x end cell row cell rightwards double arrow space 6 straight x space equals space 12 end cell row cell rightwards double arrow space straight x equals space 2 end cell end table end cell end table So comma space the space correct space option space is space left parenthesis straight d right parenthesis. end style

Solution 34

begin mathsize 11px style In space increment ADE space and space increment ABC space angle ADE space equals space angle ABC space left parenthesis given right parenthesis angle DAE space equals space angle BAC space left parenthesis common space angle right parenthesis Hence space increment ADE space and space increment ABC space left parenthesis By space AA space criterion right parenthesis table attributes columnalign left end attributes row cell So comma text   end text AD over AB equals AE over AC end cell row cell rightwards double arrow text   end text fraction numerator AD over denominator AD plus DB end fraction equals fraction numerator AE over denominator AE plus EC end fraction end cell row cell rightwards double arrow text   end text 2 over 5 equals fraction numerator 3 over denominator 3 plus EC end fraction end cell row cell rightwards double arrow text  6+2EC = 15 end text end cell row cell rightwards double arrow text  EC= end text 9 over 2 end cell end table So comma space the space correct space option space is space left parenthesis straight c right parenthesis. end style

Solution 35

begin mathsize 11px style table attributes columnalign left end attributes row cell PQ parallel to BD parallel to SR end cell row cell So comma text   end text straight capital delta text ASR end text tilde straight capital delta text ABD end text end cell row cell text Hence  end text AR over AD equals RS over DB end cell row cell text     end text rightwards double arrow text    end text 3 over 6 equals straight y over straight x end cell row cell text    end text rightwards double arrow text    x=2y ---- end text box enclose 1 end cell row cell Only text   end text option text   end text left parenthesis straight d right parenthesis satisfies text     end text box enclose 1 end cell end table So comma space the space correct space option space is space left parenthesis straight d right parenthesis. end style

Triangles Exercise 7.135

Solution 36

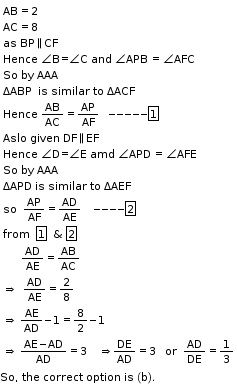
begin mathsize 11px style table attributes columnalign left end attributes row cell AB equals 2 end cell row cell AC equals 8 end cell row cell as text   end text BP parallel to CF end cell row cell Hence text   end text angle text B= end text angle text C and  end text angle text APB =  end text angle AFC end cell row cell So text  by AAA end text end cell row cell straight capital delta text ABP  is similar to  end text straight capital delta text ACF end text end cell row cell text Hence  end text AB over AC equals AP over AF text    ----- end text box enclose 1 end cell row cell Aslo text  given DF end text parallel to text EF end text end cell row cell text Hence  end text angle text D= end text angle straight E text  amd  end text angle text APD =  end text angle text AFE end text end cell row cell text So by AAA end text end cell row cell straight capital delta text APD is similar to  end text straight capital delta text AEF end text end cell row cell text so   end text AP over AF equals AD over AE text     ---- end text box enclose 2 end cell row cell from text    end text box enclose 1 text   &  end text box enclose 2 end cell row cell text        end text AD over AE equals AB over AC end cell row cell rightwards double arrow text    end text AD over AE equals 2 over 8 end cell row cell rightwards double arrow text   end text AE over AD minus 1 equals 8 over 2 minus 1 end cell row cell rightwards double arrow text   end text fraction numerator AE minus AD over denominator AD end fraction equals 3 text      end text rightwards double arrow DE over AD equals 3 text    or   end text AD over DE equals 1 third end cell end table So comma space the space correct space option space is space left parenthesis straight b right parenthesis. end style

Solution 37

                 

begin mathsize 11px style OA space and space OB space are space radius space of space circle space space space So space OA space equals space OB equals space 10 space cm space given space angle AOB space equals space 90 degree space space Hence space increment AOB space is space straight a space right space angled space triangle. end style

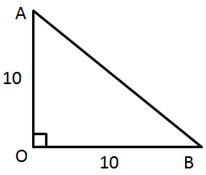
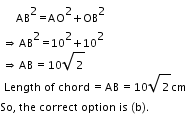
Error converting from MathML to accessible text.

Solution 38

begin mathsize 11px style table attributes columnalign left end attributes row cell from space figure space 1 space space space tanθ space equals space 20 over 10 equals 2 space space space space space space space space minus negative negative box enclose 1 end cell row cell from space figure space 2 space space space tanθ space equals space straight h over 50 space space space space space space space space minus negative negative box enclose 2 space space space space space end cell row cell from space space box enclose 1 space & space box enclose 2 end cell row cell space space space space space space space space space straight h over 50 equals 2 space space equals space straight h equals 100 space straight m end cell end table So comma space the space correct space option space is space left parenthesis straight a right parenthesis. end style

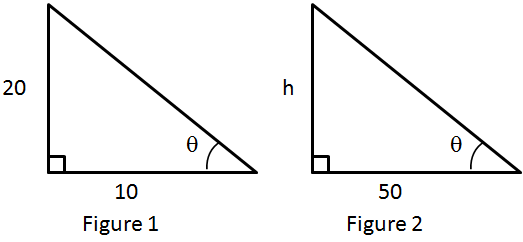
Solution 39

begin mathsize 11px style As space given space angles space are space equal. space So space by space AAA space criteria space the space given space triangles space are space similar. space Given space ratio space of space area space is space 16 colon 25. space So comma space ratio space of space this space corresponding space heights space table attributes columnalign left end attributes row cell equals text   end text square root of 16 colon square root of 25 end cell row cell equals text   end text 4 colon 5 end cell end table end style

So, the correct option is (a).

Solution 40

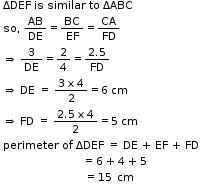
Error converting from MathML to accessible text.

So, the correct option is (b).

Triangles Exercise 7.136

Solution 41

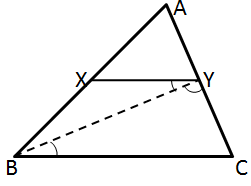
begin mathsize 11px style table attributes columnalign left end attributes row cell Given space XY parallel to BC end cell row cell Hence space angle XYB space equals space angle YBC end cell row cell By space alterate space Interior space angle space property end cell row cell In straight space ΔYBC end cell row cell angle space BYC space equals space angle YBC end cell row cell And space sides space opposite space to space equal space angles space are space also space equal. end cell row cell so space box enclose CY equals BC end enclose end cell end table So comma space the space correct space option space is space left parenthesis straight a right parenthesis. end style

Solution 42

begin mathsize 11px style table attributes columnalign left end attributes row cell In straight space ΔABC end cell row cell space space space AB to the power of straight 2 plus AC squared equals BC squared end cell row cell rightwards double arrow space CB to the power of straight 2 equals space 5 to the power of straight 2 plus 12 squared end cell row cell rightwards double arrow space CB to the power of straight 2 equals space 169 end cell row cell rightwards double arrow space BC equals 13 end cell row cell Let space BD equals straight x space space space space space space then space DC space equals space BC minus BD end cell row cell space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space equals space 13 minus straight x end cell row cell In space ΔBDA end cell row cell BD to the power of straight 2 plus AD squared equals AB squared end cell row cell rightwards double arrow space straight x to the power of straight 2 plus AD squared equals 5 squared end cell row cell rightwards double arrow straight space AD squared equals 25 minus straight x squared space space space space space space space space minus negative negative box enclose 1 end cell row cell In space ΔCDA straight space end cell row cell space space space space CD to the power of straight 2 plus AD squared equals AC squared end cell row cell rightwards double arrow straight space left parenthesis 13 minus straight x right parenthesis squared plus AD squared equals 12 squared end cell row cell rightwards double arrow space AD to the power of straight 2 equals space 12 to the power of straight 2 minus straight space left parenthesis 13 minus straight x right parenthesis squared space space space space minus negative negative negative box enclose 2 end cell row cell From space space space box enclose 1 space space & space space box enclose 2 end cell row cell rightwards double arrow space 25 minus straight x to the power of straight 2 space equals space 12 to the power of straight 2 minus left parenthesis 13 minus straight x right parenthesis squared end cell row cell rightwards double arrow space 25 minus straight x to the power of straight 2 equals 144 minus left parenthesis 169 plus straight x squared minus 26 straight x right parenthesis end cell row cell rightwards double arrow space 25 minus straight x to the power of straight 2 equals 144 minus 169 minus straight x squared plus 26 straight x end cell row cell rightwards double arrow space 26 straight x space equals space 25 straight x 2 end cell row cell rightwards double arrow space straight x equals fraction numerator 25 straight space straight x straight space 2 over denominator 26 end fraction equals 25 over 13 end cell row cell Hence space AD to the power of straight 2 equals 25 minus straight x squared end cell row cell space space space space space space space space space space space space space space space space equals space 25 left parenthesis 1 minus 25 over 169 right parenthesis end cell row cell space space space space space space space space space space space space space space space space equals space 25 left parenthesis 144 over 169 right parenthesis end cell row cell space space space space space space space space AD space space space space equals space fraction numerator 5 cross times 12 over denominator 13 end fraction equals 60 over 13 cm end cell end table So comma space the space correct space option space is space left parenthesis straight b right parenthesis. end style

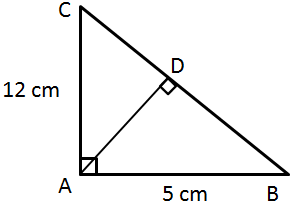
Solution 43

begin mathsize 11px style table attributes columnalign left end attributes row cell space space space space AC squared equals AD squared plus DC squared end cell row cell space space space space AC squared equals 4 squared plus 2 squared end cell row cell space space space space AC equals square root of 20 equals 2 square root of 5 end cell row cell space space space space BD squared plus AD squared equals AB squared end cell row cell rightwards double arrow straight space AB squared equals 8 squared plus 4 squared end cell row cell space space space space space space space space space space space equals space 80 end cell row cell rightwards double arrow thin space space AB space equals space square root of 80 equals 4 square root of 5 end cell row cell space space space space space BC space equals space 10 end cell row cell From space sides space of space triangle end cell row cell space space space space space AC to the power of straight 2 plus AB squared equals BC squared end cell row cell Hence straight space ΔABC straight space is space right space angled space at space straight A. end cell end table So comma space the space correct space option space is space left parenthesis straight d right parenthesis. end style

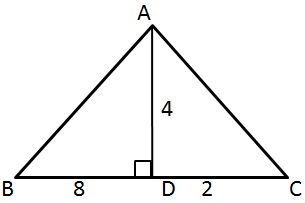
Solution 44

begin mathsize 11px style table attributes columnalign left end attributes row cell Given space DECB space is space trapezium comma space end cell row cell so space DE parallel to BC end cell row cell Hence space ΔADE space is space similar space to space ΔABC end cell row cell Given space DE over BC equals 3 over 5 end cell row cell Hence straight space fraction numerator area straight space left parenthesis ΔADE right parenthesis over denominator area straight space left parenthesis ΔABC right parenthesis end fraction equals left parenthesis 3 over 5 right parenthesis squared equals 9 over 25 end cell row cell rightwards double arrow straight space fraction numerator area straight space left parenthesis ΔABC right parenthesis over denominator area straight space left parenthesis ΔADE right parenthesis end fraction equals 25 over 9 end cell row cell rightwards double arrow fraction numerator area straight space left parenthesis ΔABC right parenthesis over denominator area straight space left parenthesis ΔADE right parenthesis end fraction minus 1 equals 25 over 9 minus 1 end cell row cell rightwards double arrow fraction numerator area straight space left parenthesis ΔABC right parenthesis minus area straight space left parenthesis ΔADE right parenthesis over denominator area straight space left parenthesis ΔADE right parenthesis end fraction equals 16 over 9 end cell row cell rightwards double arrow fraction numerator area straight space left parenthesis square BCED right parenthesis over denominator area straight space left parenthesis ΔADE right parenthesis end fraction equals 16 over 9 end cell row cell rightwards double arrow fraction numerator area straight space left parenthesis ΔADE right parenthesis over denominator area straight space left parenthesis square BCED right parenthesis end fraction equals 9 over 16 end cell end table So comma space the space correct space option space is space left parenthesis straight b right parenthesis. end style

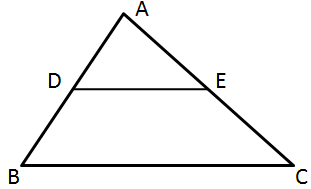
Solution 45

begin mathsize 11px style table attributes columnalign left end attributes row cell AB squared equals AD squared plus BD squared space space space space space space space space minus negative negative negative box enclose 1 end cell row cell AC squared equals AD squared plus DC squared space space space space space space space space minus negative negative negative box enclose 2 end cell row cell Given straight space ΔABC straight space is straight space an straight space isosceles space triangle space end cell row cell Hence space AB equals AC end cell row cell So space from space box enclose 1 space space & space space box enclose 2 end cell row cell BD equals DC end cell row cell rightwards double arrow straight space AB squared minus AD squared equals BD squared end cell row cell rightwards double arrow straight space AB squared minus AD squared equals BD straight space. straight space BD end cell row cell rightwards double arrow straight space AB squared minus AD squared equals BD straight space. space DC end cell end table So comma space the space correct space option space is space left parenthesis straight a right parenthesis. end style

Solution 46

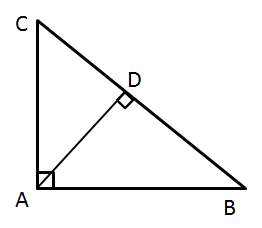
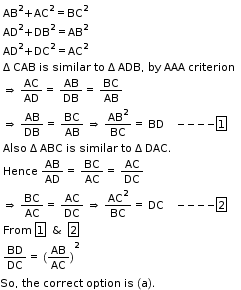
begin mathsize 11px style table attributes columnalign left end attributes row cell AB squared plus AC squared equals BC squared end cell row cell AD squared plus DB squared equals AB squared end cell row cell AD squared plus DC squared equals AC squared end cell row cell straight capital delta space CAB space is space similar space to space straight capital delta space ADB comma space by space AAA space criterion space end cell row cell rightwards double arrow straight space AC over AD equals straight space AB over DB equals straight space BC over AB end cell row cell rightwards double arrow straight space AB over DB equals straight space BC over AB straight space rightwards double arrow straight space AB squared over BC equals space BD space space space space minus negative negative negative box enclose 1 end cell row cell Also straight space straight capital delta space ABC space is space similar space to space straight capital delta space DAC. end cell row cell Hence space AB over AD equals straight space BC over AC equals straight space AC over DC end cell row cell rightwards double arrow straight space BC over AC equals straight space AC over DC straight space rightwards double arrow straight space AC squared over BC equals space DC space space space space minus negative negative negative box enclose 2 end cell row cell From straight space box enclose 1 space space & space space box enclose 2 end cell row cell BD over DC equals straight space left parenthesis AB over AC right parenthesis squared end cell end table So comma space the space correct space option space is space left parenthesis straight a right parenthesis. end style

Solution 47

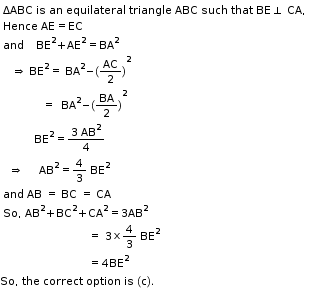
begin mathsize 11px style table attributes columnalign left end attributes row cell ΔABC space is space an space equilateral space triangle space ABC space such space that space BE perpendicular space CA comma end cell row cell Hence space AE equals EC space end cell row cell and space space space space BE to the power of straight 2 plus AE to the power of straight 2 equals BA to the power of straight 2 straight space end cell row cell     rightwards double arrow space BE to the power of straight 2 =  BA to the power of straight 2 minus left parenthesis AC over 2 right parenthesis squared end cell row cell              =   BA to the power of straight 2 minus left parenthesis BA over 2 right parenthesis squared end cell row cell           BE to the power of straight 2 equals fraction numerator 3 space AB to the power of straight 2 over denominator 4 end fraction end cell row cell    rightwards double arrow      AB to the power of straight 2 equals straight 4 over 3 space BE to the power of straight 2 straight space end cell row cell and space AB space equals space BC space equals space CA end cell row cell So comma space AB to the power of straight 2 plus BC to the power of straight 2 plus CA to the power of straight 2 equals 3 AB squared end cell row cell                              =   3 cross times 4 over 3 straight space BE squared end cell row cell                              =  4 BE squared end cell end table So comma space the space correct space option space is space left parenthesis straight c right parenthesis. end style

Solution 48

begin mathsize 11px style table attributes columnalign left end attributes row cell AB squared plus BC squared equals AC squared space space space space space minus negative negative negative box enclose 1 end cell row cell BQ squared plus BA squared equals AQ squared space space space space space minus negative negative negative box enclose 2 end cell row cell BC squared plus BP squared equals PC squared space space space space space minus negative negative negative box enclose 3 end cell row cell BP squared plus BQ squared equals PQ squared space space space space space minus negative negative negative box enclose 4 end cell row cell on space adding space box enclose 2 space & space box enclose 3 end cell row cell AQ squared plus PC squared equals BQ squared plus BA squared plus BC squared plus BP squared end cell row cell thin space space space space space space space space space space space space space equals space BQ squared plus BP squared plus BA squared plus BC squared end cell row cell space space space space space space space space space space space space space space equals space PQ squared plus AC squared end cell end table So comma space the space correct space option space is space left parenthesis straight c right parenthesis. end style

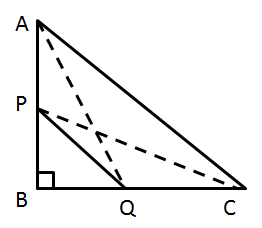
Solution 49

begin mathsize 11px style table attributes columnalign left end attributes row cell      AB over DE equals BC over EF equals CA over FD end cell row cell rightwards double arrow straight space AB over 3 equals 4 over 2 equals fraction numerator CA over denominator 2.5 end fraction end cell row cell rightwards double arrow straight space AB over 3 equals 4 over 2 space space space and space space space rightwards double arrow straight space 4 over 2 equals fraction numerator CA over denominator 2.5 end fraction end cell row cell rightwards double arrow space AB space equals space 6 space space space space and space space AC space equals space 5 end cell row cell perimeter space equals space AB space plus space BC space plus space CA end cell row               =  6  +  4  +  5 row cell space space space space space space space space space space space space space space equals space 15 space cm end cell end table So comma space the space correct space option space is space left parenthesis straight d right parenthesis. end style

Solution 50

begin mathsize 11px style table attributes columnalign left end attributes row cell space space space space space AB over DE equals fraction numerator perimeter space of space ΔABC over denominator perimeter space of space ΔDEF end fraction end cell row cell rightwards double arrow straight space fraction numerator 9.1 over denominator 6.5 end fraction equals fraction numerator perimeter space of space ΔABC over denominator 25 end fraction end cell row cell rightwards double arrow straight space perimeter space of space ΔABC equals fraction numerator 9.1 over denominator 6.5 end fraction space straight x space 25 end cell row cell space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space space equals space 35 space cm end cell end table So comma space the space correct space option space is space left parenthesis straight d right parenthesis. end style

Solution 51

begin mathsize 11px style table attributes columnalign left end attributes row cell Let space BD space equals space straight x space space then space DC space equals space 14 minus straight x end cell row cell Also space space space AD to the power of straight 2 space plus space BD to the power of straight 2 equals AB squared end cell row cell rightwards double arrow space space space space AD to the power of straight 2 plus straight x squared equals 25 squared minus negative negative negative box enclose 1 end cell row cell And space space AD to the power of straight 2 plus DC squared equals AC squared end cell row cell rightwards double arrow space space space space AD to the power of straight 2 plus left parenthesis 14 minus straight x right parenthesis squared equals 25 squared minus negative negative negative box enclose 2 end cell row cell from space space box enclose 1 space space & space box enclose 2 end cell row cell rightwards double arrow space space straight x to the power of straight 2 space space equals space left parenthesis 14 minus straight x right parenthesis squared end cell row cell rightwards double arrow space space space straight x space equals space 14 space minus space straight x end cell row cell rightwards double arrow space space 2 straight x space equals 14 end cell row cell rightwards double arrow space straight x equals 7 end cell row cell Hence space AD to the power of straight 2 plus 7 squared equals 25 squared end cell row cell AD squared equals 625 minus 49 equals 576 end cell row cell AD equals 24 cm end cell end table So comma space the space correct space option space is space left parenthesis straight d right parenthesis. end style